| Revision as of 10:33, 15 July 2013 view sourceChjoaygame (talk | contribs)Extended confirmed users13,141 edits New section: Macroscopic view of heat transfer← Previous edit | Revision as of 18:22, 15 July 2013 view source Damorbel (talk | contribs)Extended confirmed users1,925 edits Undid revision 564342796 by Chjoaygame (talk)Undone! Refs. @$300.00 absurdly inaccessible. Please find something better!Next edit → | ||
| Line 71: | Line 71: | ||
| ] involves three or more systems, which may be closed or open. A process of convection takes some finite amount of time, because it involves three steps at least. The simplest kind of convection has a hot reservoir, a cold reservoir, and a carrier body. In this simplest kind of convection, the carrier body exchanges heat successively with the respective thermal reservoirs. The second law of thermodynamics requires the carrier body to be initially colder than the hot reservoir and finally warmer than the cold reservoir. For convection in general, the transfers of energy can be of more general kinds. For example, for convection between open systems, the transfers may be more conveniently described in terms of internal energy, or of ], or of some other quantity of energy. Here a convenient model is described by internal energy. First, the carrier body increases its internal energy by taking internal energy from the source reservoir. Then it moves through space and carries its internal energy from the location of the source reservoir to that of the destination reservoir; this step is characteristic of convection, and is sometimes called advection. Then it decreases its internal energy by giving energy to the destination reservoir. Convection can transfer internal energy as latent heat, and can be from a source at a lower temperature to a destination at a higher one, work being provided to drive the transfer. | ] involves three or more systems, which may be closed or open. A process of convection takes some finite amount of time, because it involves three steps at least. The simplest kind of convection has a hot reservoir, a cold reservoir, and a carrier body. In this simplest kind of convection, the carrier body exchanges heat successively with the respective thermal reservoirs. The second law of thermodynamics requires the carrier body to be initially colder than the hot reservoir and finally warmer than the cold reservoir. For convection in general, the transfers of energy can be of more general kinds. For example, for convection between open systems, the transfers may be more conveniently described in terms of internal energy, or of ], or of some other quantity of energy. Here a convenient model is described by internal energy. First, the carrier body increases its internal energy by taking internal energy from the source reservoir. Then it moves through space and carries its internal energy from the location of the source reservoir to that of the destination reservoir; this step is characteristic of convection, and is sometimes called advection. Then it decreases its internal energy by giving energy to the destination reservoir. Convection can transfer internal energy as latent heat, and can be from a source at a lower temperature to a destination at a higher one, work being provided to drive the transfer. | ||
| ==Macroscopic view of quantity of energy transferred as heat== | |||
| According to Bailyn, there are two main conceptual approaches to macroscopic quantity of energy transferred as heat, the thermodynamic view, and the mechanical view.<ref>Bailyn, M. (1994), pp. 65, 79.</ref> The thermodynamic view was taken by the founders of thermodynamics in the nineteenth century. It regards quantity of energy transferred as heat as a primitive concept coherent with a primitive concept of temperature, measured primarily by calorimetry. The mechanical view was developed and used in the twentieth century, largely through the influence of ].<ref>](1949), Lecture ] (1949), p. 44.</ref> It follows that the mechanical view does not provide a definition of quantity of energy transferred as heat between open systems. | |||
| Nevertheless, for the thermodynamical description of non-equilibrium processes, it is desired to consider the effect of a temperature gradient established by the surroundings across the system of interest when there is no physical barrier or wall between system and surroundings, that is to say, when they are open with respect to one another. The impossibility of a mechanical definition in terms of work for this circumstance does not alter the physical fact that a temperature gradient causes a diffusive flux of internal energy, a process that, in the thermodynamic view, is regarded as primitively presupposed as transfer of energy as heat. | |||
| In this circumstance, it may expected that there may also be active other drivers of diffusive flux of internal energy, such as gradient of chemical potential which drives transfer of matter, and gradient of electric potential which drives electric current and iontophoresis; such effects usually interact with diffusive flux of internal energy driven by temperature gradient, and such interactions are known as cross-effects.<ref>De Groot, S.R., Mazur, P. (1962), p. 30.</ref> | |||
| If cross-effects that result in diffusive transfer of internal energy were also labeled as heat transfers, they would seem to be able to violate the rule that pure heat transfer occurs only down a temperature gradient, never up one, and to contradict the principle that all heat transfer is of one and the same kind, a principle founded on the idea of heat conduction between closed systems. Therefore it is reasonable to think narrowly of heat flux driven purely by temperature gradient as a conceptual component of diffusive internal energy flux, in the thermodynamic view, the concept resting specifically on careful calculations based on detailed knowledge of the processes and being indirectly assessed. In these circumstances, if perchance it happens that no transfer of matter is actualized, and there are no cross-effects, then the thermodynamic concept and the mechanical concept coincide, as if one were dealing with closed systems. | |||
| In many writings in this context, the term "heat flux" is used when what is meant is therefore more accurately called diffusive flux of internal energy; such usage of the term "heat flux" is a residue of older and now obsolete language usage that allowed that a body may have a "heat content".<ref>Gyarmati, I. (1970), p. 68.</ref> | |||
| ==Notation and units== | ==Notation and units== | ||
| Line 269: | Line 257: | ||
| ==References== | ==References== | ||
| {{reflist |
{{reflist}} | ||
| === Bibliography === | === Bibliography === | ||
| Line 276: | Line 264: | ||
| *Bailyn, M. (1994). ''A Survey of Thermodynamics'', American Institute of Physics Press, New York, ISBN 0-88318-797-3. | *Bailyn, M. (1994). ''A Survey of Thermodynamics'', American Institute of Physics Press, New York, ISBN 0-88318-797-3. | ||
| *Beattie, J.A., Oppenheim, I. (1979). ''Principles of Thermodynamics'', Elsevier, Amsterdam, ISBN 0–444–41806–7. | *Beattie, J.A., Oppenheim, I. (1979). ''Principles of Thermodynamics'', Elsevier, Amsterdam, ISBN 0–444–41806–7. | ||
| ⚫ | *Bryan, G.H. (1907). ''Thermodynamics. An Introductory Treatise dealing mainly with First Principles and their Direct Applications'', B.G. Teubner, Leipzig. | ||
| *] (1949). ''Natural Philosophy of Cause and Chance'', Oxford University Press, London. | |||
| ⚫ | * |
||
| *Buchdahl, H.A. (1966), ''The Concepts of Classical Thermodynamics'', Cambridge University Press, London. | *Buchdahl, H.A. (1966), ''The Concepts of Classical Thermodynamics'', Cambridge University Press, London. | ||
| *] (1960/1985). ''Thermodynamics and an Introduction to Thermostatistics'', (1st edition 1960) 2nd edition 1985, Wiley, New York, ISBN 0-471-86256-8. | *] (1960/1985). ''Thermodynamics and an Introduction to Thermostatistics'', (1st edition 1960) 2nd edition 1985, Wiley, New York, ISBN 0-471-86256-8. | ||
| *de Groot, S.R., Mazur, P. (1962). ''Non-equilibrium Thermodynamics'', North-Holland, Amsterdam. Reprinted (1984), Dover Publications Inc., New York, ISBN 0486647412. | |||
| *Fowler, R., Guggenheim, E.A. (1939/1965). ''Statistical Thermodynamics. A version of Statistical Mechanics for Students of Physics and Chemistry'', Cambridge University Press, Cambridge UK. | *Fowler, R., Guggenheim, E.A. (1939/1965). ''Statistical Thermodynamics. A version of Statistical Mechanics for Students of Physics and Chemistry'', Cambridge University Press, Cambridge UK. | ||
| *{{Citation | *{{Citation | ||
| Line 307: | Line 293: | ||
| | isbn = | | isbn = | ||
| }} | }} | ||
| *Gyarmati, I. (1967/1970). ''Non-equilibrium Thermodynamics. Field Theory and Variational Principles'', translated from the 1967 Hungarian by E. Gyarmati and W.F. Heinz, Springer-Verlag, New York. | |||
| *Haase, R. (1971). Survey of Fundamental Laws, chapter 1 of ''Thermodynamics'', pages 1–97 of volume 1, ed. W. Jost, of ''Physical Chemistry. An Advanced Treatise'', ed. H. Eyring, D. Henderson, W. Jost, Academic Press, New York, lcn 73–117081. | *Haase, R. (1971). Survey of Fundamental Laws, chapter 1 of ''Thermodynamics'', pages 1–97 of volume 1, ed. W. Jost, of ''Physical Chemistry. An Advanced Treatise'', ed. H. Eyring, D. Henderson, W. Jost, Academic Press, New York, lcn 73–117081. | ||
| *{{Citation | *{{Citation | ||
Revision as of 18:22, 15 July 2013
For other uses, see Heat (disambiguation).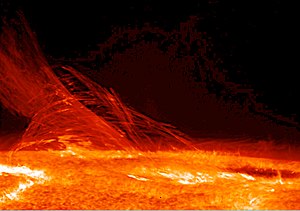
In physics and chemistry, heat is energy transferred from one body to another by thermal interactions. The transfer of energy can occur in a variety of ways, among them conduction, radiation, and convection. Heat is not a property of a system or body, but instead is always associated with a process of some kind, and is synonymous with heat flow and heat transfer.
Heat flow from hotter to colder systems occurs spontaneously, and is always accompanied by an increase in entropy. In a heat engine, internal energy of bodies is harnessed to provide useful work. The second law of thermodynamics states the principle that heat cannot flow directly from cold to hot systems, but with the aid of a heat pump external work can be used to transport internal energy indirectly from a cold to a hot body.
Transfers of energy as heat are macroscopic processes. The origin and properties of heat can be understood through the statistical mechanics of microscopic constituents such as molecules and photons. For instance, heat flow can occur when the rapidly vibrating molecules in a high temperature body transfer some of their energy (by direct contact, radiation exchange, or other mechanisms) to the more slowly vibrating molecules in a lower temperature body.
The SI unit of heat is the joule. Heat can be measured by calorimetry, or determined indirectly by calculations based on other quantities, relying for instance on the first law of thermodynamics. In calorimetry, the concepts of latent heat and of sensible heat are used. Latent heat produces changes of state without temperature change, while sensible heat produces temperature change.
Overview

Heat in physics is defined as energy transferred by thermal interactions. Heat flows spontaneously from hotter to colder systems. When two closed systems come into thermal contact, they exchange energy through the microscopic interactions of their particles. When the systems are at different temperatures, the result is a spontaneous net flow of energy that continues until the temperatures are equal. At that point the net flow of energy is zero, and the systems are said to be in thermal equilibrium. Spontaneous heat transfer is an irreversible process.
According to the first law of thermodynamics, the internal energy of an isolated system is conserved. To change the internal energy of a system, energy must be transferred to or from the system. For a closed system, heat and work are the mechanisms by which energy can be transferred. For an open system, internal energy can be changed also by transfer of matter. Work performed by a closed system is, by definition, an energy transfer from the system that is due to a change to its external or mechanical parameters, such as the volume, magnetization, and location of center of mass in a gravitational field.
When energy is transferred to a body purely as heat, its internal energy increases. This additional energy is stored as kinetic and potential energy of the atoms and molecules in the body. Heat itself is not stored within a body. Like work, it exists only as energy in transit from one body to another or between a body and its surroundings.
Between closed systems, a given suitable heat conductor is indifferent to the source and destination of the heat it conducts, and a given heat transfer between two bodies is indifferent to the choice of a suitable heat conductor. Consequently all purely conducted heat is of the same kind.
Microscopic view of heat
Heat characterizes macroscopic systems and processes, but like other thermodynamic quantities it has a fundamental origin in the energy of particles, atoms, molecules etc. and their underlying microscopic degrees of freedom.
For example,, the temperature of a gas is proportional (via Boltzmann's constant kB) to the average kinetic energy of its molecules. Heat transfer between a low and high temperature gas brought into contact arises due to the exchange of kinetic and potential energy in molecular collisions. As more and more molecules undergo collisions, their kinetic energy equilibrates to a distribution that corresponds to an intermediate temperature somewhere between the low and high initial temperatures of the two gases. An early and vague expression of this was by Francis Bacon. Precise and detailed versions of it were developed in the nineteenth century.
For solids, conduction of heat occurs through collective motions of microscopic particles, such as phonons, or through the motion of mobile particles like conduction band electrons. As these excitations move around inside the solid and interact with it and each other, they transfer energy from higher to lower temperature regions, eventually leading to thermal equilibrium.
History
Scottish physicist James Clerk Maxwell, in his 1871 classic Theory of Heat, was one of many who began to build on the already established idea that heat has something to do with matter in motion. This was the same idea put forth by Sir Benjamin Thompson in 1798, who said he was only following up on the work of many others. One of Maxwell's recommended books was Heat as a Mode of Motion, by John Tyndall. Maxwell outlined four stipulations for the definition of heat:
- It is something which may be transferred from one body to another, according to the second law of thermodynamics.
- It is a measurable quantity, and thus treated mathematically.
- It cannot be treated as a substance, because it may be transformed into something that is not a substance, e.g., mechanical work.
- Heat is one of the forms of energy.
From empirically based ideas of heat, and from other empirical observations, the notions of internal energy and of entropy can be derived, so as to lead to the recognition of the first and second laws of thermodynamics. This was the way of the historical pioneers of thermodynamics.
Transfers of energy between closed systems
Adiabatic transfer of energy as work between two bodies
A body can be connected to its surroundings by links that allow transfer of energy only as work, not as heat, because the body is adiabatically isolated. Such transfer can be of two pure kinds, volume work, and isochoric work. Volume work means that the initial volume and the final volume of the body are different, and that mechanical work is transferred through the forces that cause the changes in the deformation parameters. Isochoric work is done on the body by the surroundings when the initial and final volumes and all deformation parameters of the body are unchanged. For example, the surroundings can do work through a changing magnetic field that rotates a magnetic stirrer within the body. Another example is 'shaft work', in which an externally driven shaft rotates fan- or paddle-blades within the body. Another example is rubbing, considered as tangential motion of a wall that contains the body. Stirring and rubbing were the main forms of work in Joule's experiments.
Transfers of energy as heat between two bodies
Referring to conduction, Partington writes: "If a hot body is brought in conducting contact with a cold body, the temperature of the hot body falls and that of the cold body rises, and it is said that a quantity of heat has passed from the hot body to the cold body."
Referring to radiation, Maxwell writes: "In Radiation, the hotter body loses heat, and the colder body receives heat by means of a process occurring in some intervening medium which does not itself thereby become hot."
Diathermal wall
In considering transfer of energy between two bodies, it is customary to allow the existence of a wall between them which is permeable only to heat. This allowance is a presupposition of thermodynamics. Such a wall is called diathermal. It is usual for many theoretical discussions to allow that the wall itself has negligibly small internal energy. Then the only property of interest in the wall is that it allows conduction and radiation of heat between the two bodies of interest. While walls are readily found which are permeable only to heat, and impermeable to matter, it is very exceptional indeed to find walls which are permeable to matter but not to entropy. Such a rare exception is a wall penetrated by fine capillaries, which allow the passage of the superfluid of helium II but not of the normal fluid.
It is sometimes allowed that the diathermal wall is substantial and has properties of its own including a temperature of its own, and it is considered that the wall is a body in its own right, but is still considered as a closed system not permitted to exchange matter. Then when there is thermal equilibrium between the two bodies of interest, there is also thermal equilibrium between each of them and the wall, and all three have the same temperature. Then from the viewpoint of states of thermal equilibrium, all diathermal walls are equivalent; this is proposed as a possible statement of the zeroth law of thermodynamics. If heat is considered with respect to diathermal walls, then, because all diathermal walls are equivalent, all heat is of the same kind.
Convective circulation
When two bodies are initially separate and not connected by a substantial wall, and at different temperatures, and are then connected with a substantial diathermal wall as connecting medium, the properties and state of the wall need to be taken into account for the process of transfer of energy as heat. If the wall contains fluid, and there is a gravitational field, then transfer of energy as heat between the two bodies of interest may involve convective circulation within the wall, with no transfer of matter into or out of the wall. In this sense, it can be said that convective circulation is a mechanism of transfer of energy as heat, but in this case, the transfer of energy as heat is complex, because of change of internal energy and temperature of the wall. Thermal convective circulation is always opposed by friction, and consequently occurs only above a threshold of thermal difference between source and destination of the transferred energy. Thermal equilibrium between source and destination is therefore finally reached by a non-convective stage, of conduction and radiation. In discussions of thermodynamics, such a convective circulatory diathermal wall and process of transfer of energy as heat is not usually intended unless they are explicitly expressed.
Transfers of energy involving more than two bodies
Heat engine
In classical thermodynamics, a commonly considered model is the heat engine. It consists of four bodies: the working body, the hot reservoir, the cold reservoir, and the work reservoir. A cyclic process leaves the working body in an unchanged state, and is envisaged as being repeated indefinitely often. Work transfers between the working body and the work reservoir are envisaged as reversible, and thus only one work reservoir is needed. But two thermal reservoirs are needed, because transfer of energy as heat is irreversible. A single cycle sees energy taken by the working body from the hot reservoir and sent to the two other reservoirs, the work reservoir and the cold reservoir. The hot reservoir always and only supplies energy and the cold reservoir always and only receives energy. The second law of thermodynamics requires that no cycle can occur in which no energy is received by the cold reservoir.
Convective transfer of energy
Convective transfer of energy involves three or more systems, which may be closed or open. A process of convection takes some finite amount of time, because it involves three steps at least. The simplest kind of convection has a hot reservoir, a cold reservoir, and a carrier body. In this simplest kind of convection, the carrier body exchanges heat successively with the respective thermal reservoirs. The second law of thermodynamics requires the carrier body to be initially colder than the hot reservoir and finally warmer than the cold reservoir. For convection in general, the transfers of energy can be of more general kinds. For example, for convection between open systems, the transfers may be more conveniently described in terms of internal energy, or of enthalpy, or of some other quantity of energy. Here a convenient model is described by internal energy. First, the carrier body increases its internal energy by taking internal energy from the source reservoir. Then it moves through space and carries its internal energy from the location of the source reservoir to that of the destination reservoir; this step is characteristic of convection, and is sometimes called advection. Then it decreases its internal energy by giving energy to the destination reservoir. Convection can transfer internal energy as latent heat, and can be from a source at a lower temperature to a destination at a higher one, work being provided to drive the transfer.
Notation and units
As a form of energy heat has the unit joule (J) in the International System of Units (SI). However, in many applied fields in engineering the British Thermal Unit (BTU) and the calorie are often used. The standard unit for the rate of heat transferred is the watt (W), defined as joules per second.
The total amount of energy transferred as heat is conventionally written as Q for algebraic purposes. Heat released by a system into its surroundings is by convention a negative quantity (Q < 0); when a system absorbs heat from its surroundings, it is positive (Q > 0). Heat transfer rate, or heat flow per unit time, is denoted by . This should not be confused with a time derivative of a function of state (which can also be written with the dot notation) since heat is not a function of state. Heat flux is defined as rate of heat transfer per unit cross-sectional area, resulting in the unit watts per square metre.
Estimation of quantity of heat
Quantity of heat transferred can measured by calorimetry, or determined through calculations based on other quantities.
Calorimetry is the empirical basis of the idea of quantity of heat transferred in a process. The transferred heat is measured by changes in a body of known properties, for example, temperature rise, change in volume or length, or phase change, such as melting of ice.
A calculation of quantity of heat transferred can rely on a hypothetical quantity of energy transferred as adiabatic work and on the first law of thermodynamics. Such calculation is the primary approach of many theoretical studies of quantity of heat transferred.
Internal energy and enthalpy
For a closed system (a system from which no matter can enter or exit), the first law of thermodynamics states that the change in internal energy ΔU of the system is equal to the amount of heat Q supplied to the system minus the amount of work W done by system on its surroundings.
The expressions 'Q' and 'W' represent amounts of energy but energy in different forms, heat and work. It is fundamental to the science of heat that conversion between heat (Q) and work (W) is never 100% efficient, so the ' = ' sign cannot have its usual mathematical meaning.
The work done by the system includes boundary work (when the system increases its volume against an external force, such as that exerted by a piston) and other work (e.g. shaft work performed by a compressor fan), which is called isochoric work:
In this Section we will neglect the "other-work" contribution.
The internal energy, U, is a state function. In cyclical processes, such as the operation of a heat engine, state functions of the working substance return to their initial values upon completion of a cycle.
The differential, or infinitesimal increment, for the internal energy in an infinitesimal process is an exact differential dU. The symbol for exact differentials is the lowercase letter d.
In contrast, neither of the infintestimal increments δQ nor δW in an infinitesimal process represents the state of the system. Thus, infinitesimal increments of heat and work are inexact differentials. The lowercase Greek letter delta, δ, is the symbol for inexact differentials. The integral of any inexact differential over the time it takes for a system to leave and return to the same thermodynamic state does not necessarily equal zero.
The second law of thermodynamics observes that if heat is supplied to a system in which no irreversible processes take place and which has a well-defined temperature T, the increment of heat δQ and the temperature T form the exact differential
and that S, the entropy of the working body, is a function of state. Likewise, with a well-defined pressure, P, behind the moving boundary, the work differential, δW, and the pressure, P, combine to form the exact differential
with V the volume of the system, which is a state variable. In general, for homogeneous systems,
Associated with this differential equation is that the internal energy may be considered to be a function U (S,V) of its natural variables S and V. The internal energy representation of the fundamental thermodynamic relation is written
If V is constant
and if P is constant
with H the enthalpy defined by
The enthalpy may be considered to be a function H (S,P) of its natural variables S and P. The enthalpy representation of the fundamental thermodynamic relation is written
The internal energy representation and the enthalpy representation are partial Legendre transforms of one another. They contain the same physical information, written in different ways.
Latent and sensible heat

In an 1847 lecture entitled On Matter, Living Force, and Heat, James Prescott Joule characterized the terms latent heat and sensible heat as components of heat each affecting distinct physical phenomena, namely the potential and kinetic energy of particles, respectively. He described latent energy as the energy possessed via a distancing of particles where attraction was over a greater distance, i.e. a form of potential energy, and the sensible heat as an energy involving the motion of particles or what was known as a living force. At the time of Joule kinetic energy either held 'invisibly' internally or held 'visibly' externally was known as a living force.
Latent heat is the heat released or absorbed by a chemical substance or a thermodynamic system during a change of state that occurs without a change in temperature. Such a process may be a phase transition, such as the melting of ice or the boiling of water. The term was introduced around 1750 by Joseph Black as derived from the Latin latere (to lie hidden), characterizing its effect as not being directly measurable with a thermometer.
Sensible heat, in contrast to latent heat, is the heat exchanged by a thermodynamic system that has as its sole effect a change of temperature. Sensible heat therefore only increases the thermal energy of a system.
Consequences of Black's distinction between sensible and latent heat are examined in the Misplaced Pages article on calorimetry.
Specific heat
Specific heat, also called specific heat capacity, is defined as the amount of energy that has to be transferred to or from one unit of mass (kilogram) or amount of substance (mole) to change the system temperature by one degree. Specific heat is a physical property, which means that it depends on the substance under consideration and its state as specified by its properties.
The specific heats of monatomic gases (e.g., helium) are nearly constant with temperature. Diatomic gases such as hydrogen display some temperature dependence, and triatomic gases (e.g., carbon dioxide) still more.
Rigorous mathematical definition of quantity of energy transferred as heat
It is sometimes convenient to have a very rigorous mathematically stated definition of quantity of energy transferred as heat. Such definition is customarily based on the work of Carathéodory (1909), referring to processes in a closed system, as follows.
The internal energy UX of a body in an arbitrary state X can be determined by amounts of work adiabatically performed by the body on its surrounds when it starts from a reference state O, allowing that sometimes the amount of work is calculated by assuming that some adiabatic process is virtually though not actually reversible. Adiabatic work is defined in terms of adiabatic walls, which allow the frictionless performance of work but no other transfer, of energy or matter. In particular they do not allow the passage of energy as heat. According to Carathéodory (1909), passage of energy as heat is allowed, by walls which are "permeable only to heat".
For the definition of quantity of energy transferred as heat, it is customarily envisaged that an arbitrary state of interest Y is reached from state O by a process with two components, one adiabatic and the other not adiabatic. For convenience one may say that the adiabatic component was the sum of work done by the body through volume change through movement of the walls while the non-adiabatic partition was excluded, and of isochoric adiabatic work. Then the non-adiabatic component is a process of energy transfer through the wall that passes only heat, newly made accessible for the purpose of this transfer, from the surroundings to the body. The change in internal energy to reach the state Y from the state O is the difference of the two amounts of energy transferred.
Although Carathéodory himself did not state such a definition, following his work it is customary in theoretical studies to define the quantity of energy transferred as heat, Q, to the body from its surroundings, in the combined process of change to state Y from the state O, as the change in internal energy, ΔUY, minus the amount of work, W, done by the body on its surrounds by the adiabatic process, so that Q = ΔUY − W.
In this definition, for the sake of mathematical rigour, the quantity of energy transferred as heat is not specified directly in terms of the non-adiabatic process. It is defined through knowledge of precisely two variables, the change of internal energy and the amount of adiabatic work done, for the combined process of change from the reference state O to the arbitrary state Y. It is important that this does not explicitly involve the amount of energy transferred in the non-adiabatic component of the combined process. It is assumed here that the amount of energy required to pass from state O to state Y, the change of internal energy, is known, independently of the combined process, by a determination through a purely adiabatic process, like that for the determination of the internal energy of state X above. The mathematical rigour that is prized in this definition is that there is one and only one kind of energy transfer admitted as fundamental: energy transferred as work. Energy transfer as heat is considered as a derived quantity. The uniqueness of work in this scheme is considered to provide purity of conception, which is considered as guaranteeing mathematical rigour. The conceptual purity of this definition, based on the concept of energy transferred as work as an ideal notion, relies on the idea that some frictionless and otherwise non-dissipative processes of energy transfer can be realized in physical actuality. The second law of thermodynamics, on the other hand, assures us that such processes are not found in nature.
Heat, temperature, and thermal equilibrium regarded as jointly primitive notions
Before the rigorous mathematical definition of heat based on Carathéodory's 1909 paper, recounted just above, historically, heat, temperature, and thermal equilibrium were presented in thermodynamics textbooks as jointly primitive notions. Carathéodory introduced his 1909 paper thus: "The proposition that the discipline of thermodynamics can be justified without recourse to any hypothesis that cannot be verified experimentally must be regarded as one of the most noteworthy results of the research in thermodynamics that was accomplished during the last century." Referring to the "point of view adopted by most authors who were active in the last fifty years", Carathéodory wrote: "There exists a physical quantity called heat that is not identical with the mechanical quantities (mass, force, pressure, etc.) and whose variations can be determined by calorimetric measurements." James Serrin introduces an account of the theory of thermodynamics thus: "In the following section, we shall use the classical notions of heat, work, and hotness as primitive elements, ... That heat is an appropriate and natural primitive for thermodynamics was already accepted by Carnot. Its continued validity as a primitive element of thermodynamical structure is due to the fact that it synthesizes an essential physical concept, as well as to its successful use in recent work to unify different constitutive theories." This traditional kind of presentation of the basis of thermodynamics includes ideas that may be summarized by the statement that heat transfer is purely due to spatial non-uniformity of temperature, and is by conduction and radiation, from hotter to colder bodies. It is sometimes proposed that this traditional kind of presentation necessarily rests on "circular reasoning"; against this proposal, there stands the rigorously logical mathematical development of the theory presented by Truesdell and Bharatha (1977).
This alternative approach to the definition of quantity of energy transferred as heat differs in logical structure from that of Carathéodory, recounted just above.
This alternative approach admits calorimetry as a primary or direct way to measure quantity of energy transferred as heat. It relies on temperature as one of its primitive concepts, and used in calorimetry. It is presupposed that enough processes exist physically to allow measurement of differences in internal energies. Such processes are not restricted to adiabatic transfers of energy as work. They include calorimetry, which is the commonest practical way of finding internal energy differences. The needed temperature can be either empirical or absolute thermodynamic.
In contrast, the Carathéodory way recounted just above does not use calorimetry or temperature in its primary definition of quantity of energy transferred as heat. The Carathédory way regards calorimetry only as a secondary or indirect way of measuring quantity of energy transferred as heat. As recounted in more detail just above, the Carathéodory way regards quantity of energy transferred as heat in a process as primarily or directly defined as a residual quantity. It is calculated from the difference of the internal energies of the initial and final states of the system, and from the actual work done by the system during the process. That internal energy difference is supposed to have been measured in advance through processes of purely adiabatic transfer of energy as work, processes that take the system between the initial and final states. By the Carathéodory way it is presupposed as known from experiment that there actually physically exist enough such adiabatic processes, so that there need be no recourse to calorimetry for measurement of quantity of energy transferred as heat. This presupposition is essential but is explicitly labeled neither as a law of thermodynamics nor as an axiom of the Carathéodory way. In fact, the actual physical existence of such adiabatic processes is indeed mostly supposition, and those supposed processes have in most cases not been actually verified empirically to exist.
Entropy
Main article: Entropy
In 1856, German physicist Rudolf Clausius defined the second fundamental theorem (the second law of thermodynamics) in the mechanical theory of heat (thermodynamics): "if two transformations which, without necessitating any other permanent change, can mutually replace one another, be called equivalent, then the generations of the quantity of heat Q from work at the temperature T, has the equivalence-value:"
In 1865, he came to define the entropy symbolized by S, such that, due to the supply of the amount of heat Q at temperature T the entropy of the system is increased by
and thus, for small changes, quantities of heat δQ (an inexact differential) are defined as quantities of TdS, with dS an exact differential:
This equality is only valid for a closed system and if no irreversible processes take place inside the system while the heat δQ is applied. If, in contrast, irreversible processes are involved, e.g. some sort of friction, then there is entropy production and, instead of the above equation, one has
This is the second law of thermodynamics for closed systems.
Heat transfer in engineering

The discipline of heat transfer, typically considered an aspect of mechanical engineering and chemical engineering, deals with specific applied methods by which thermal energy in a system is generated, or converted, or transferred to another system. Although the definition of heat implicitly means the transfer of energy, the term heat transfer encompasses this traditional usage in many engineering disciplines and laymen language.
Heat transfer includes the mechanisms of heat conduction, thermal radiation, and mass transfer.
In engineering, the term convective heat transfer is used to describe the combined effects of conduction and fluid flow. From the thermodynamic point of view, heat flows into a fluid by diffusion to increase its energy, the fluid then transfers (advects) this increased internal energy (not heat) from one location to another, and this is then followed by a second thermal interaction which transfers heat to a second body or system, again by diffusion. This entire process is often regarded as an additional mechanism of heat transfer, although technically, "heat transfer" and thus heating and cooling occurs only on either end of such a conductive flow, but not as a result of flow. Thus, conduction can be said to "transfer" heat only as a net result of the process, but may not do so at every time within the complicated convective process.
Although distinct physical laws may describe the behavior of each of these methods, real systems often exhibit a complicated combination which are often described by a variety of complex mathematical methods.
Practical applications
In accordance with the first law for closed systems, energy transferred as heat enters one body and leaves another, changing the internal energies of each. Transfer, between bodies, of energy as work is a complementary way of changing internal energies. Though it is not logically rigorous from the viewpoint of strict physical concepts, a common form of words that expresses this is to say that heat and work are interconvertible.
Heat engines operate by converting heat flow from a high temperature reservoir to a low temperature reservoir into work. One example are steam engines, where the high temperature reservoir is steam generated by boiling water. The flow of heat from the hot steam to water is converted into mechanical work via a turbine or piston. Heat engines achieve high efficiency when the difference between initial and final temperature is high.
Heat pumps, by contrast, use work to cause thermal energy to flow from low to high temperature, the opposite direction heat would flow spontaneously. An example is a refrigerator or air conditioner, where electric power is used to cool a low temperature system (the interior of the refrigerator) while heating a higher temperature environment (the exterior). High efficiency is achieved when the temperature difference is small.
Usage of words
The strictly defined physical term 'quantity of energy transferred as heat' has a resonance with the ordinary language noun 'heat' and the ordinary language verb 'heat'. This can lead to confusion if ordinary language is muddled with strictly defined physical language. In the strict terminology of physics, heat is defined as a word that refers to a process, not to a state of a system. In ordinary language one can speak of a process that increases the temperature of a body as 'heating' it, ignoring the nature of the process, which could be one of adiabatic transfer of energy as work. But in strict physical terms, a process is admitted as heating only when what is meant is transfer of energy as heat. Such a process does not necessarily increase the temperature of the heated body, which may instead change its phase, for example by melting. In the strict physical sense, heat cannot be 'produced', because the usage 'production of heat' misleadingly seems to refer to a state variable. Thus, it would be physically improper to speak of 'heat production by friction', or of 'heating by adiabatic compression on descent of an air parcel' or of 'heat production by chemical reaction'; instead, proper physical usage speaks of conversion of kinetic energy of bulk flow, or of potential energy of bulk matter, or of chemical potential energy, into internal energy, and of transfer of energy as heat. Occasionally a present-day author, especially when referring to history, writes of "adiabatic heating", though this is a contradiction in terms of present day physics. Historically, before the concept of internal energy became clear over the period 1850 to 1869, physicists spoke of "heat production" where nowadays one speaks of conversion of other forms of energy into internal energy.
See also
- Effect of sun angle on climate
- Heat death of the Universe
- Heat diffusion
- Heat equation
- Heat exchanger
- Heat flux sensor
- Heat transfer coefficient
- History of heat
- Sigma heat
- Shock heating
- Thermal management of electronic devices and systems
- Thermometer
- Relativistic heat conduction
- Waste heat
Notes
- An alternate sign convention (followed by IUPAC) for the work is to consider the work performed on the system by its surroundings. This is the convention adopted by many modern textbooks of physical chemistry, such as those by Peter Atkins and Ira Levine, but many textbooks on physics define work as work done by the system.
References
- Reif, F. (1965), pp. 67, 73.
- Kittel, C. Kroemer, H. (1980). Thermal Physics, second edition, W.H. Freeman, San Francisco, ISBN 0-7167-1088-9, p. 227.
- Guggenheim, E.A. (1949/1967), p. 8 harvnb error: no target: CITEREFGuggenheim,_E.A._(1949/1967) (help).
- Planck. M. (1914). The Theory of Heat Radiation, a translation by Masius, M. of the second German edition, P. Blakiston's Son & Co., Philadelphia.
- Maxwell 1871, Chapter III.
- Kondepudi, D. (2008), p. 59. harvnb error: no target: CITEREFKondepudi,_D._(2008) (help)
- Planck, M. (1927), p. 4.
- Crawford, F.H. (1963). Heat, Thermodynamics, and Statistical Physics, Rupert Hart-Davis, Harcourt, Brace & World, p. 98.
- Reif, F. (1965), p. 73.
- Gislason, E.A., Craig, N.C. (2005). Cementing the foundations of thermodynamics: Comparison of system-based and surroundings-based definitions of heat and work, J. Chem. Thermodynamics, 37: 954–966.
- Anacleto, J., Ferreira, J.M. (2008). Surroundings-based and system-based heat and work definitions: Which one is most suitable?, J. Chem. Thermodynamics, 40: 134–135.
- Smith, J.M., Van Ness, H.C., Abbot, M.M. (2005). Introduction to Chemical Engineering Thermodynamics. McGraw-Hill. ISBN 0073104450.
{{cite book}}: CS1 maint: multiple names: authors list (link) - ^ Maxwell, J.C. (1871), p. 57.
- Fowler, R., Guggenheim, E.A. (1939/1965). Statistical Thermodynamics. A version of Statistical Mechanics for Students of Physics and Chemistry, Cambridge University Press, Cambridge UK, pp. 70, 255.
- Bacon, F. (1620). Novum Organum Scientiarum, translated by Devey, J., P.F. Collier & Son, New York, 1902.
- Partington, J.R. (1949), page 131.
- Partington, J.R. (1949), pages 132–136.
- Kittel, C. (1953/1980). Introduction to Solid State Physics, (first edition 1953), fifth edition 1980, John Wiley & Sons, New York, ISBN 0-471-49024-5, Chapters 4, 5.
- Planck, M. (1903).
- Partington, J.R. (1949).
- Truesdell, C. (1980), page 15.
- Planck, M. (1923/1927), p. 8.
- Buchdahl H.A. (1966), pp. 6–7.
- Wilson, A.H. (1957), p. 7.
- Reif, F. (1965), p. 72.
- Münster, A. (1970), p. 45.
- Partington, J.R. (1949), p. 118.
- Maxwell, J.C. (1871), p. 10.
- ^ C. Carathéodory (1909). "Untersuchungen über die Grundlagen der Thermodynamik". Mathematische Annalen. 67: 355–386. doi:10.1007/BF01450409. A mostly reliable translation is to be found at Kestin, J. (1976). The Second Law of Thermodynamics, Dowden, Hutchinson & Ross, Stroudsburg PA.
- Tisza, L. (1966), pp. 139–140.
- Bailyn, M. (1994), pp. 22–23.
- Chandrasekhar, S. (1961). Hydrodynamic and Hydromagnetic Stability, Oxford University Press, Oxford UK.
- Maxwell J.C. (1872), p. 54.
- Planck (1927), Chapter 3.
- Bryan, G.H. (1907), p. 47.
- Callen, H.B. (1985), Section 1-8.
- Callen, H.B., (1985), Section 2-3, pp. 40–42.
- ^ Adkins, C.J. (1983), p. 101.
- ^ Callen, H.B. (1985), p. 147.
- Adkins, C.J. (1983), pp. 100–104.
- J. P. Joule (1884), The Scientific Paper of James Prescott Joule, The Physical Society of London, p. 274,
Heat must therefore consist of either living force or of attraction through space. In the former case we can conceive the constituent particles of heated bodies to be, either in whole or in part, in a state of motion. In the latter we may suppose the particles to be removed by the process of heating, so as to exert attraction through greater space. I am inclined to believe that both of these hypotheses will be found to hold good,—that in some instances, particularly in the case of sensible heat, or such as is indicated by the thermometer, heat will be found to consist in the living force of the particles of the bodies in which it is induced; whilst in others, particularly in the case of latent heat, the phenomena are produced by the separation of particle from particle, so as to cause them to attract one another through a greater space.
, Lecture on Matter, Living Force, and Heat. May 5 and 12, 1847 - Perrot, Pierre (1998). A to Z of Thermodynamics. Oxford University Press. ISBN 0-19-856552-6.
- Clark, John, O.E. (2004). The Essential Dictionary of Science. Barnes & Noble Books. ISBN 0-7607-4616-8.
{{cite book}}: CS1 maint: multiple names: authors list (link) - Ritter, Michael E. (2006). "The Physical Environment: an Introduction to Physical Geography".
- Adkins, C.J. (1968/1983).
- Münster, A. (1970).
- Pippard, A.B. (1957).
- Fowler, R., Guggenheim, E.A. (1939).
- Buchdahl, H.A. (1966).
- Lieb, E.H., Yngvason, J. (1999). The physics and mathematics of the second law of thermodynamics, Physics Reports, 314: 1–96, p. 10.
- Serrin, J. (1986). Chapter 1, 'An Outline of Thermodynamical Structure', pages 3-32, in New Perspectives in Thermodynamics, edited by J. Serrin, Springer, Berlin, ISBN 3-540-15931-2, p. 5 .
- Owen, D.R. (1984), pp. 43–45.
- Truesdell, C., Bharatha, S. (1977). The Concepts and Logic of Classical Thermodynamics as a Theory of Heat Engines, Rigorously Constructed upon the Foundation Laid by S. Carnot and F. Reech, Springer, New York, ISBN 0-387-07971-8.
- Maxwell, J.C. (1871), p. v.
- Atkins, P., de Paula, J. (1978/2010). Physical Chemistry, (first edition 1978), ninth edition 2010, Oxford University Press, Oxford UK, ISBN 978-0-19-954337-3, p. 54.
- Pippard, A.B. (1957/1966), p. 15.
- Published in Poggendoff’s Annalen, Dec. 1854, vol. xciii. p. 481; translated in the Journal de Mathematiques, vol. xx. Paris, 1855, and in the Philosophical Magazine, August 1856, s. 4. vol. xii, p. 81
- Clausius, R. (1865). The Mechanical Theory of Heat –with its Applications to the Steam Engine and to Physical Properties of Bodies. London: John van Voorst, 1 Paternoster Row. MDCCCLXVII.
- Iribarne, J.V., Godson, W.L. (1973/1989). Atmospheric thermodynamics, second edition, reprinted 1989, Kluwer Academic Publishers, Dordrecht, ISBN 90-277-1296-4, p. 136.
- Bailyn, M. (1994), Part D, pp. 50–56.
- For example, Clausius, R. (1857). Über die Art der Bewegung, welche wir Wärme nennen, Annalen der Physik und Chemie, 100: 353–380. Translated as On the nature of the motion which we call heat, Phil. Mag. series 4, 14: 108–128.
Bibliography
- Adkins, C.J. (1968/1983). Equilibrium Thermodynamics, (1st edition 1968), third edition 1983, Cambridge University Press, Cambridge UK, ISBN 0-521-25445-0.
- Bailyn, M. (1994). A Survey of Thermodynamics, American Institute of Physics Press, New York, ISBN 0-88318-797-3.
- Beattie, J.A., Oppenheim, I. (1979). Principles of Thermodynamics, Elsevier, Amsterdam, ISBN 0–444–41806–7.
- Bryan, G.H. (1907). Thermodynamics. An Introductory Treatise dealing mainly with First Principles and their Direct Applications, B.G. Teubner, Leipzig.
- Buchdahl, H.A. (1966), The Concepts of Classical Thermodynamics, Cambridge University Press, London.
- Callen, H.B. (1960/1985). Thermodynamics and an Introduction to Thermostatistics, (1st edition 1960) 2nd edition 1985, Wiley, New York, ISBN 0-471-86256-8.
- Fowler, R., Guggenheim, E.A. (1939/1965). Statistical Thermodynamics. A version of Statistical Mechanics for Students of Physics and Chemistry, Cambridge University Press, Cambridge UK.
- Guggenheim, E.A. (1967) , Thermodynamics. An Advanced Treatment for Chemists and Physicists (fifth ed.), Amsterdam: North-Holland Publishing Company.
{{citation}}: Cite has empty unknown parameters:|other=and|month=(help) - Haase, R. (1971). Survey of Fundamental Laws, chapter 1 of Thermodynamics, pages 1–97 of volume 1, ed. W. Jost, of Physical Chemistry. An Advanced Treatise, ed. H. Eyring, D. Henderson, W. Jost, Academic Press, New York, lcn 73–117081.
- Kondepudi, D. (2008), Introduction to Modern Thermodynamics, Chichester UK: Wiley, ISBN 978–0–470–01598–8.
{{citation}}: Check|isbn=value: invalid character (help); Cite has empty unknown parameters:|other=and|month=(help) - Kondepudi, D., Prigogine, I. (1998). Modern Thermodynamics: From Heat Engines to Dissipative Structures, John Wiley & Sons, Chichester, ISBN 0–471–97393–9.
- Maxwell, J.C. (1871), Theory of Heat (first ed.), London: Longmans, Green and Co.
{{citation}}: Cite has empty unknown parameters:|other=and|month=(help) - Münster, A. (1970), Classical Thermodynamics, translated by E.S. Halberstadt, Wiley–Interscience, London, ISBN 0-471-62430-6.
- Owen, D.R. (1984). A First Course in the Mathematical Foundations of Thermodynamics, Springer, New York, ISBN 0-387-90897-8.
- Partington, J.R. (1949), An Advanced Treatise on Physical Chemistry., vol. volume 1, Fundamental Principles. The Properties of Gases, London: Longmans, Green and Co.
{{citation}}:|volume=has extra text (help); Cite has empty unknown parameters:|other=and|month=(help) - Pippard, A.B. (1957/1966). Elements of Classical Thermodynamics for Advanced Students of Physics, original publication 1957, reprint 1966, Cambridge University Press, Cambridge UK.
- Planck, M., (1897/1903). Treatise on Thermodynamics, translated by A. Ogg, first English edition, Longmans, Green and Co., London.
- Planck, M., (1923/1927). Treatise on Thermodynamics, translated by A. Ogg, third English edition, Longmans, Green and Co., London.
- Reif, F. (1965). Fundamentals of Statistical and Thermal Physics. New York: McGraw-Hll, Inc.
- Tisza, L. (1966). Generalized Thermodynamics, M.I.T. Press, Cambridge MA.
- Truesdell, C. (1980). The Tragicomical History of Thermodynamics 1822-1854, Springer, New York, ISBN 0–387–90403–4.
- Wilson, A.H. (1957). Thermodynamics and Statistical Mechanics, Cambridge University Press, London.
External links
- Heat on In Our Time at the BBC
- Plasma heat at 2 gigakelvins - Article about extremely high temperature generated by scientists (Foxnews.com)
- Correlations for Convective Heat Transfer - ChE Online Resources
- An Introduction to the Quantitative Definition and Analysis of Heat written for High School Students
 . This should not be confused with a time derivative of a function of state (which can also be written with the dot notation) since heat is not a function of state. Heat flux is defined as rate of heat transfer per unit cross-sectional area, resulting in the unit watts per square metre.
. This should not be confused with a time derivative of a function of state (which can also be written with the dot notation) since heat is not a function of state. Heat flux is defined as rate of heat transfer per unit cross-sectional area, resulting in the unit watts per square metre.














