| Revision as of 19:53, 4 November 2010 editCBM (talk | contribs)Extended confirmed users, File movers, Pending changes reviewers, Rollbackers55,390 edits →RfC: c← Previous edit | Revision as of 19:55, 4 November 2010 edit undoCBM (talk | contribs)Extended confirmed users, File movers, Pending changes reviewers, Rollbackers55,390 edits →RfC: cNext edit → | ||
| Line 169: | Line 169: | ||
| :::Let me rewrite this for you. Suppose we start with a square of side ''c'' and area ''c''<sup>2</sup>. Then a right angle can be inserted with acute angle on its four corners. One can deduce that 'A = a<sup>2</sup> + b<sup>2</sup> etc. You are not assuming an axis-aligned square and axis-aligned right triangles in your version of this paragraph (and if you were I'd question your assumptions), so the "rotate it about a vertical axis" looks totally irrelevant to me: why does rotating a square make it any easier or any more difficult to insert right triangles in it? It doesn't. You are locked into some Cartesian mindset that has nothing to do with the actual geometry. —] (]) 18:41, 4 November 2010 (UTC) | :::Let me rewrite this for you. Suppose we start with a square of side ''c'' and area ''c''<sup>2</sup>. Then a right angle can be inserted with acute angle on its four corners. One can deduce that 'A = a<sup>2</sup> + b<sup>2</sup> etc. You are not assuming an axis-aligned square and axis-aligned right triangles in your version of this paragraph (and if you were I'd question your assumptions), so the "rotate it about a vertical axis" looks totally irrelevant to me: why does rotating a square make it any easier or any more difficult to insert right triangles in it? It doesn't. You are locked into some Cartesian mindset that has nothing to do with the actual geometry. —] (]) 18:41, 4 November 2010 (UTC) | ||
| ::::Hi David: A Cartesian mindset is not what I am using. It appears to boil down to whether the observer rotates with the square so it appears fixed in orientation and examines various possible triangle orientations within the square, or the observer rotates the square and examines its orientation using various triangles that maintain the alignment of their shorter sides. Does the observer rotate the square or rotate with the square? WP seems to be having some trouble, but you can see the new figure by clicking on the small pair of overlaid rectangles in the display case. ] (]) 19:48, 4 November 2010 (UTC) | ::::Hi David: A Cartesian mindset is not what I am using. It appears to boil down to whether the observer rotates with the square so it appears fixed in orientation and examines various possible triangle orientations within the square, or the observer rotates the square and examines its orientation using various triangles that maintain the alignment of their shorter sides. Does the observer rotate the square or rotate with the square? WP seems to be having some trouble, but you can see the new figure by clicking on the small pair of overlaid rectangles in the display case. ] (]) 19:48, 4 November 2010 (UTC) | ||
| :::::I don't understand why anything has to be rotated. Any square of side length ''c'' has area ''c''<sup>2</sup> regardless of orientation. Similarly the area of a triangle is not affected by rotation or translating it. — Carl <small>(] · ])</small> 19:55, 4 November 2010 (UTC) | |||
| ::Now, one can arrive at the relation ''A = a<sup>2</sup> + b<sup>2</sup> = c<sup>2</sup>'' directly without invoking rotational invariance, but one can also do it this way using rotational invariance. The fact that rotational invariance is not required by one proof and is required by the other is not an argument for or against either method, would you agree? | ::Now, one can arrive at the relation ''A = a<sup>2</sup> + b<sup>2</sup> = c<sup>2</sup>'' directly without invoking rotational invariance, but one can also do it this way using rotational invariance. The fact that rotational invariance is not required by one proof and is required by the other is not an argument for or against either method, would you agree? | ||
Revision as of 19:55, 4 November 2010
| Pythagorean theorem is a former featured article. Please see the links under Article milestones below for its original nomination page (for older articles, check the nomination archive) and why it was removed. | ||||||||||||||||||||||
| Pythagorean theorem has been listed as one of the Mathematics good articles under the good article criteria. If you can improve it further, please do so. If it no longer meets these criteria, you can reassess it. | ||||||||||||||||||||||
| ||||||||||||||||||||||
| This article has not yet been rated on Misplaced Pages's content assessment scale. It is of interest to the following WikiProjects: | |||||||||||
Please add the quality rating to the {{WikiProject banner shell}} template instead of this project banner. See WP:PIQA for details.
| |||||||||||
| This article was reviewed by Nature on December 14, 2005. Comments: It was found to have 1 error; this error has been fixed. For more information about external reviews of Misplaced Pages articles and about this review in particular, see this page. |
|
Archives |
|
This page has archives. Sections older than 60 days may be automatically archived by Lowercase sigmabot III when more than 4 sections are present. |
Converse?
The first statement in the converse section is not quite right:
For any three positive numbers a, b, and c such that , there exists a triangle with sides a, b and c, and every such triangle has a right angle between the sides of lengths a and b.
The first part of this statement is not the converse to the Pythagorean theorem. It actually follows directly from the Pythagorean theorem. Simply take a right triangle with side lengths a and b. By Pythagoras' theorem, the hypotenuse must be c. Showing that any triangle with side lengths a,b, and c satisfying has a right angle is the converse. This is stated a few lines down, but I wonder why the section on the converse does not start with this. I will change if no one objects. Paul Laroque (talk) 03:02, 28 September 2010 (UTC)
- You have a point and the cited reference doesn't support the addition either. Euclid's version of the converse is noteworthy simply because it's Euclid's, and another one using modern notation is useful, but it seems a bit redundant to have three versions of it.--RDBury (talk) 08:27, 28 September 2010 (UTC)
Dimensional analysis
I have added a dimensional proof of the Pythagorean theorem. I believe it, at least for me (a physicist), much more convincing than all those complicated triangle rearrangements which are used in most other proofs. Unfortunately, though I have given an academic reference, I don't know who originally proposed it. If someone knows, please add the reference.--GianniG46 (talk) 12:50, 13 October 2010 (UTC)
- The proof is very interesting, but I'm afraid that it isn't actually a proof. The issue is that you have assumed that the form of the area is where f is dimensionless. Ignoring the question of whether dimensional analysis is a valid proof technique at all (which I would say it is not), the assumption about the form of the area is far from obvious. It would be equally possible for the area to be of the form where g has units of length (for example, if g includes some constant that has units of length). It would even be possible, in principle, for the area to be of the form where h has units of (length). The deeper issue is that dimensional analysis is not a valid proof technique, but even if it was there is no reason that the area would need to be in the form specified in that argument. In general all that can be said is that the area can be written as some function of c, α, and β – the form of the function cannot be known ahead of time. — Carl (CBM · talk) 14:34, 13 October 2010 (UTC)
- I don't quite understand why it's called "dimensional analysis", but it is a general property of the n-dimensional Lebesgue (or Hausdorff) measure that if A and B are similar objects with coefficient of similarity r, then λB = rλA. Triangles with the same angles are similar, and the coefficient is the ratio of their longest sides, which indeed implies that the area is cf(α,β) for some f.—Emil J. 14:51, 13 October 2010 (UTC)
- Yes; I was commenting only on the proof as it was written, which seemed to claim that the form of the area function follows solely from its units.
- I don't quite understand why it's called "dimensional analysis", but it is a general property of the n-dimensional Lebesgue (or Hausdorff) measure that if A and B are similar objects with coefficient of similarity r, then λB = rλA. Triangles with the same angles are similar, and the coefficient is the ratio of their longest sides, which indeed implies that the area is cf(α,β) for some f.—Emil J. 14:51, 13 October 2010 (UTC)
- If we rewrite the proof in terms of similarity, it has the same essence as the "similarity proof" that the article already includes. I think it would be nice to expand out that proof sketch to something more detailed, since this is a very pretty proof. I'll see what I can do. — Carl (CBM · talk) 17:03, 13 October 2010 (UTC)
- So, now the proof is correct, provided that you change the words "dimensions of a length squared" with "scales by a factor of s^2". Now I ask: this is the same concept or not? And, if the question was only the language, it was not possible to correct my text, rather than deleting it, keep its concepts, and rewrite it again? I think it is incorrect: a) to delete without a previous discussion a contribution with citations and not obviously wrong . b) to use the material to write another contribution instead of trying to amend the original contribution. --GianniG46 (talk) 20:45, 13 October 2010 (UTC)
- I thought that, modulo the issue with dimensional analysis, the idea behind the proof was nice. At first, I was somewhat blinded by the dimensional analysis issue and didn't I realize that the text could be transformed into a better proof of the "similarity" method that was already sketched obliquely in the article. The invocation of dimensional analysis was a red herring for me.
- Once EmilJ pointed out how the proof method could be salvaged, I went right back and did that. I apologize for not taking the most direct route to the current state of the article. However, I think that the outcome is good so far: we have a more clear version of what you wrote, merged with a previously less clear section on similarity. — Carl (CBM · talk) 21:09, 13 October 2010 (UTC)
This proof is not as well described as it might be. For example, when the hypotenuse is scaled by c, it is assumed, but not pointed out, that all the angles (not just the right angle) are held fixed, and that this implies the other two sides are also scaled in the same proportion. That is tantamount to a theorem that the sine of an angle is a function of the angle only, and not the lengths of the sides, an observation tantamount to Pythagoras' theorem, and the reason the proof works. This article in general is unclear about the difference between equivalence of statements and proofs strictly based upon the fundamental axioms themselves. That failure unfortunately undermines the notion of a deductive system. Brews ohare (talk) 17:31, 14 October 2010 (UTC)
Proof by area vs. proof by rotation
Perhaps the interested parties can bring this discussion to the talk page instead of brewing an edit war? Orange Suede Sofa (talk) 18:13, 3 November 2010 (UTC)
- I don't think we're anywhere near an edit war, but for the record here's my reason for restoring Hgilbert's change, expanded from the edit summary. The proof has little to do with rotation. The only things that need to be rotated are the triangles, but that is true of many of the proofs that involve triangles, such as the one immediately above. The square does not need to be rotated as it is clear it is a square, with sides length c and right angled corners, even inclined.--JohnBlackburnedeeds 18:31, 3 November 2010 (UTC)
- FWIW, I agree with your reasoning.—Emil J. 18:41, 3 November 2010 (UTC)
Proof by rotation

It seems that this example can be misinterpreted, so I've tried to rewrite this section to be clearer.
The top square in this figure is a rotated version of the lower square. The angle of rotation can be expressed as one or the other of the two acute angles of the right triangles inserted in the top figure.
The area of the square is not changed by its rotation, so the area computed from the top square is the same as that of the bottom square. It is of interest to point out that the rotational invariance of area is a factor in this proof. Brews ohare (talk) 18:46, 3 November 2010 (UTC)
- As noted above the proof has nothing to do with such rotation, as in it works fine without rotating the square. The source also says nothing about rotating the square, just that the triangles are rotated as in many of the other proofs, so the rotation of the square is something added by an editor which is unnecessary for the proof.--JohnBlackburnedeeds 19:00, 3 November 2010 (UTC)
- John: The proof that doesn't involve rotation, does not involve rotation. As such it is a repetition of proofs by rearrangement already in this article, and can be deleted altogether. Its only interest here is the rotational connection. Brews ohare (talk) 19:22, 3 November 2010 (UTC)
- I'm not sure what you mean by your first sentence. I don't see any need to remove it: it's sourced and is one of two algebraic proofs, i.e. one where the areas of the figures are derived and expressed algebraically, which then gives the result. The two proofs are similar but different both in the figures used and the algebra.--JohnBlackburnedeeds 19:41, 3 November 2010 (UTC)
- John: The proof that doesn't involve rotation, does not involve rotation. As such it is a repetition of proofs by rearrangement already in this article, and can be deleted altogether. Its only interest here is the rotational connection. Brews ohare (talk) 19:22, 3 November 2010 (UTC)
John, as you are probably aware, Euclidean geometry tacitly assumes that translations and rotations do not alter the geometric properties of figures, such as their areas. I believe the concept is Euclidean congruence transformations or Euclidean motions.
In this particular example, the top panel is a square of area a + b that is a rotation of the square in the lower panel of area c. Because rotation doesn't alter the area of a figure, a + b = c.
Now you may argue that this isn't the only way to look at it, but I'd hope you would agree that it is a valid way to look at it. More than that, it is an interesting way to look at because it invokes a different aspect of Euclidean geometry not before discussed in this article that leads to Pythagoras' theorem.
So I have added this view to the discussion. Brews ohare (talk) 12:17, 4 November 2010 (UTC)
- I have removed this "interesting perspective" since it is unsourced: giving two sourced statements and drawing a conclusion from them is not allowed per wp:SYNTH in wp:NOR. DVdm (talk) 12:28, 4 November 2010 (UTC)
DVdm: You have mischaracterized the example. It consists of the following statements:
1. The top panel is a rotation of the square in the lower panel. I believe that is an obvious statement that you would not disagree with and would not require a source for.
2. The invariance of figures under Euclidean motions is attested to by the sources provided. In any event, such sourcing is overkill inasmuch as Euclid made this assumption centuries ago without comment. The main purpose of the sources is to expand the topic for a reader that might find a more general approach of interest.
3. The conclusion is hardly WP:SYN since it amounts to A = c and A = a + b so c = a + b. Simple syllogism is allowed by WP:SYN.
On the basis that your reversion is incorrectly based I have replaced the observation. Brews ohare (talk) 12:40, 4 November 2010 (UTC)
- You don't need to explain things here. You have given two sourced statements and have drawn an unsourced conclusion. That is a schoolbook example of wp:SYNTH. Please remove that entry again. DVdm (talk) 12:46, 4 November 2010 (UTC)
- Pardon me, but can you identify the unsourced conclusion? Is it that the two areas are equal? Is it that rotations leave area unaltered? What is it that you find to be an unwarranted conclusion, please? Brews ohare (talk) 12:56, 4 November 2010 (UTC)
- Referring to this edit:
- Sourced statement #1: "... observing that the top square of area b^2 + a^2 is simply a rotation of the lower square of area c^2"
- Sourced statement #2: "A tacit assumption of Euclidean geometry is that geometric properties of figures (area being one) are not changed by translations and rotations."
- Unsourced conclusion: "As a consequence of this invariance under rotations, b^2 + a^2 = c^2"
- A schoolbook example of wp:SYNTH. DVdm (talk) 13:21, 4 November 2010 (UTC)
- DVdm: No it is isn't. It's a Routine math operation, an obvious use of a common syllogism that no-one in their right mind would challenge. Brews ohare (talk) 13:35, 4 November 2010 (UTC)
- ==> "... routine mathematical calculations, such as adding numbers, converting units, or calculating a person's age ...". They don't say anything a logical deductions like in "Do not combine material from multiple sources to reach or imply a conclusion not explicitly stated by any of the sources. If one reliable source says A, and another reliable source says B, do not join A and B together to imply a conclusion C that is not mentioned by either of the sources." If you don't like this, then feel free to file for policy change at, perhaps, Wikipedia_talk:No_original_research. DVdm (talk) 15:07, 4 November 2010 (UTC)
- DVdm: No it is isn't. It's a Routine math operation, an obvious use of a common syllogism that no-one in their right mind would challenge. Brews ohare (talk) 13:35, 4 November 2010 (UTC)
- Referring to this edit:
- Pardon me, but can you identify the unsourced conclusion? Is it that the two areas are equal? Is it that rotations leave area unaltered? What is it that you find to be an unwarranted conclusion, please? Brews ohare (talk) 12:56, 4 November 2010 (UTC)
- The lower square is irrelevant. The upper square is a square with side c and so area c, there's no need to rotate it to see this. There are now four editors who disagree with your irrelevant additions or rewriting of this proof, so please stop editing against consensus.--JohnBlackburnedeeds 12:48, 4 November 2010 (UTC)
- John: No argument from me that you can establish the result differently. The point is that this example also illustrates a different point of view, invariance under rotations. You do not suggest that this argument is mistaken, do you? The "four editors" haven't addressed this issue; the two editors that have are you and DVdm. Brews ohare (talk) 12:56, 4 November 2010 (UTC)
- Four being User:Hgilbert who fixed the erroneous association with rotation, I who restored his improvements after you reverted them User:EmilJ who agreed with my reasons for doing so and User:DVdm who also reverted your unnecessary changes. We already have a clear consensus on this, there's no need to waste other editors' time with it. Please remove the RfC.--JohnBlackburnedeeds 14:03, 4 November 2010 (UTC)
- User:Hgilbert and User:EmilJ simply agreed that the algebraic version followed the source given. There is no statement by anyone, except possibly yourself, that the connection to rotation is "erroneous". Is that really your considered opinion as a mathematician?? I'll let other editors comment directly upon the matter as actually proposed through the RfC. Brews ohare (talk) 14:22, 4 November 2010 (UTC)
- I didn't care about any sources. The problem is that the rotation of the outer square is pointless and misleading, and misses the actual reason why the argument works.—Emil J. 14:29, 4 November 2010 (UTC)
- EmilJ: I understand that the argument already presented works. That doesn't mean another argument is false, pointless, or misleading; it is just different. An argument based upon rotation may be strange to you, but it is as valid as the first argument. Rotational invariance is a well recognized concept within Euclidean geometry, and pointing out its connection here seems to me to broaden the reader's understanding and provide some new directions to think in. Brews ohare (talk) 14:45, 4 November 2010 (UTC)
- I didn't care about any sources. The problem is that the rotation of the outer square is pointless and misleading, and misses the actual reason why the argument works.—Emil J. 14:29, 4 November 2010 (UTC)
- User:Hgilbert and User:EmilJ simply agreed that the algebraic version followed the source given. There is no statement by anyone, except possibly yourself, that the connection to rotation is "erroneous". Is that really your considered opinion as a mathematician?? I'll let other editors comment directly upon the matter as actually proposed through the RfC. Brews ohare (talk) 14:22, 4 November 2010 (UTC)
- Four being User:Hgilbert who fixed the erroneous association with rotation, I who restored his improvements after you reverted them User:EmilJ who agreed with my reasons for doing so and User:DVdm who also reverted your unnecessary changes. We already have a clear consensus on this, there's no need to waste other editors' time with it. Please remove the RfC.--JohnBlackburnedeeds 14:03, 4 November 2010 (UTC)
- John: No argument from me that you can establish the result differently. The point is that this example also illustrates a different point of view, invariance under rotations. You do not suggest that this argument is mistaken, do you? The "four editors" haven't addressed this issue; the two editors that have are you and DVdm. Brews ohare (talk) 12:56, 4 November 2010 (UTC)
I've fixed one aspect of it, which was the confusing diagram with the redundant second square. I've replaced this with a simpler diagram which only has the inclined square, along with a copy of one of the triangles which it's easier to label the sides of. The diagram now matches the text and proof much better. As a bonus it is an SVG image, to make it easier for editors to improve or make other use of.--JohnBlackburnedeeds 14:18, 4 November 2010 (UTC)
- That is not a "fix", John, it is an interruption of an RfC to make its assessment more difficult by altering the context within which it is to be judged and prejudice the outcome. Brews ohare (talk) 14:22, 4 November 2010 (UTC)
- As noted the RfC is unnecessary as there is already clear consensus, there is nothing to stop editors improving the article while one is in progress, and the old image is still below for editors to consider. The image needed replacing anyway: the symbols "a", "b" and "c" were not italicised as they should be, had a couple of other errors, and was in PNG which makes it impossible for other editors to improve.--JohnBlackburnedeeds 14:33, 4 November 2010 (UTC)
RfC
|
It is proposed that the discussion of an example in Pythagorean theorem#Proof by areas be extended to include an alternative proof illustrating a role for rotation. Please comment upon the interest of this addition to readers, and whether you regard the discussion to be a violation of WP:SYN. The paragraph is to be placed below the algebraic discussion already present. The addition is appended below. Brews ohare (talk) 13:36, 4 November 2010 (UTC)

- An interesting perspective upon this example is provided by observing that the top square already established to have an area b + a is simply a rotation of the lower square of area c. A tacit assumption of Euclidean geometry is that geometric properties of figures (area being one) are not changed by translations and rotations. As a consequence of this invariance under rotations, b + a = c.
- References & notes
- The angle of rotation of the square in the top panel of this example is the same as the smaller acute angle of the four right triangles.
- The general topic is that of Euclidean motions; see, for example: Luciano da Fontoura Costa, Roberto Marcondes Cesar (2001). Shape analysis and classification: theory and practice. CRC Press. p. 314. ISBN 0849334934. and Helmut Pottmann, Johannes Wallner (2010). Computational Line Geometry. Springer. p. 60. ISBN 3642040179.
Comments
Please add your comments below with a leading *.
- Include: The example provides a simple and intuitive proof of the Pythagorean theorem that uses the interesting property of invariance of geometrical properties under Euclidean motions. The proposed addition is a useful supplement to the preceding algebraic treatment because it ties into this topic of invariance. There is nothing here that violates WP:SYN. Brews ohare (talk) 13:36, 4 November 2010 (UTC)
- I think there are too many proofs in that section already. Also, I don't think that it's necessary to emphasize Euclidean geometry more than it is already emphasized. — Carl (CBM · talk) 14:00, 4 November 2010 (UTC)
- CBM: Somewhere in the article it says there are more proofs for this theorem than almost any other. The choice of proofs here is selective, based upon (i) historical importance (ii) unusual clarity or (iii) variety of approach, that is, introducing some connections that are a bit unusual. It is on this last basis that I've made this suggestion to connect the Pythagorean theorem to a proof by rotational invariance, which strikes me as bringing out a different aspect of the theorem that might broaden the reader's perspective and lead them in some unexpected directions. That's the value of the thing, it's not (hopefully) just "another proof". Brews ohare (talk) 15:16, 4 November 2010 (UTC)
- There are other proofs that mention similarity (of which rotational invariance is just a special case), and other proofs that do dissections of the square, already in the article. I don't think the proof "by areas" is much different than the two proofs immediately above it. — Carl (CBM · talk) 19:52, 4 November 2010 (UTC)
- Reject per "Do not combine material from multiple sources to reach or imply a conclusion not explicitly stated by any of the sources. If one reliable source says A, and another reliable source says B, do not join A and B together to imply a conclusion C that is not mentioned by either of the sources." DVdm (talk) 15:10, 4 November 2010 (UTC)
- If a math argument states A = c and A = a + b & therefore the conclusion is that c = a + b, calling this routine math operation a violation of WP:SYN is ridiculous. Brews ohare (talk) 15:21, 4 November 2010 (UTC)
- Don't include. The rotation is completely irrelevant both to the theorem and to its proof, and it makes it much more difficult to see that the two squares have the same side length (instead, one is led to the erroneous conclusion that the lower square has side length b). —David Eppstein (talk) 15:22, 4 November 2010 (UTC)
- The lower square side length is labeled c in the diagram, so confusion should be avoidable. As this proof is intended to be a proof using the concept of rotational invariance, evidently rotational invariance is not irrelevant to such a proof. It looks like what you mean is that rotational invariance is not the method used in the prior proof, already in the article, but that is not in dispute here. Brews ohare (talk) 15:40, 4 November 2010 (UTC)
- Please don't try to tell me what I see or don't see with my own eyes. Yes, obviously it's labeled c. Nevertheless it took me a long time to realize that the side length is not b. And I see nothing in the illustration that involves rotation in any necessary way. I should add that responding to all negative comments as you seem to be doing by repeating your same arguments over again comes across as somewhat tendentious. —David Eppstein (talk) 15:53, 4 November 2010 (UTC)
- David: I'm sorry you see my pointing out a label as somehow an insult; that wasn't my intention at all. Maybe the illustration is imperfect. The imperfections of the diagram have nothing to do with the argument, however, and to say that an argument based upon rotational invariance is not necessarily connected to rotation simply doesn't compute. Rotation is exactly what this particular proof involves. Aren't you saying the proof already presented in the article doesn't require rotation? That is not at issue. What do you mean? Brews ohare (talk) 16:08, 4 November 2010 (UTC)
- I believe that it only uses right-angle rotations and translations. The irrational rotation shown in the figure is an irrelevant distraction. There is no need to start with the cxc square being axis-aligned, nor is there any need to think about or depict the Cartesian coordinates of anything. —David Eppstein (talk) 16:19, 4 November 2010 (UTC)
- David: I'm sorry you see my pointing out a label as somehow an insult; that wasn't my intention at all. Maybe the illustration is imperfect. The imperfections of the diagram have nothing to do with the argument, however, and to say that an argument based upon rotational invariance is not necessarily connected to rotation simply doesn't compute. Rotation is exactly what this particular proof involves. Aren't you saying the proof already presented in the article doesn't require rotation? That is not at issue. What do you mean? Brews ohare (talk) 16:08, 4 November 2010 (UTC)
- David: I'd like to open this conversation up a little, and frame matters anew.
- Please don't try to tell me what I see or don't see with my own eyes. Yes, obviously it's labeled c. Nevertheless it took me a long time to realize that the side length is not b. And I see nothing in the illustration that involves rotation in any necessary way. I should add that responding to all negative comments as you seem to be doing by repeating your same arguments over again comes across as somewhat tendentious. —David Eppstein (talk) 15:53, 4 November 2010 (UTC)
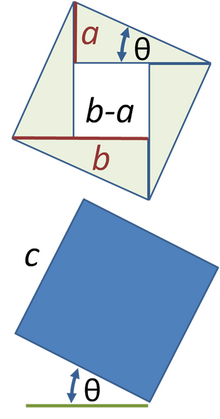
- Suppose we start with a square of side c and area A = c and rotate it about a vertical axis through an angle θ. I'd assume that you'd agree that the area of the square is unaffected according to the notion of Euclidean motions. Then looking at the rotated square, a right triangle can be inserted with acute angle θ, and its hypotenuse along (say) the top side of the rotated square. Taking the two short sides of the right triangle as a and b, one can deduce the rotated square has area A = a + b. Then by invariance of A one finds A = a + b = c.
- Let me rewrite this for you. Suppose we start with a square of side c and area c. Then a right angle can be inserted with acute angle on its four corners. One can deduce that 'A = a + b etc. You are not assuming an axis-aligned square and axis-aligned right triangles in your version of this paragraph (and if you were I'd question your assumptions), so the "rotate it about a vertical axis" looks totally irrelevant to me: why does rotating a square make it any easier or any more difficult to insert right triangles in it? It doesn't. You are locked into some Cartesian mindset that has nothing to do with the actual geometry. —David Eppstein (talk) 18:41, 4 November 2010 (UTC)
- Hi David: A Cartesian mindset is not what I am using. It appears to boil down to whether the observer rotates with the square so it appears fixed in orientation and examines various possible triangle orientations within the square, or the observer rotates the square and examines its orientation using various triangles that maintain the alignment of their shorter sides. Does the observer rotate the square or rotate with the square? WP seems to be having some trouble, but you can see the new figure by clicking on the small pair of overlaid rectangles in the display case. Brews ohare (talk) 19:48, 4 November 2010 (UTC)
- I don't understand why anything has to be rotated. Any square of side length c has area c regardless of orientation. Similarly the area of a triangle is not affected by rotation or translating it. — Carl (CBM · talk) 19:55, 4 November 2010 (UTC)
- Hi David: A Cartesian mindset is not what I am using. It appears to boil down to whether the observer rotates with the square so it appears fixed in orientation and examines various possible triangle orientations within the square, or the observer rotates the square and examines its orientation using various triangles that maintain the alignment of their shorter sides. Does the observer rotate the square or rotate with the square? WP seems to be having some trouble, but you can see the new figure by clicking on the small pair of overlaid rectangles in the display case. Brews ohare (talk) 19:48, 4 November 2010 (UTC)
- Let me rewrite this for you. Suppose we start with a square of side c and area c. Then a right angle can be inserted with acute angle on its four corners. One can deduce that 'A = a + b etc. You are not assuming an axis-aligned square and axis-aligned right triangles in your version of this paragraph (and if you were I'd question your assumptions), so the "rotate it about a vertical axis" looks totally irrelevant to me: why does rotating a square make it any easier or any more difficult to insert right triangles in it? It doesn't. You are locked into some Cartesian mindset that has nothing to do with the actual geometry. —David Eppstein (talk) 18:41, 4 November 2010 (UTC)
- Suppose we start with a square of side c and area A = c and rotate it about a vertical axis through an angle θ. I'd assume that you'd agree that the area of the square is unaffected according to the notion of Euclidean motions. Then looking at the rotated square, a right triangle can be inserted with acute angle θ, and its hypotenuse along (say) the top side of the rotated square. Taking the two short sides of the right triangle as a and b, one can deduce the rotated square has area A = a + b. Then by invariance of A one finds A = a + b = c.
- Now, one can arrive at the relation A = a + b = c directly without invoking rotational invariance, but one can also do it this way using rotational invariance. The fact that rotational invariance is not required by one proof and is required by the other is not an argument for or against either method, would you agree?
- The question of the right diagram to illustrate this matter is a separate issue worth discussing by itself. Brews ohare (talk) 16:43, 4 November 2010 (UTC)
- Suppose we start with a square of side c and area A = c. Let us also take k, l, m, and n such that k + l = m. I'd assume that you'd agree that Fermat's Last Theorem has been proven by Wiles, hence n ≤ 2. Then looking at the original square, a right triangle can be inserted with acute angle θ, and its hypotenuse along (say) the top side of the square. Taking the two short sides of the right triangle as a and b, one can deduce the square has area A = a + b. Then one finds c = A = a + b.
- The question of the right diagram to illustrate this matter is a separate issue worth discussing by itself. Brews ohare (talk) 16:43, 4 November 2010 (UTC)
- Now, one can arrive at the relation A = a + b = c directly without invoking Fermat's Last Theorem, but one can also do it this way using Fermat's Last Theorem. The fact that Fermat's Last Theorem is not required by one proof and is required by the other is not an argument for or against either method, would you agree?—Emil J. 16:48, 4 November 2010 (UTC)
- EmilJ: I understand that you are trying to make it appear that rotational invariance is irrelevant to the argument, but this fact it is used to show the areas are the same. If you want to attempt this with Fermat's last theorem, you'll need to lay a basis. Brews ohare (talk) 17:54, 4 November 2010 (UTC)
- There is no need to show "the areas are the same" as there's no need for the second square. The top square in your diagram is a square with side c so area c, as required. Rotating it achieves nothing and shows nothing.--JohnBlackburnedeeds 17:59, 4 November 2010 (UTC)
- EmilJ: I understand that you are trying to make it appear that rotational invariance is irrelevant to the argument, but this fact it is used to show the areas are the same. If you want to attempt this with Fermat's last theorem, you'll need to lay a basis. Brews ohare (talk) 17:54, 4 November 2010 (UTC)
- The fact that this is being discussed here in the first place, clearly shows that we are indeed dealing with original research. This discussion should not even happen. DVdm (talk) 17:31, 4 November 2010 (UTC)
- Now, one can arrive at the relation A = a + b = c directly without invoking Fermat's Last Theorem, but one can also do it this way using Fermat's Last Theorem. The fact that Fermat's Last Theorem is not required by one proof and is required by the other is not an argument for or against either method, would you agree?—Emil J. 16:48, 4 November 2010 (UTC)
-
- The angle of rotation of the square in the top panel of this example is the same as the smaller acute angle of the four right triangles.
-
- The general topic is that of Euclidean motions; see, for example: Luciano da Fontoura Costa, Roberto Marcondes Cesar (2001). Shape analysis and classification: theory and practice. CRC Press. p. 314. ISBN 0849334934. and Helmut Pottmann, Johannes Wallner (2010). Computational Line Geometry. Springer. p. 60. ISBN 3642040179.

 , there exists a triangle with sides a, b and c, and every such triangle has a right angle between the sides of lengths a and b.
, there exists a triangle with sides a, b and c, and every such triangle has a right angle between the sides of lengths a and b.  where f is dimensionless. Ignoring the question of whether dimensional analysis is a valid proof technique at all (which I would say it is not), the assumption about the form of the area is far from obvious. It would be equally possible for the area to be of the form
where f is dimensionless. Ignoring the question of whether dimensional analysis is a valid proof technique at all (which I would say it is not), the assumption about the form of the area is far from obvious. It would be equally possible for the area to be of the form  where g has units of length (for example, if g includes some constant that has units of length). It would even be possible, in principle, for the area to be of the form
where g has units of length (for example, if g includes some constant that has units of length). It would even be possible, in principle, for the area to be of the form  where h has units of (length). The deeper issue is that dimensional analysis is not a valid proof technique, but even if it was there is no reason that the area would need to be in the form specified in that argument. In general all that can be said is that the area can be written as some function of c, α, and β – the form of the function cannot be known ahead of time. — Carl (
where h has units of (length). The deeper issue is that dimensional analysis is not a valid proof technique, but even if it was there is no reason that the area would need to be in the form specified in that argument. In general all that can be said is that the area can be written as some function of c, α, and β – the form of the function cannot be known ahead of time. — Carl (