| Revision as of 07:50, 12 November 2008 editThijs!bot (talk | contribs)470,128 editsm robot Adding: uk:Ефект Кондо← Previous edit | Revision as of 11:30, 2 March 2009 edit undoDreamer08 (talk | contribs)27 edits →External linksNext edit → | ||
| Line 21: | Line 21: | ||
| * ''Exotic Kondo Effects in Metals'' - Monograph on newer versions of the Kondo effect in non-magnetic contexts especially (ISBN 0-7484-0889-4) | * ''Exotic Kondo Effects in Metals'' - Monograph on newer versions of the Kondo effect in non-magnetic contexts especially (ISBN 0-7484-0889-4) | ||
| * , Nature '''410''', 793 (2001). Nature article exploring the links of the Kondo effect and plutonium | * , Nature '''410''', 793 (2001). Nature article exploring the links of the Kondo effect and plutonium | ||
| * | * | ||
| ] | ] | ||
Revision as of 11:30, 2 March 2009
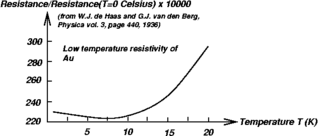
In physics, according to the Kondo effect, the electrical resistance will diverge as the temperature approaches 0 K. The temperature dependence of the resistance including the Kondo effect is written as:
where is the residual resistance, shows the contribution from the Fermi liquid properties, and the term is from the lattice vibrations. Jun Kondo has derived the third term of the logarithmic dependence. Later calculations refined this result to produce a finite resistivity but retained the feature of a resistance minimum at a non-zero temperature. One defines the Kondo temperature as the energy scale limiting the validity of the Kondo results. The Anderson model and accompanying renormalization theory was an important contribution to understanding the underlying physics of the problem.

The Kondo effect is the first known example of asymptotic freedom in physics, in which the coupling becomes non-perturbatively strong at low temperatures/low energies. In the Kondo problem, this refers to the interaction between the localized magnetic impurities and the itinerant electrons. In a more complex form, asymptotic freedom underlies the theory of quantum chromodynamics, or the so-called strong nuclear force so that quarks, the fundamental constituents of nuclear matter, interact weakly at high energies and strongly at low energies, preventing the unbinding of baryons (fermions like protons or neutrons with three quarks) or mesons (bosons like pions with two quarks), the composite particles of nuclear matter. For this discovery, Frank Wilczek, David Gross, and David Politzer shared the 2004 Nobel Prize in physics.
Extended to a lattice of magnetic impurities, the Kondo effect is believed to underly the formation of heavy fermions in intermetallic compounds based especially upon rare earth elements like cerium, praseodymium, and ytterbium, and actinide elements like uranium. In these materials, the nonperturbative growth of the interaction leads to quasi-electrons with masses up to thousands of times the free electron mass, ie, the electrons are dramatically slowed by the interactions. In a number of instances they actually are superconductors. More recently, it is believed that a manifestation of the Kondo effect is necessary for understanding the unusual metallic delta-phase of plutonium.
External links
- Jun Kondo's web page - follow links in English, other parts are written in Japanese
- Kondo Effect - 40 Years after the Discovery - special issue of the Journal of the Physical Society of Japan
- The Kondo Problem to Heavy Fermions - Monograph on the Kondo effect by A.C. Hewson (ISBN 0-521-59947-4)
- Exotic Kondo Effects in Metals - Monograph on newer versions of the Kondo effect in non-magnetic contexts especially (ISBN 0-7484-0889-4)
- Correlated electrons in -plutonium within a dynamical mean-field picture, Nature 410, 793 (2001). Nature article exploring the links of the Kondo effect and plutonium
- Kondo effect on arxiv.org

 is the residual resistance,
is the residual resistance,  shows the contribution from the Fermi liquid properties, and the term
shows the contribution from the Fermi liquid properties, and the term  is from the lattice vibrations.
is from the lattice vibrations.  , the Fermi velocity, experiencing only a mild antiferromagnetic correlation in the vicinity of the impurity. In contrast, as the temperature tends to zero the impurity magnetic moment and one conduction electron moment bind very strongly to form an overall non-magnetic state.
, the Fermi velocity, experiencing only a mild antiferromagnetic correlation in the vicinity of the impurity. In contrast, as the temperature tends to zero the impurity magnetic moment and one conduction electron moment bind very strongly to form an overall non-magnetic state. -plutonium within a dynamical mean-field picture
-plutonium within a dynamical mean-field picture