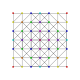| #
|
Coxeter-Dynkin diagram
Schläfli symbol
Johnson name
|
Ak orthogonal projection graphs
|
A8
|
A7
|
A6
|
A5
|
A4
|
A3
|
A2
|
| 1
|
              
t0{3,3,3,3,3,3,3}
8-simplex
|

|

|

|

|

|

|
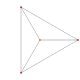
|
| 2
|
              
t1{3,3,3,3,3,3,3}
Rectified 8-simplex
|

|

|

|
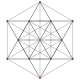
|

|

|
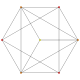
|
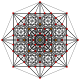
| 3
|
              
t2{3,3,3,3,3,3,3}
Birectified 8-simplex
|

|
|

|

|

|

|

|
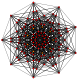
| 4
|
              
t3{3,3,3,3,3,3,3}
Trirectified 8-simplex
|
|

|

|

|

|

|

|
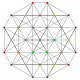
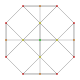
| 5
|
              
t0,1{3,3,3,3,3,3,3}
Truncated 8-simplex
|

|

|

|
|

|
|

|
| 6
|
              
t0,2{3,3,3,3,3,3,3}
Cantellated 8-simplex
|

|

|

|

|

|

|
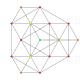
|
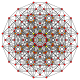
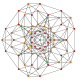
| 7
|
              
t1,2{3,3,3,3,3,3,3}
Bitruncated 8-simplex
|

|
|

|

|
|

|

|
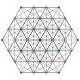
| 8
|
              
t0,3{3,3,3,3,3,3,3}
Runcinated 8-simplex
|

|

|

|
|

|

|

|
| 9
|
              
t1,3{3,3,3,3,3,3,3}
Bicantellated 8-simplex
|

|

|

|

|

|

|

|
| 10
|
              
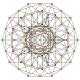
t2,3{3,3,3,3,3,3,3}
Tritruncated 8-simplex
|

|

|

|

|
|

|

|
| 11
|
              
t0,4{3,3,3,3,3,3,3}
Stericated 8-simplex
|

|

|

|

|

|

|

|
| 12
|
              
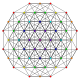
t1,4{3,3,3,3,3,3,3}
Biruncinated 8-simplex
|

|

|

|
|

|

|

|
| 13
|
              
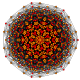
t2,4{3,3,3,3,3,3,3}
Tricantellated 8-simplex
|

|

|
|

|

|

|

|
| 14
|
              
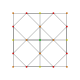
t3,4{3,3,3,3,3,3,3}
Quadritruncated 8-simplex
|

|

|

|

|

|
|

|
| 15
|
              
t0,5{3,3,3,3,3,3,3}
Pentellated 8-simplex
|

|

|

|

|

|

|

|
| 16
|
              
t1,5{3,3,3,3,3,3,3}
Bistericated 8-simplex
|

|

|

|
|

|

|

|
| 17
|
              
t2,5{3,3,3,3,3,3,3}
Triruncinated 8-simplex
|

|

|

|

|

|

|
|
| 18
|
              
t0,6{3,3,3,3,3,3,3}
Hexicated 8-simplex
|
|

|
|

|

|

|

|
| 19
|
              
t1,6{3,3,3,3,3,3,3}
Bipentellated 8-simplex
|

|

|
|

|

|

|
|
| 20
|
              
t0,7{3,3,3,3,3,3,3}
Heptellated 8-simplex
|

|

|
|

|

|

|

|
| 21
|
              
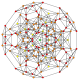
t0,1,2{3,3,3,3,3,3,3}
Cantitruncated 8-simplex
|

|

|
|

|
|

|

|
| 22
|
              
t0,1,3{3,3,3,3,3,3,3}
Runcitruncated 8-simplex
|

|

|

|

|

|

|

|
| 23
|
              
t0,2,3{3,3,3,3,3,3,3}
Runcicantellated 8-simplex
|

|

|

|

|

|

|

|
| 24
|
              
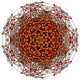
t1,2,3{3,3,3,3,3,3,3}
Bicantitruncated 8-simplex
|

|

|
|

|

|

|

|
| 25
|
              
t0,1,4{3,3,3,3,3,3,3}
Steritruncated 8-simplex
|

|

|

|

|

|

|

|
| 26
|
              
t0,2,4{3,3,3,3,3,3,3}
Stericantellated 8-simplex
|

|

|

|

|

|

|

|
| 27
|
              
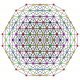
t1,2,4{3,3,3,3,3,3,3}
Biruncitruncated 8-simplex
|

|

|

|
|

|
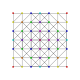
|

|
| 28
|
              
t0,3,4{3,3,3,3,3,3,3}
Steriruncinated 8-simplex
|

|

|

|

|

|

|

|
| 29
|
              
t1,3,4{3,3,3,3,3,3,3}
Biruncicantellated 8-simplex
|

|

|

|
|

|

|

|
| 30
|
              
t2,3,4{3,3,3,3,3,3,3}
Tricantitruncated 8-simplex
|

|

|

|

|
|
|

|
| 31
|
              
t0,1,5{3,3,3,3,3,3,3}
Pentitruncated 8-simplex
|

|
|

|

|

|

|

|
| 32
|
              
t0,2,5{3,3,3,3,3,3,3}
Penticantellated 8-simplex
|

|

|

|

|

|

|

|
| 33
|
              
t1,2,5{3,3,3,3,3,3,3}
Bisteritruncated 8-simplex
|

|

|

|

|

|

|

|
| 34
|
              
t0,3,5{3,3,3,3,3,3,3}
Pentiruncinated 8-simplex
|

|

|

|

|

|
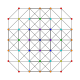
|

|
| 35
|
              
t1,3,5{3,3,3,3,3,3,3}
Bistericantellated 8-simplex
|

|

|

|

|

|

|

|
| 36
|
              
t2,3,5{3,3,3,3,3,3,3}
Triruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 37
|
              
t0,4,5{3,3,3,3,3,3,3}
Pentistericated 8-simplex
|

|

|

|

|

|
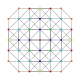
|

|
| 38
|
              
t1,4,5{3,3,3,3,3,3,3}
Bisteriruncinated 8-simplex
|
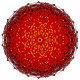
|

|

|

|

|

|

|
| 39
|
              
t0,1,6{3,3,3,3,3,3,3}
Hexitruncated 8-simplex
|

|

|

|

|

|

|

|
| 40
|
              
t0,2,6{3,3,3,3,3,3,3}
Hexicantellated 8-simplex
|

|

|

|

|

|

|

|
| 41
|
              
t1,2,6{3,3,3,3,3,3,3}
Bipentitruncated 8-simplex
|

|

|

|

|

|

|

|
| 42
|
              
t0,3,6{3,3,3,3,3,3,3}
Hexiruncinated 8-simplex
|

|

|
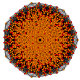
|

|

|

|

|
| 43
|
              
t1,3,6{3,3,3,3,3,3,3}
Bipenticantellated 8-simplex
|

|

|

|
|

|

|

|
| 44
|
              
t0,4,6{3,3,3,3,3,3,3}
Hexistericated 8-simplex
|

|

|

|

|
|

|

|
| 45
|
              
t0,5,6{3,3,3,3,3,3,3}
Hexipentellated 8-simplex
|

|

|

|

|

|

|

|
| 46
|
              
t0,1,7{3,3,3,3,3,3,3}
Heptitruncated 8-simplex
|

|

|

|

|

|
|

|
| 47
|
              
t0,2,7{3,3,3,3,3,3,3}
Hepticantellated 8-simplex
|

|

|

|

|

|

|
|
| 48
|
              
t0,3,7{3,3,3,3,3,3,3}
Heptiruncinated 8-simplex
|

|

|

|

|

|

|

|
| 49
|
              
t0,1,2,3{3,3,3,3,3,3,3}
Runcicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 50
|
              
t0,1,2,4{3,3,3,3,3,3,3}
Stericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 51
|
              
t0,1,3,4{3,3,3,3,3,3,3}
Steriruncitruncated 8-simplex
|

|

|
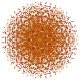
|
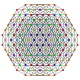
|
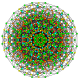
|

|

|
| 52
|
              
t0,2,3,4{3,3,3,3,3,3,3}
Steriruncicantellated 8-simplex
|

|

|

|

|

|
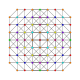
|

|
| 53
|
              
t1,2,3,4{3,3,3,3,3,3,3}
Biruncicantitruncated 8-simplex
|

|

|

|

|
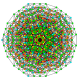
|

|

|
| 54
|
              
t0,1,2,5{3,3,3,3,3,3,3}
Penticantitruncated 8-simplex
|

|

|

|

|
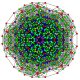
|

|

|
| 55
|
              
t0,1,3,5{3,3,3,3,3,3,3}
Pentiruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 56
|
              
t0,2,3,5{3,3,3,3,3,3,3}
Pentiruncicantellated 8-simplex
|

|

|

|
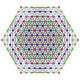
|

|
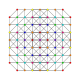
|

|
| 57
|
              
t1,2,3,5{3,3,3,3,3,3,3}
Bistericantitruncated 8-simplex
|

|

|

|
|

|

|

|
| 58
|
              
t0,1,4,5{3,3,3,3,3,3,3}
Pentisteritruncated 8-simplex
|

|

|
|

|

|

|

|
| 59
|
              
t0,2,4,5{3,3,3,3,3,3,3}
Pentistericantellated 8-simplex
|

|

|

|

|
|
|

|
| 60
|
              
t1,2,4,5{3,3,3,3,3,3,3}
Bisteriruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 61
|
              
t0,3,4,5{3,3,3,3,3,3,3}
Pentisteriruncinated 8-simplex
|

|

|

|
|

|

|

|
| 62
|
              
t1,3,4,5{3,3,3,3,3,3,3}
Bisteriruncicantellated 8-simplex
|

|

|

|
|

|

|

|
| 63
|
              
t2,3,4,5{3,3,3,3,3,3,3}
Triruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 64
|
              
t0,1,2,6{3,3,3,3,3,3,3}
Hexicantitruncated 8-simplex
|

|

|

|

|

|

|
|
| 65
|
              
t0,1,3,6{3,3,3,3,3,3,3}
Hexiruncitruncated 8-simplex
|
|

|

|

|

|

|

|
| 66
|
              
t0,2,3,6{3,3,3,3,3,3,3}
Hexiruncicantellated 8-simplex
|

|

|

|

|

|
|

|
| 67
|
              
t1,2,3,6{3,3,3,3,3,3,3}
Bipenticantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 68
|
              
t0,1,4,6{3,3,3,3,3,3,3}
Hexisteritruncated 8-simplex
|

|

|

|

|

|

|

|
| 69
|
              
t0,2,4,6{3,3,3,3,3,3,3}
Hexistericantellated 8-simplex
|

|

|

|

|

|

|

|
| 70
|
              
t1,2,4,6{3,3,3,3,3,3,3}
Bipentiruncitruncated 8-simplex
|

|

|

|

|
|

|

|
| 71
|
              
t0,3,4,6{3,3,3,3,3,3,3}
Hexisteriruncinated 8-simplex
|

|

|

|

|

|

|

|
| 72
|
              
t1,3,4,6{3,3,3,3,3,3,3}
Bipentiruncicantellated 8-simplex
|

|
|

|

|

|

|

|
| 73
|
              
t0,1,5,6{3,3,3,3,3,3,3}
Hexipentitruncated 8-simplex
|

|

|

|

|

|

|

|
| 74
|
              
t0,2,5,6{3,3,3,3,3,3,3}
Hexipenticantellated 8-simplex
|

|

|

|

|

|

|

|
| 75
|
              
t1,2,5,6{3,3,3,3,3,3,3}
Bipentisteritruncated 8-simplex
|

|

|

|
|

|

|

|
| 76
|
              
t0,3,5,6{3,3,3,3,3,3,3}
Hexipentiruncinated 8-simplex
|

|

|

|

|

|

|

|
| 77
|
              
t0,4,5,6{3,3,3,3,3,3,3}
Hexipentistericated 8-simplex
|
|

|

|

|

|

|

|
| 78
|
              
t0,1,2,7{3,3,3,3,3,3,3}
Hepticantitruncated 8-simplex
|

|

|

|
|

|

|

|
| 79
|
              
t0,1,3,7{3,3,3,3,3,3,3}
Heptiruncitruncated 8-simplex
|

|

|

|

|
|
|

|
| 80
|
              
t0,2,3,7{3,3,3,3,3,3,3}
Heptiruncicantellated 8-simplex
|

|

|
|

|

|

|
|
| 81
|
              
t0,1,4,7{3,3,3,3,3,3,3}
Heptisteritruncated 8-simplex
|

|

|

|

|

|

|

|
| 82
|
              
t0,2,4,7{3,3,3,3,3,3,3}
Heptistericantellated 8-simplex
|

|

|
|

|

|

|

|
| 83
|
              
t0,3,4,7{3,3,3,3,3,3,3}
Heptisteriruncinated 8-simplex
|

|

|

|

|

|

|

|
| 84
|
              
t0,1,5,7{3,3,3,3,3,3,3}
Heptipentitruncated 8-simplex
|

|

|

|

|

|

|
|
| 85
|
              
t0,2,5,7{3,3,3,3,3,3,3}
Heptipenticantellated 8-simplex
|

|

|

|

|

|

|

|
| 86
|
              
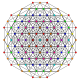
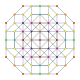
t0,1,6,7{3,3,3,3,3,3,3}
Heptihexitruncated 8-simplex
|

|

|

|
|

|
|

|
| 87
|
              
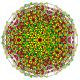
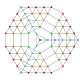
t0,1,2,3,4{3,3,3,3,3,3,3}
Steriruncicantitruncated 8-simplex
|

|

|

|

|
|

|
|
| 88
|
              
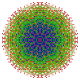
t0,1,2,3,5{3,3,3,3,3,3,3}
Pentiruncicantitruncated 8-simplex
|

|
|

|

|

|

|

|
| 89
|
              
t0,1,2,4,5{3,3,3,3,3,3,3}
Pentistericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 90
|
              
t0,1,3,4,5{3,3,3,3,3,3,3}
Pentisteriruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 91
|
              
t0,2,3,4,5{3,3,3,3,3,3,3}
Pentisteriruncicantellated 8-simplex
|

|

|

|

|

|

|

|
| 92
|
              
t1,2,3,4,5{3,3,3,3,3,3,3}
Bisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 93
|
              
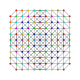
t0,1,2,3,6{3,3,3,3,3,3,3}
Hexiruncicantitruncated 8-simplex
|

|

|

|

|

|
|

|
| 94
|
              
t0,1,2,4,6{3,3,3,3,3,3,3}
Hexistericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 95
|
              
t0,1,3,4,6{3,3,3,3,3,3,3}
Hexisteriruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 96
|
              
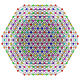
t0,2,3,4,6{3,3,3,3,3,3,3}
Hexisteriruncicantellated 8-simplex
|

|

|

|
|

|

|

|
| 97
|
              
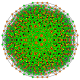
t1,2,3,4,6{3,3,3,3,3,3,3}
Bipentiruncicantitruncated 8-simplex
|

|

|

|

|
|

|

|
| 98
|
              
t0,1,2,5,6{3,3,3,3,3,3,3}
Hexipenticantitruncated 8-simplex
|

|

|

|

|
|

|

|
| 99
|
              
t0,1,3,5,6{3,3,3,3,3,3,3}
Hexipentiruncitruncated 8-simplex
|
|

|

|

|

|

|

|
| 100
|
              
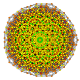
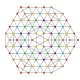
t0,2,3,5,6{3,3,3,3,3,3,3}
Hexipentiruncicantellated 8-simplex
|

|
|

|
|
|

|
|
| 101
|
              
t1,2,3,5,6{3,3,3,3,3,3,3}
Bipentistericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 102
|
              
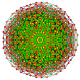
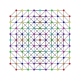
t0,1,4,5,6{3,3,3,3,3,3,3}
Hexipentisteritruncated 8-simplex
|

|

|

|

|
|
|
|
| 103
|
              
t0,2,4,5,6{3,3,3,3,3,3,3}
Hexipentistericantellated 8-simplex
|

|

|

|
|

|

|
|
| 104
|
              
t0,3,4,5,6{3,3,3,3,3,3,3}
Hexipentisteriruncinated 8-simplex
|

|

|

|

|
|

|

|
| 105
|
              
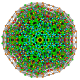
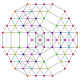
t0,1,2,3,7{3,3,3,3,3,3,3}
Heptiruncicantitruncated 8-simplex
|

|

|

|

|
|

|
|
| 106
|
              
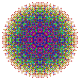
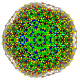
t0,1,2,4,7{3,3,3,3,3,3,3}
Heptistericantitruncated 8-simplex
|

|
|

|

|
|

|

|
| 107
|
              
t0,1,3,4,7{3,3,3,3,3,3,3}
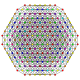
Heptisteriruncitruncated 8-simplex
|

|

|

|
|

|

|

|
| 108
|
              
t0,2,3,4,7{3,3,3,3,3,3,3}
Heptisteriruncicantellated 8-simplex
|

|

|

|

|

|

|

|
| 109
|
              
t0,1,2,5,7{3,3,3,3,3,3,3}
Heptipenticantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 110
|
              
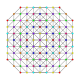
t0,1,3,5,7{3,3,3,3,3,3,3}
Heptipentiruncitruncated 8-simplex
|

|

|
|

|

|
|

|
| 111
|
              
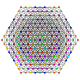
t0,2,3,5,7{3,3,3,3,3,3,3}
Heptipentiruncicantellated 8-simplex
|

|

|

|
|

|

|

|
| 112
|
              
t0,1,4,5,7{3,3,3,3,3,3,3}
Heptipentisteritruncated 8-simplex
|

|

|

|

|

|

|
|
| 113
|
              
t0,1,2,6,7{3,3,3,3,3,3,3}
Heptihexicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 114
|
              
t0,1,3,6,7{3,3,3,3,3,3,3}
Heptihexiruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 115
|
              
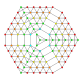
t0,1,2,3,4,5{3,3,3,3,3,3,3}
Pentisteriruncicantitruncated 8-simplex
|

|
|

|

|
|
|
|
| 116
|
              
t0,1,2,3,4,6{3,3,3,3,3,3,3}
Hexisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 117
|
              
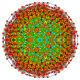
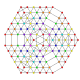
t0,1,2,3,5,6{3,3,3,3,3,3,3}
Hexipentiruncicantitruncated 8-simplex
|

|

|

|

|
|

|
|
| 118
|
              
t0,1,2,4,5,6{3,3,3,3,3,3,3}
Hexipentistericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 119
|
              
t0,1,3,4,5,6{3,3,3,3,3,3,3}
Hexipentisteriruncitruncated 8-simplex
|

|

|

|

|

|

|

|
| 120
|
              
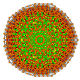
t0,2,3,4,5,6{3,3,3,3,3,3,3}
Hexipentisteriruncicantellated 8-simplex
|

|

|

|

|
|

|

|
| 121
|
              
t1,2,3,4,5,6{3,3,3,3,3,3,3}
Bipentisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 122
|
              
t0,1,2,3,4,7{3,3,3,3,3,3,3}
Heptisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 123
|
              
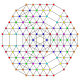
t0,1,2,3,5,7{3,3,3,3,3,3,3}
Heptipentiruncicantitruncated 8-simplex
|

|

|

|

|

|

|
|
| 124
|
              
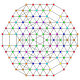
t0,1,2,4,5,7{3,3,3,3,3,3,3}
Heptipentistericantitruncated 8-simplex
|

|

|

|

|

|

|
|
| 125
|
              
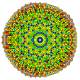
t0,1,3,4,5,7{3,3,3,3,3,3,3}
Heptipentisteriruncitruncated 8-simplex
|

|

|

|

|
|

|

|
| 126
|
              
t0,2,3,4,5,7{3,3,3,3,3,3,3}
Heptipentisteriruncicantellated 8-simplex
|

|

|

|

|

|

|

|
| 127
|
              
t0,1,2,3,6,7{3,3,3,3,3,3,3}
Heptihexiruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 128
|
              
t0,1,2,4,6,7{3,3,3,3,3,3,3}
Heptihexistericantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 129
|
              
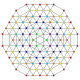
t0,1,3,4,6,7{3,3,3,3,3,3,3}
Heptihexisteriruncitruncated 8-simplex
|

|

|

|

|

|

|
|
| 130
|
              
t0,1,2,5,6,7{3,3,3,3,3,3,3}
Heptihexipenticantitruncated 8-simplex
|

|

|

|

|

|
|
|
| 131
|
              
t0,1,2,3,4,5,6{3,3,3,3,3,3,3}
Hexipentisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|

|
| 132
|
              
t0,1,2,3,4,5,7{3,3,3,3,3,3,3}
Heptipentisteriruncicantitruncated 8-simplex
|

|

|

|

|

|
|

|
| 133
|
              
t0,1,2,3,4,6,7{3,3,3,3,3,3,3}
Heptihexisteriruncicantitruncated 8-simplex
|

|

|

|

|

|

|
|
| 134
|
              
t0,1,2,3,5,6,7{3,3,3,3,3,3,3}
Heptihexipentiruncicantitruncated 8-simplex
|

|

|

|
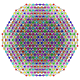
|

|

|

|
| 135
|
              
t0,1,2,3,4,5,6,7{3,3,3,3,3,3,3}
Omnitruncated 8-simplex
|

|

|

|

|

|

|
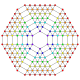
|