| Revision as of 09:29, 10 July 2014 editTarnishedPath (talk | contribs)Extended confirmed users, IP block exemptions, New page reviewers, Pending changes reviewers17,887 editsm Reverted 1 edit by 190.161.186.94 (talk) to last revision by AlanS. (TW)← Previous edit | Latest revision as of 16:35, 2 November 2024 edit undoCitation bot (talk | contribs)Bots5,457,356 edits Altered doi-broken-date. | Use this bot. Report bugs. | #UCB_CommandLine | ||
| (609 intermediate revisions by more than 100 users not shown) | |||
| Line 1: | Line 1: | ||
| {{short description|Surface waves generated by wind on open water}} | |||
| {{redirect3|Ocean wave|For the film, see ]}} | |||
| {{redirect|Ocean wave||Ocean Wave (disambiguation)}} | |||
| ]]] | |||
| ] along the coast of ], ]]] | |||
| In ], a '''wind wave''', or '''wind-generated water wave''', is a ] that occurs on the ] of ] as a result of the ] blowing over the water's surface. The contact distance in the ] is known as the '']''. Waves in the oceans can travel thousands of kilometers before reaching land. Wind waves on Earth range in size from small ] to waves over {{convert|100|ft|m|abbr=on|order=flip}} high, being limited by wind speed, duration, fetch, and water depth.<ref>{{cite book | first=H. L. | last=Tolman | title=CBMS Conference Proceedings on Water Waves: Theory and Experiment | location=Howard University, US, 13–18 May 2008 | publisher=World Scientific Publications | url=http://polar.ncep.noaa.gov/mmab/papers/tn270/Howard_08.pdf | editor-first=M.F. | editor-last=Mahmood | date=23 June 2010 | isbn=978-981-4304-23-8}}</ref> | |||
| ] storm waves as seen from the ] ] ''Noble Star'', Winter 1989.]] | |||
| ].]] | |||
| ] | |||
| [[File:Global_Wave_Height_Speed.jpg|thumb| The image shows the global distribution of wind speed and wave height as observed by NASA's TOPEX/Poseidon's dual-frequency radar altimeter from October 3 to October 12, 1992. Simultaneous observations of wind speed and wave height are helping scientists to predict ocean waves . Wind speed is determined by the strength of the radar signal after it has bounced off the ocean surface and returned to the satellite. A calm sea serves as a good reflector and returns a strong signal; a rough sea tends to scatter the signals and returns a weak pulse. Wave height is determined by the shape of the return radar pulse. | |||
| A calm sea with low waves returns a condensed pulse whereas a rough sea with high waves returns a stretched pulse. Comparing the two images above shows a high degree of correlation between wind speed and wave height. The strongest winds (over 54 kilometers per hour or 33.6 miles per hour) and highest waves are found in the Southern Ocean. The weakest winds -- shown as areas of magenta and dark blue -- are generally found in the tropical Oceans.]] | |||
| In ], '''wind waves''' or, more precisely, '''wind-generated waves''' are ]s that occur on the ] of ]s, ]s, ]s, ]s, and ]s or even on small ]s and ]s. They usually result from the ] blowing over a vast enough stretch of fluid surface. Waves in the oceans can travel thousands of miles before reaching land. Wind waves range in size from small ] to huge waves over 30 m high.<ref>{{citation | first=H.L. | last=Tolman | contribution=Practical wind wave modeling | title=CBMS Conference Proceedings on Water Waves: Theory and Experiment | location=Howard University, US, 13–18 May 2008 | publisher=World Scientific Publiations | url=http://polar.ncep.noaa.gov/mmab/papers/tn270/Howard_08.pdf | editor-first=M.F. | editor-last=Mahmood | publication-date=23 June 2010 | isbn=978-981-4304-23-8}}</ref> | |||
| When directly generated and affected by local |
When directly generated and affected by local wind, a wind wave system is called a '''wind sea'''. Wind waves will travel in a ] after being generated – curving slightly left in the southern hemisphere and slightly right in the northern hemisphere. After moving out of the area of fetch and no longer being affected by the local wind, wind waves are called '']'' and can travel thousands of kilometers. A noteworthy example of this is waves generated south of Tasmania during heavy winds that will travel across the Pacific to southern California, producing desirable surfing conditions.<ref>Holthuijsen (2007), page 5.{{quote needed|date=May 2024}}</ref> Wind waves in the ocean are also called '''ocean surface waves''' and are mainly '']'', where ] is the main equilibrium force. | ||
| Wind waves have a certain amount of ]: subsequent waves differ in height, duration, and shape with limited predictability. They can be described as a ], in combination with the physics governing their generation, growth, propagation and |
Wind waves have a certain amount of ]: subsequent waves differ in height, duration, and shape with limited predictability. They can be described as a ], in combination with the physics governing their generation, growth, propagation, and decay – as well as governing the interdependence between flow quantities such as the ] movements, ], and water ]. The key ]s of wind waves (both seas and swells) in evolving ]s can be predicted with ]s. | ||
| Although waves are usually considered in the water seas of Earth, the hydrocarbon seas of ] may also have wind-driven waves.<ref>{{cite journal |last1=Lorenz |first1=R. D. |last2=Hayes |first2= A. G. |year=2012 |title=The Growth of Wind-Waves in Titan's Hydrocarbon Seas |journal=Icarus |volume=219 |issue=1 |pages=468–475|bibcode=2012Icar..219..468L |doi=10.1016/j.icarus.2012.03.002 }}</ref><ref>{{Cite journal|last1=Barnes|first1=Jason W.|last2=Sotin|first2=Christophe|last3=Soderblom|first3=Jason M.|last4=Brown|first4=Robert H.|last5=Hayes|first5=Alexander G.|last6=Donelan|first6=Mark|last7=Rodriguez|first7=Sebastien|last8=Mouélic|first8=Stéphane Le|last9=Baines|first9=Kevin H.|last10=McCord|first10=Thomas B.|date=2014-08-21|title=Cassini/VIMS observes rough surfaces on Titan's Punga Mare in specular reflection|journal=Planetary Science|volume=3|issue=1|pages=3|doi=10.1186/s13535-014-0003-4|issn=2191-2521|pmc=4959132|pmid=27512619|bibcode=2014PlSci...3....3B |doi-access=free }}</ref><ref>{{Cite journal|last1=Heslar|first1=Michael F.|last2=Barnes|first2=Jason W.|last3=Soderblom|first3=Jason M.|last4=Seignovert|first4=Benoît|last5=Dhingra|first5=Rajani D.|last6=Sotin|first6=Christophe|date=2020-08-14|title=Tidal Currents Detected in Kraken Mare Straits from Cassini VIMS Sun Glitter Observations|journal=The Planetary Science Journal|language=en|volume=1|issue=2|pages=35|doi=10.3847/PSJ/aba191|arxiv=2007.00804 |bibcode=2020PSJ.....1...35H |s2cid=220301577 |issn=2632-3338 |doi-access=free }}</ref> Waves in bodies of water may also be generated by other causes, both at the surface and underwater (such as ], ]s, ]s, ]s, ]s, ]s, and ]s). | |||
| ]s are a specific type of wave not caused by wind but by geological effects. In deep water, tsunamis are not visible because they are small in height and very long in ]. They may grow to devastating proportions at the coast due to reduced water depth. | |||
| ==Formation== | |||
| Although waves are usually considered in the water seas of Earth, the hydrocarbon seas of ] may also have wind-driven waves. <ref> Lorenz, R. D. and A. G. Hayes, The Growth of Wind-Waves in Titan's Hydrocarbon Seas, Icarus, 219, 468–475, 2012 </ref> | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] ship ''Delaware II'' in bad weather on ]]] | |||
| The great majority of large breakers seen at a beach result from distant winds. Five factors influence the formation of the flow structures in wind waves:<ref>{{cite book |title=Wind generated ocean waves |first=I. R. |last=Young |publisher=Elsevier |year=1999 |isbn=978-0-08-043317-2 |page=83}}</ref> | |||
| ==Wave formation== | |||
| # ] or strength relative to wave speed – the wind must be moving faster than the wave crest for energy transfer to the wave. | |||
| ] ship ''Delaware II'' in bad weather on ].]] | |||
| # The uninterrupted distance of open water over which the wind blows without significant change in direction (called the '']'') | |||
| The great majority of large breakers one observes on a beach result from distant winds. Five factors influence the formation of wind waves:<ref>{{cite book | title=Wind generated ocean waves | first=I. R. | last=Young | publisher=Elsevier | year=1999 | isbn=0-08-043317-0 }} p. 83.</ref> | |||
| # Width of the area affected by fetch (at a right angle to the distance) | |||
| * ] or strength relative to wave speed- the wind must be moving faster than the wave crest for energy transfer | |||
| # Wind duration – the time for which the wind has blown over the water. | |||
| * The uninterrupted distance of open water over which the wind blows without significant change in direction (called the '']'') | |||
| # Water depth | |||
| * Width of area affected by fetch | |||
| All of these factors work together to determine the size of the water waves and the structure of the flow within them. | |||
| * Wind ]- the time over which the wind has blown over a given area | |||
| * Water depth | |||
| The main dimensions associated with ] are: | |||
| All of these factors work together to determine the size of wind waves: | |||
| * ] (vertical distance from trough to ]) | |||
| * ] (from |
* ] (distance from crest to crest in the direction of propagation) | ||
| * ] (from crest to crest) | |||
| * ] (time interval between arrival of consecutive crests at a stationary point) | * ] (time interval between arrival of consecutive crests at a stationary point) | ||
| * |
* Wave direction or ] (predominantly driven by ]) | ||
| ]A fully developed sea has the maximum wave size theoretically possible for a wind of a specific strength, duration, and fetch. Further exposure to that specific wind could only cause a loss of energy due to the breaking of wave tops and formation of "whitecaps". Waves in a given area typically have a range of heights. For weather reporting and for scientific analysis of wind wave statistics, their characteristic height over a period of time is usually expressed as '']''. This figure represents an ] height of the highest one-third of the waves in a given time period (usually chosen somewhere in the range from 20 minutes to twelve hours), or in a specific wave or storm system. The significant wave height is also the value a "trained observer" (e.g. from a ship's crew) would estimate from visual observation of a sea state. Given the variability of wave height, the largest individual waves are likely to be somewhat less than twice the reported significant wave height for a particular day or storm.<ref>{{Cite book | publisher = Springer | isbn = 978-3-540-25316-7 | last1 = Weisse | first1 = Ralf | first2 = Hans | last2 = von Storch | title = Marine climate change: Ocean waves, storms and surges in the perspective of climate change | year = 2008 | page = 51 }}</ref> | |||
| ] | |||
| '''• Sources of wind wave generation:''' Sea water wave is generated by many kinds of disturbances such as Seismic events, gravity, and crossing wind. The generation of wind wave is initiated by the disturbances of cross wind field on the surface of the sea water. Two major Mechanisms of surface wave formation by winds (a.k.a.‘The Miles-Phillips Mechanism’) and other sources (ex. earthquakes) of wave formation can explain the generation of wind waves. | |||
| However, if one set a flat water surface (Beaufort Point,0) and abrupt cross wind flows on the surface of the water,then the generation of surface wind waves can be explained by following two mechanisms which initiated by normal pressure fluctuations of turbulent winds and parallel wind shear flows. | |||
| '''• The mechanism of the surface wave generation by winds''' | |||
| ] | |||
| 1) Starts from “Fluctuations of wind” (O.M.Phillips) : the wind wave formation on water surface by wind is started by a random distribution of normal pressure acting on the water from the wind. By the mechanism developed by O.M. Phillips (in 1957), the water surface is initially at rest and the generation of wave is | |||
| initiated by adding turbulent wind flows and then, by the fluctuations of the wind, normal pressure acting on the water surface. This pressure fluctuation arise normal and tangential stresses to the surface water, and generates wave behavior on the water surface. | |||
| {''Assumptions'' | |||
| 1. water originally at rest | |||
| 2. water is inviscid | |||
| 3. Water is irrotational | |||
| 4. Random distribution of normal pressure to the water surface from the turbulent wind | |||
| 5. Correlations between air and Water motions are neglected}<ref name="Phillips, O. M. 1957">Phillips, O. M. (1957), "On the generation of waves by turbulent wind", Journal of Fluid Mechanics 2 (5): 417–445, Bibcode:1957JFM.....2..417P, doi:10.1017/S0022112057000233</ref> | |||
| A fully developed sea has the maximum wave size theoretically possible for a wind of specific strength, duration, and fetch. Further exposure to that specific wind could only cause a dissipation of energy due to the breaking of wave tops and formation of "whitecaps". Waves in a given area typically have a range of heights. For weather reporting and for scientific analysis of wind wave statistics, their characteristic height over a period of time is usually expressed as '']''. This figure represents an ] height of the highest one-third of the waves in a given time period (usually chosen somewhere in the range from 20 minutes to twelve hours), or in a specific wave or storm system. The significant wave height is also the value a "trained observer" (e.g. from a ship's crew) would estimate from visual observation of a sea state. Given the variability of wave height, the largest individual waves are likely to be somewhat less than twice the reported significant wave height for a particular day or storm.<ref>{{Cite book | publisher = Springer | isbn = 978-3-540-25316-7 | last1 = Weisse | first1 = Ralf | first2 = Hans | last2 = von Storch | title = Marine climate change: Ocean waves, storms and surges in the perspective of climate change | year = 2008 | page = 51 }}</ref> | |||
| 2) starts from “wind shear forces” on the water surface (J.W.Miles, applied to mainly 2D deep water gravity waves) ; John W. Miles suggested a surface wave generation mechanism which is initiated by turbulent wind shear flows Ua(y), based on the inviscid Orr-Sommerfeld equation in 1957. He found the energy transfer from wind to water surface as a wave speed, c is proportional to the curvature of the velocity profile of wind Ua’’(y) at point where the mean wind speed is equal to the wave speed (Ua=c, where, Ua is the Mean turbulent wind speed). Since the wind profile Ua(y) is logarithmic to the water surface, the curvature Ua’’(y) have negative sign at the point of Ua=c. This relations show the wind flow transferring its kinetic energy to the water surface at their interface, and arises wave speed, c. | |||
| Wave formation on an initially flat water surface by wind is started by a random distribution of normal pressure of turbulent wind flow over the water. This pressure fluctuation produces normal and tangential stresses in the surface water, which generates waves. It is usually assumed for the purpose of theoretical analysis that:<ref name="Phillips, O. M. 1957">{{cite journal |last1=Phillips |first1=O. M. |author-link=Owen Martin Phillips |title=On the generation of waves by turbulent wind |journal=Journal of Fluid Mechanics |date=2006 |volume=2 |issue=5 |pages=417 |doi=10.1017/S0022112057000233 |doi-broken-date=1 November 2024 |bibcode=1957JFM.....2..417P|s2cid=116675962 }}</ref> | |||
| the growth-rate can be determined by the curvature of the winds ((d^2 Ua)/(dz^2 )) at the steering height (Ua (z=z_h)=c) for a given wind speed Ua | |||
| # The water is originally at rest. | |||
| {''Assumptions''; | |||
| # The water is not viscous. | |||
| 1. 2D parallel shear flow, Ua(y) | |||
| # The water is ]. | |||
| 2. incompressible, inviscid water / wind | |||
| # There is a random distribution of normal pressure to the water surface from the turbulent wind. | |||
| 3. irrotational water | |||
| # Correlations between air and water motions are neglected. | |||
| 4. slope of the displacement of surface is small}<ref>Miles, J. W. (1957), "On the generation of surface waves by shear flows", Journal of Fluid Mechanics 3 (2): 185–204, Bibcode:1957JFM.....3..185M, doi:10.1017/S0022112057000567</ref> | |||
| The second mechanism involves wind shear forces on the water surface. ] suggested a surface wave generation mechanism that is initiated by turbulent wind shear flows based on the inviscid ] in 1957. He found the energy transfer from the wind to the water surface is proportional to the curvature of the velocity profile of the wind at the point where the mean wind speed is equal to the wave speed. Since the wind speed profile is logarithmic to the water surface, the curvature has a negative sign at this point. This relation shows the wind flow transferring its kinetic energy to the water surface at their interface. | |||
| '''• Generally''', these wave formation mechanisms occur together on the ocean surface and arise wind waves and grows up to the fully developed waves. | |||
| Assumptions: | |||
| For example,<ref></ref> | |||
| # two-dimensional parallel shear flow | |||
| # incompressible, inviscid water and wind | |||
| # irrotational water | |||
| # slope of the displacement of the water surface is small<ref>{{cite journal |last1=Miles |first1=John W. |author-link=John W. Miles |title=On the generation of surface waves by shear flows |journal=Journal of Fluid Mechanics |date=2006 |volume=3 |issue=2 |pages=185 |doi=10.1017/S0022112057000567 |doi-broken-date=1 November 2024 |bibcode=1957JFM.....3..185M|s2cid=119795395 }}</ref> | |||
| Generally, these wave formation mechanisms occur together on the water surface and eventually produce fully developed waves. | |||
| If we suppose a very flat sea surface (Beaufort number, 0), and sudden wind flow blows steadily across the sea surface, physical wave generation process will be like; | |||
| For example,<ref>{{Cite web |url=http://oceanworld.tamu.edu/resources/ocng_textbook/chapter16/chapter16_04.htm |title=Chapter 16, ''Ocean Waves'' |access-date=2013-11-12 |archive-date=2016-05-11 |archive-url=https://web.archive.org/web/20160511210532/http://oceanworld.tamu.edu/resources/ocng_textbook/chapter16/chapter16_04.htm |url-status=dead }}</ref> if we assume a flat sea surface (Beaufort state 0), and a sudden wind flow blows steadily across the sea surface, the physical wave generation process follows the sequence: | |||
| 1. Turbulent wind flows form random pressure fluctuations at the sea surface. Small waves with a few centimeters order of wavelengths is generated by the pressure fluctuations. (The Phillips mechanism<ref name="Phillips, O. M. 1957"/>) | |||
| # Turbulent wind forms random pressure fluctuations at the sea surface. Ripples with wavelengths in the order of a few centimeters are generated by the pressure fluctuations. (The ] mechanism<ref name="Phillips, O. M. 1957"/>) | |||
| # The winds keep acting on the initially rippled sea surface causing the waves to become larger. As the waves grow, the pressure differences get larger causing the growth rate to increase. Finally, the shear instability expedites the wave growth exponentially. (The Miles mechanism<ref name="Phillips, O. M. 1957"/>) | |||
| # The interactions between the waves on the surface generate longer waves<ref>{{cite journal |last=Hasselmann |first=K. |display-authors=etal |year=1973 |title=Measurements of wind-wave growth and swell decay during the Joint North Sea Wave Project (JONSWAP) |url=https://www.researchgate.net/publication/256197895 |journal=Ergnzungsheft zur Deutschen Hydrographischen Zeitschrift Reihe A |volume=8 |issue=12 |page=95 |hdl=10013/epic.20654}}</ref> and the interaction will transfer wave energy from the shorter waves generated by the Miles mechanism to the waves which have slightly lower frequencies than the frequency at the peak wave magnitudes, then finally the waves will be faster than the crosswind speed (Pierson & Moskowitz<ref>{{cite journal|last1=Pierson|first1=Willard J.|last2=Moskowitz|first2=Lionel|title=A proposed spectral form for fully developed wind seas based on the similarity theory of S. A. Kitaigorodskii|journal=Journal of Geophysical Research|date=15 December 1964|volume=69|issue=24|pages=5181–5190|doi=10.1029/JZ069i024p05181|bibcode=1964JGR....69.5181P}}</ref>). | |||
| {| class="collapsible wikitable" border="1" style="font-size:92%" | |||
| 3. The interactions between the waves on the surface generate longer waves (Hasselmann et al., 1973<ref>Hasselmann K., T.P. Barnett, E. Bouws, H. Carlson, D.E. Cartwright, K. Enke, J.A. Ewing, H. Gienapp, D.E. Hasselmann, P. Kruseman, A. Meerburg, P. Mller, D.J. Olbers, K. Richter, W. Sell, and H. Walden. Measurements of wind-wave growth and swell decay during the Joint North Sea Wave Project (JONSWAP)' Ergnzungsheft zur Deutschen Hydrographischen Zeitschrift Reihe, A(8) (Nr. 12), p.95, 1973.</ref>) and the interaction will transfer wave energy from the shorter waves generated by the Miles mechanism to the waves have slightly lower frequencies than the frequency at the peak wave magnitudes, then finally the waves will be faster than the cross wind speed (Pierson & Moskowitz<ref>Pierson, Willard J., Jr. and Moskowitz, Lionel A. Proposed Spectral Form for Fully Developed Wind Seas Based on the Similarity Theory of S. A. Kitaigorodskii, Journal of Geophysical Research, Vol. 69, p.5181-5190, 1964.</ref>). | |||
| ! colspan=6 style="background: #ccf;" | Conditions necessary for a fully developed sea at given wind speeds, and the parameters of the resulting waves | |||
| {| class="collapsible wikitable" border="1" style="font-size:92%; | |||
| ! colspan=6 style="background: #ccf;" | Conditions Necessary for a Fully Developed Sea at Given Wind Speeds, and the Parameters of the Resulting Waves | |||
| |- | |- | ||
| ! colspan=3 style | Wind |
! colspan=3 style | Wind conditions | ||
| ! colspan= 3 style | Wave |
! colspan= 3 style | Wave size | ||
| |- | |- | ||
| ! Wind |
! Wind speed in one direction !! Fetch !! Wind duration !! Average height !! Average wavelength !! Average period and speed | ||
| |- | |- | ||
| | 19 |
| {{convert|19|km/h|mph|abbr=on}}|| {{convert|19|km|mi|abbr=on}} || 2 hr || {{convert|0.27|m|ft|abbr=on}}|| {{convert|8.5|m|ft|abbr=on}} || 3.0 sec, 10.2 km/h (9.3 ft/sec) | ||
| |- | |- | ||
| | 37 |
| {{convert|37|km/h|mph|abbr=on}}|| {{convert|139|km|mi|abbr=on}} || 10 hr || {{convert|1.5|m|ft|abbr=on}}|| {{convert|33.8|m|ft|abbr=on}} || 5.7 sec, 21.4 km/h (19.5 ft/sec) | ||
| |- | |- | ||
| | 56 |
| {{convert|56|km/h|mph|abbr=on}}|| {{convert|518|km|mi|abbr=on}} || 23 hr || {{convert|4.1|m|ft|abbr=on}}|| {{convert|76.5|m|ft|abbr=on}} || 8.6 sec, 32.0 km/h (29.2 ft/sec) | ||
| |- | |- | ||
| | 74 |
| {{convert|74|km/h|mph|abbr=on}}|| {{convert|1,313|km|mi|abbr=on}} || 42 hr || {{convert|8.5|m|ft|abbr=on}}|| {{convert|136|m|ft|abbr=on}} || 11.4 sec, 42.9 km/h (39.1 ft/sec) | ||
| |- | |- | ||
| | 92 |
| {{convert|92|km/h|mph|abbr=on}}|| {{convert|2,627|km|mi|abbr=on}} || 69 hr || {{convert|14.8|m|ft|abbr=on}}|| {{convert|212.2|m|ft|abbr=on}} || 14.3 sec, 53.4 km/h (48.7 ft/sec) | ||
| |- | |||
| | colspan=6 | NOTE: Most of the wave speeds calculated from the wave length divided by the period are proportional to the square root of the wave length. Thus, except for the shortest wave length, the waves follow the deep water theory. The 28 ft long wave must be either in shallow water or intermediate depth. | |||
| |} | |} | ||
| ==Types== | |||
| ((NOTE: Most of the wave speeds calculated from the wave length divided by the period are proportional to sqrt (length). Thus, except for the shortest wave length, the waves follow the deep water theory described in the next section. The 28 ft long wave must be either in shallow water or between deep and shallow.)) | |||
| ], west coast of Portugal]] | |||
| ==Types of wind waves== | |||
| ] of ocean waves according to wave ].<ref>{{Citation | last=Munk |first=Walter H. |authorlink=Walter Munk |year=1950 |contribution=Origin and generation of waves |title=Proceedings 1st International Conference on Coastal Engineering |location=Long Beach, California |publisher=] |pages=1–4 |url=http://journals.tdl.org/ICCE/article/view/904 }}</ref> ]] | |||
| ] | |||
| Three different types of wind waves develop over time: | Three different types of wind waves develop over time: | ||
| * ]s, or ripples | * ]s, or ripples, dominated by surface tension effects. | ||
| * ], dominated by gravitational and inertial forces. | |||
| * Seas | |||
| ** Seas, raised locally by the wind. | |||
| * ] | |||
| * ], which have traveled away from where they were raised by the wind, and have to a greater or lesser extent dispersed. | |||
| Ripples appear on smooth water when the wind blows, but will die quickly if the wind stops. The restoring force that allows them to propagate is ]. |
Ripples appear on smooth water when the wind blows, but will die quickly if the wind stops. The restoring force that allows them to propagate is ]. Sea waves are larger-scale, often irregular motions that form under sustained winds. These waves tend to last much longer, even after the wind has died, and the restoring force that allows them to propagate is gravity. As waves propagate away from their area of origin, they naturally separate into groups of common direction and wavelength. The sets of waves formed in this manner are known as swells. The ] is {{Convert|19,800|km|abbr=on}} from ] to the coast of ] and, based on an average wavelength of {{Convert|76.5|m|abbr=on}}, would have ~258,824 swells over that width. | ||
| It is sometimes alleged that out of a set of waves, the seventh wave in a set is always the largest; while this isn't the case, the waves in the middle of a given set tend to be larger than those before and after them.<ref>{{Cite web |title=Know the risks: Waves |url=https://rnli.org/safety/know-the-risks/waves |access-date=31 August 2024 |website=]}}</ref> | |||
| Individual "]s" (also called "freak waves", "monster waves", "killer waves", and "king waves") much higher than the other waves in the ] can occur. In the case of the ], its {{convert|25|m|ft|abbr=on}} height was 2.2 times the ]. Such waves are distinct from ]s, caused by the ] and ]'s ], ]s that are caused by underwater ]s or ]s, and waves generated by ]s or the fall of ]s—all having far longer ]s than wind waves. | Individual "]s" (also called "freak waves", "monster waves", "killer waves", and "king waves") much higher than the other waves in the ] can occur. In the case of the ], its {{convert|25|m|ft|abbr=on}} height was 2.2 times the ]. Such waves are distinct from ]s, caused by the ] and ]'s ], ]s that are caused by underwater ]s or ]s, and waves generated by ]s or the fall of ]s—all having far longer ]s than wind waves. | ||
| The largest ever recorded wind waves are not rogue waves, but standard waves in extreme sea states. For example, {{convert|29.1|m|ft|abbr=on}} high waves were recorded on the ] in a sea with {{convert|18.5|m|ft|abbr=on}} significant wave height, so the highest wave was only 1.6 times the significant wave height.<ref>{{cite journal | first1=Naomi P. | last1=Holliday | first2=Margaret J. | last2=Yelland | first3=Robin | last3=Pascal | first4=Val R. | last4=Swail | first5=Peter K. | last5=Taylor | first6=Colin R. | last6=Griffiths | first7=Elizabeth | last7=Kent | title=Were extreme waves in the Rockall Trough the largest ever recorded? | journal=] | volume=33 | year=2006 | issue=L05613 | doi=10.1029/2005GL025238 |bibcode = 2006GeoRL..33.5613H| doi-access=free }}</ref> | |||
| The biggest recorded by a buoy (as of 2011) was {{convert|32.3|m|ft|abbr=on}} high during the ] near Taiwan.<ref>{{ |
The biggest recorded by a buoy (as of 2011) was {{convert|32.3|m|ft|abbr=on}} high during the ] near Taiwan.<ref>{{cite journal |author= P. C. Liu |author2= H. S. Chen |author3= D.-J. Doong |author4= C. C. Kao |author5= Y.-J. G. Hsu |title= Monstrous ocean waves during typhoon Krosa |journal= Annales Geophysicae |volume= 26 |issue= 6 |date= 11 June 2008 |pages= 1327–1329 |doi= 10.5194/angeo-26-1327-2008|bibcode = 2008AnGeo..26.1327L|doi-access= free }}</ref> | ||
| ==Spectrum== | |||
| ] of ocean waves according to wave ]<ref>{{Cite journal | last=Munk |first=Walter H. |author-link=Walter Munk |year=1950 |title=Proceedings 1st International Conference on Coastal Engineering |journal=Coastal Engineering Proceedings |issue=1 |location=Long Beach, California |publisher=] |pages=1–4 |doi=10.9753/icce.v1.1 |url=http://journals.tdl.org/ICCE/article/view/904 |doi-access=free }}</ref> ]] | |||
| Ocean waves can be classified based on: the disturbing force |
Ocean waves can be classified based on: the disturbing force that creates them; the extent to which the disturbing force continues to influence them after formation; the extent to which the restoring force weakens or flattens them; and their wavelength or period. Seismic sea waves have a period of about 20 minutes, and speeds of {{convert|760|km/h|mph|abbr=on}}. Wind waves (deep-water waves) have a period up to about 20 seconds. | ||
| {| class="wikitable" | {| class="wikitable" | ||
| |+<ref name="Garrison">{{cite book|author=Tom Garrison|title =Oceanography: An Invitation to Marine Science |
|+<ref name="Garrison">{{cite book|author=Tom Garrison|title =Oceanography: An Invitation to Marine Science|publisher =Yolanda Cossio|year =2009|isbn =978-0495391937|edition =7th}}</ref> | ||
| |- | |- | ||
| ! Wave type!! Typical wavelength !! Disturbing force !! Restoring force | ! Wave type!! Typical wavelength !! Disturbing force !! Restoring force | ||
| |- | |- | ||
| | |
| Capillary wave|| < 2 cm || Wind || Surface tension | ||
| |- | |- | ||
| | |
| Wind wave || {{convert|60|–|150|m|ft|abbr=on}} || Wind over ocean || Gravity | ||
| |- | |- | ||
| | |
| ] || Large, variable; a function of basin size || Change in atmospheric pressure, storm surge || Gravity | ||
| |- | |- | ||
| | |
| Seismic sea wave (tsunami) || {{convert|200|km|mi|abbr=on}} || Faulting of sea floor, volcanic eruption, landslide || Gravity | ||
| |- | |- | ||
| | |
| Tide || Half the circumference of Earth || Gravitational attraction, rotation of Earth || Gravity | ||
| |} | |} | ||
| The speed of all ocean waves is controlled by gravity, wavelength, and water depth. Most characteristics of ocean waves depend on the relationship between their wavelength and water depth. Wavelength determines the size of the orbits of water molecules within a wave, but water depth determines the shape of the orbits. The paths of water molecules in a wind wave are circular only when the wave is traveling in deep water. A wave cannot "feel" the bottom when it moves through water deeper than half its wavelength because too little wave energy is contained in the |
The speed of all ocean waves is controlled by gravity, wavelength, and water depth. Most characteristics of ocean waves depend on the relationship between their wavelength and water depth. Wavelength determines the size of the orbits of water molecules within a wave, but water depth determines the shape of the orbits. The paths of water molecules in a wind wave are circular only when the wave is traveling in deep water. A wave cannot "feel" the bottom when it moves through water deeper than half its wavelength because too little wave energy is contained in the water movement below that depth. Waves moving through water deeper than half their wavelength are known as deep-water waves. On the other hand, the orbits of water molecules in waves moving through shallow water are flattened by the proximity of the sea bottom surface. Waves in water shallower than 1/20 their original wavelength are known as shallow-water waves. Transitional waves travel through water deeper than 1/20 their original wavelength but shallower than half their original wavelength. | ||
| In general, the longer the wavelength, the faster the wave energy will move through the water. |
In general, the longer the wavelength, the faster the wave energy will move through the water. The relationship between the wavelength, period and velocity of any wave is: | ||
| :::<math> C = {L}/{T} </math> | :::<math> C = {L}/{T} </math> | ||
| where C is speed (celerity), L is wavelength, and T is |
where C is speed (celerity), L is the wavelength, and T is the period (in seconds). Thus the speed of the wave derives from the functional dependence <math> L(T) </math> of the wavelength on the period (the ]). | ||
| The speed of a deep-water wave may also be approximated by: | The speed of a deep-water wave may also be approximated by: | ||
| Line 139: | Line 130: | ||
| :::<math> C = \sqrt{{gL}/{2\pi}} </math> | :::<math> C = \sqrt{{gL}/{2\pi}} </math> | ||
| where g is the acceleration due to gravity, 9.8 |
where g is the acceleration due to gravity, {{convert|9.8|m|ft|abbr=off|sp=us}} per second squared. Because g and π (3.14) are constants, the equation can be reduced to: | ||
| :::<math> C = 1.251\sqrt{L} </math> | :::<math> C = 1.251\sqrt{L} </math> | ||
| when C is measured in meters per second and L in meters. |
when C is measured in meters per second and L in meters. In both formulas the wave speed is proportional to the square root of the wavelength. | ||
| The speed of shallow-water waves is described by a different equation that may be written as: | The speed of shallow-water waves is described by a different equation that may be written as: | ||
| Line 149: | Line 140: | ||
| :::<math> C = \sqrt{gd} = 3.1\sqrt{d} </math> | :::<math> C = \sqrt{gd} = 3.1\sqrt{d} </math> | ||
| where C is speed (in meters per second), g is the acceleration due to gravity, and d is the depth of the water (in meters). The period of a wave remains unchanged regardless of the depth of water through which it is moving. As deep-water waves enter the shallows and feel the bottom, however, their speed is reduced and their crests "bunch up |
where C is speed (in meters per second), g is the acceleration due to gravity, and d is the depth of the water (in meters). The period of a wave remains unchanged regardless of the depth of water through which it is moving. As deep-water waves enter the shallows and feel the bottom, however, their speed is reduced, and their crests "bunch up", so their wavelength shortens. | ||
| {{clear}} | {{clear}} | ||
| ===Spectral models=== | |||
| ==Wave shoaling and refraction== | |||
| ] can be described by the '''sea wave spectrum''' or just '''wave spectrum''' <math>S(\omega, \Theta)</math>. It is composed of a '''wave height spectrum''' (WHS) <math>S(\omega)</math> and a '''wave direction spectrum''' (WDS) <math>f(\Theta)</math>. Many interesting properties about the sea state can be found from the wave spectra. | |||
| {{see also|wave shoaling|refraction}} | |||
| As waves travel from deep to shallow water, their shape alters (wave height increases, speed decreases, and length decreases as wave orbits become asymmetrical). This process is called ]. | |||
| WHS describes the ] of ] ] ("power") versus ], with ] <math>\{S(\omega)\} = \{{\text{length}}^2\cdot\text{time}\}</math>. | |||
| Wave ] is the process by which wave ]s realign themselves as a result of decreasing water depths. Varying depths along a wave crest cause the crest to travel at different ]s, with those parts of the wave in deeper water moving faster than those in ]. This process continues until the crests become (nearly) parallel to the depth contours. ]—lines ] to wave crests between which a fixed amount of energy ] is contained—converge on local shallows and shoals. Therefore, the ] between rays is concentrated as they converge, with a resulting increase in wave height. | |||
| The relationship between the spectrum <math>S(\omega_j)</math> and the wave amplitude <math>A_j</math> for a wave component <math>j</math> is: | |||
| : <math>\frac{1}{2} A_j^2 = S(\omega_j)\, \Delta \omega</math>{{citation needed|date=February 2021}}{{Clarify|define the variables. What do the symbols stand for?|date=May 2023}} | |||
| Some WHS models are listed below. | |||
| * The International Towing Tank Conference (ITTC) <ref>{{citation | title=International Towing Tank Conference (ITTC) | url=http://ittc.sname.org/ | access-date=11 November 2010 }}</ref> recommended spectrum model for fully developed sea (ISSC<ref>International Ship and Offshore Structures Congress</ref> spectrum/modified ]):<ref>{{citation | doi=10.1029/JZ069i024p05181 | first1=W. J. | last1=Pierson | first2=L. | last2=Moscowitz | title=A proposed spectral form for fully developed wind seas based on the similarity theory of S A Kitaigorodskii | journal=Journal of Geophysical Research | volume=69 | issue=24 | pages=5181–5190 | year=1964 | bibcode=1964JGR....69.5181P}}</ref> | |||
| :: <math> | |||
| \frac{S(\omega)}{H_{1/3}^2 T_1} = \frac{0.11}{2\pi} \left(\frac{\omega T_1}{2\pi}\right)^{-5} \mathrm{exp} \left | |||
| </math> | |||
| * ITTC recommended spectrum model for limited ] (]) | |||
| :: <math> | |||
| S(\omega) = 155 \frac{H_{1/3}^2}{T_1^4 \omega^5} \mathrm{exp} \left(\frac{-944}{T_1^4 \omega^4}\right)(3.3)^Y, | |||
| </math> | |||
| :where | |||
| :: <math>Y = \exp \left</math> | |||
| :: <math>\sigma = | |||
| \begin{cases} | |||
| 0.07 & \text{if }\omega \le 5.24 / T_1, \\ | |||
| 0.09 & \text{if }\omega > 5.24 / T_1. | |||
| \end{cases} | |||
| </math> | |||
| :(The latter model has since its creation improved based on the work of Phillips and Kitaigorodskii to better model the wave height spectrum for high ]s.<ref>{{cite journal|last1=Elfouhaily|first1=T.|last2=Chapron|first2=B.|last3=Katsaros|first3=K.|last4=Vandemark|first4=D.|title=A unified directional spectrum for long and short wind-driven waves|journal=]|date=July 15, 1997|volume=102|issue=C7|pages=15781–15796|url=http://archimer.ifremer.fr/doc/00091/20226/17877.pdf|doi=10.1029/97jc00467|bibcode = 1997JGR...10215781E |doi-access=free}}</ref>) | |||
| As for WDS, an example model of <math>f(\Theta)</math> might be: | |||
| Because these effects are related to a spatial variation in the phase speed, and because the phase speed also changes with the ambient current – due to the ] – the same effects of refraction and altering wave height also occur due to current variations. In the case of meeting an adverse current the wave ''steepens'', i.e. its wave height increases while the wave length decreases, similar to the shoaling when the water depth decreases.<ref>{{Citation | doi = 10.1016/0011-7471(64)90001-4 | volume = 11 | issue = 4 | pages = 529–562 | last1 = Longuet-Higgins | first1 = M.S. | author-link = Michael Longuet-Higgins | first2 = R.W. | last2 = Stewart | title = Radiation stresses in water waves; a physical discussion, with applications | journal = Deep Sea Research | year = 1964 }}</ref> | |||
| : <math>f(\Theta) = \frac{2}{\pi}\cos^2\Theta, \qquad -\pi/2 \le \Theta \le \pi/2</math> | |||
| Thus the sea state is fully determined and can be recreated by the following function where <math>\zeta</math> is the wave elevation, <math>\epsilon_{j}</math> is uniformly distributed between 0 and <math>2\pi</math>, and <math>\Theta_j</math> is randomly drawn from the directional distribution function <math>\sqrt{f(\Theta)}:</math><ref>{{citation | first=E. R. | last=Jefferys | title=Directional seas should be ergodic | journal=Applied Ocean Research | volume=9 | issue=4 | year=1987 | pages=186–191 | doi=10.1016/0141-1187(87)90001-0 | bibcode=1987AppOR...9..186J }}</ref> | |||
| : <math>\zeta = \sum_{j=1}^N \sqrt{2 S(\omega_j) \Delta \omega_j}\; \sin(\omega_j t - k_j x \cos \Theta_j - k_j y \sin \Theta_j + \epsilon_{j}).</math> | |||
| ==Shoaling and refraction== | |||
| ==Wave breaking== | |||
| ] in beaches.]] | |||
| ] | |||
| {{ |
{{main|Wave shoaling|Water wave refraction}} | ||
| As waves travel from deep to shallow water, their shape changes (wave height increases, speed decreases, and length decreases as wave orbits become asymmetrical). This process is called ]. | |||
| Wave ] is the process that occurs when waves interact with the sea bed to slow the velocity of propagation as a function of wavelength and period. As the waves slow down in shoaling water, the ]s tend to realign at a decreasing angle to the depth contours. Varying depths along a wave crest cause the crest to travel at different ]s, with those parts of the wave in deeper water moving faster than those in ]. This process continues while the depth decreases, and reverses if it increases again, but the wave leaving the shoal area may have changed direction considerably. ]—lines ] to wave crests between which a fixed amount of energy ] is contained—converge on local shallows and shoals. Therefore, the ] between rays is concentrated as they converge, with a resulting increase in wave height. | |||
| Some waves undergo a ] called "breaking". A ] is one whose base can no longer support its top, causing it to collapse. A wave breaks when it runs into ], or when two wave systems oppose and combine forces. When the slope, or steepness ratio, of a wave is too great, breaking is inevitable. | |||
| Because these effects are related to a spatial variation in the phase speed, and because the phase speed also changes with the ambient current—due to the ]—the same effects of refraction and altering wave height also occur due to current variations. In the case of meeting an adverse current the wave ''steepens'', i.e. its wave height increases while the wavelength decreases, similar to the shoaling when the water depth decreases.<ref>{{cite journal | doi = 10.1016/0011-7471(64)90001-4 | volume = 11 | issue = 4 | pages = 529–562 | last1 = Longuet-Higgins | first1 = M. S. | author-link = Michael Longuet-Higgins | first2 = R. W. | last2 = Stewart | title = Radiation stresses in water waves; a physical discussion, with applications | journal = Deep-Sea Research | year = 1964 |bibcode = 1964DSRA...11..529L }}</ref> | |||
| Individual waves in deep water break when the wave steepness—the ] of the ] ''H'' to the ] ''λ''—exceeds about 0.07, so for ''H'' > 0.07 ''λ''. In shallow water, with the water depth small compared to the wavelength, the individual waves break when their wave height ''H'' is larger than 0.8 times the water depth ''h'', that is ''H'' > 0.8 ''h''.<ref>{{Cite book | author= R.J. Dean and R.A. Dalrymple | title=Coastal processes with engineering applications | year=2002 | publisher=Cambridge University Press | isbn=0-521-60275-0 }} p. 96–97.</ref> Waves can also break if the wind grows strong enough to blow the crest off the base of the wave. | |||
| ==Breaking== | |||
| Three main types of breaking waves are identified by ]s or ]. Their varying characteristics make them more or less suitable for surfing, and present different dangers. | |||
| ] | |||
| ] | |||
| {{see also|Surf wave|Breaking wave|Iribarren number}} | |||
| Some waves undergo a ] called "breaking".<ref>{{Cite book|url=https://books.google.com/books?id=E5F8KiuSVjAC&q=Some+waves+undergo+a+phenomenon+called+%22breaking%22.&pg=PA319|title=Advances in Robotics and Virtual Reality|last1=Gulrez|first1=Tauseef|last2=Hassanien|first2=Aboul Ella|date=2011-11-13|publisher=Springer Science & Business Media|isbn=9783642233630|language=en}}</ref> A ] is one whose base can no longer support its top, causing it to collapse. A wave breaks when it runs into ], or when two wave systems oppose and combine forces. When the slope, or steepness ratio, of a wave, is too great, breaking is inevitable. | |||
| *'''Spilling''', or '''rolling''': these are the safest waves on which to surf. They can be found in most areas with relatively flat shorelines. They are the most common type of shorebreak | |||
| *'''Plunging''', or '''dumping''': these break suddenly and can "dump" swimmers—pushing them to the bottom with great force. These are the preferred waves for experienced surfers. Strong offshore winds and long wave periods can cause dumpers. They are often found where there is a sudden rise in the sea floor, such as a reef or sandbar. | |||
| *'''Surging''': these may never actually break as they approach the water's edge, as the water below them is very deep. They tend to form on steep shorelines. These waves can knock swimmers over and drag them back into deeper water. | |||
| Individual waves in deep water break when the wave steepness—the ] of the ] ''H'' to the ] ''λ''—exceeds about 0.17, so for ''H'' > 0.17 ''λ''. In shallow water, with the water depth small compared to the wavelength, the individual waves break when their wave height ''H'' is larger than 0.8 times the water depth ''h'', that is ''H'' > 0.8 ''h''.<ref>{{Cite book |author= R.J. Dean and R.A. Dalrymple |title=Coastal processes with engineering applications |year=2002 |publisher=Cambridge University Press |isbn=978-0-521-60275-4 }} p. 96–97.</ref> Waves can also break if the wind grows strong enough to blow the crest off the base of the wave. | |||
| ==Science of waves== | |||
| ])]] | |||
| In shallow water, the base of the wave is decelerated by drag on the seabed. As a result, the upper parts will propagate at a higher velocity than the base and the leading face of the crest will become steeper and the trailing face flatter. This may be exaggerated to the extent that the leading face forms a barrel profile, with the crest falling forward and down as it extends over the air ahead of the wave. | |||
| Wind waves are mechanical ]s that propagate along the interface between ] and ]; the restoring force is provided by gravity, and so they are often referred to as ]. As the ] blows, pressure and friction forces perturb the equilibrium of the water surface. These forces transfer energy from the air to the water, forming waves. The initial formation of waves by the wind is described in the theory of Phillips from 1957, and the subsequent growth of the small waves has been modeled by ], also in 1957.<ref>{{citation | first=O. M. | last=Phillips | year=1957 | title=On the generation of waves by turbulent wind | journal=Journal of Fluid Mechanics | volume=2 | issue=5 | pages=417–445 | doi=10.1017/S0022112057000233 |bibcode = 1957JFM.....2..417P }}</ref><ref>{{citation | first=J. W. | last=Miles | authorlink=John W. Miles | year=1957 | title=On the generation of surface waves by shear flows | journal=Journal of Fluid Mechanics | volume=3 | issue=2 | pages=185–204 | doi=10.1017/S0022112057000567 |bibcode = 1957JFM.....3..185M }}</ref> | |||
| Three main types of breaking waves are identified by ]s or ]. Their varying characteristics make them more or less suitable for surfing and present different dangers. | |||
| ])]] | |||
| # Spilling, or rolling: these are the safest waves on which to surf. They can be found in most areas with relatively flat shorelines. They are the most common type of shorebreak. The deceleration of the wave base is gradual, and the velocity of the upper parts does not differ much with height. Breaking occurs mainly when the steepness ratio exceeds the stability limit. | |||
| ] in a ]. The wave conditions are: mean water depth ''d'' = {{convert|2.50|ft|m|abbr=on}}, ] ''H'' = {{convert|0.339|ft|m|abbr=on}}, wavelength λ = {{convert|6.42|ft|m|abbr=on}}, ] ''T'' = 1.12 s.<ref>Figure 6 from: {{citation |first1=R.L. |last1=Wiegel |first2=J.W. |last2=Johnson |year=1950 |contribution=Elements of wave theory |title=Proceedings 1st International Conference on Coastal Engineering |location=Long Beach, California |publisher=] |pages=5–21 |url=http://journals.tdl.org/ICCE/article/view/905 }}</ref>]] | |||
| # Plunging, or dumping: these break suddenly and can "dump" swimmers—pushing them to the bottom with great force. These are the preferred waves for experienced surfers. Strong offshore winds and long wave periods can cause dumpers. They are often found where there is a sudden rise in the seafloor, such as a reef or sandbar. Deceleration of the wave base is sufficient to cause upward acceleration and a significant forward velocity excess of the upper part of the crest. The peak rises and overtakes the forward face, forming a "barrel" or "tube" as it collapses. | |||
| # Surging: these may never actually break as they approach the water's edge, as the water below them is very deep. They tend to form on steep shorelines. These waves can knock swimmers over and drag them back into deeper water. | |||
| When the shoreline is near vertical, waves do not break but are reflected. Most of the energy is retained in the wave as it returns to seaward. Interference patterns are caused by superposition of the incident and reflected waves, and the superposition may cause localized instability when peaks cross, and these peaks may break due to instability. (see also ]) | |||
| ==Physics of waves== | |||
| {{see also|Airy wave theory}} | {{see also|Airy wave theory}} | ||
| ])]] | |||
| Wind waves are mechanical ]s that propagate along the interface between ] and ]; the restoring force is provided by gravity, and so they are often referred to as ]. As the ] blows, pressure and friction perturb the equilibrium of the water surface and transfer energy from the air to the water, forming waves. The initial formation of waves by the wind is described in the theory of Phillips from 1957, and the subsequent growth of the small waves has been modeled by ], also in 1957.<ref>{{cite journal | first=O. M. | last=Phillips | year=1957 | title=On the generation of waves by turbulent wind | journal=Journal of Fluid Mechanics | volume=2 | issue=5 | pages=417–445 | doi=10.1017/S0022112057000233 | doi-broken-date=1 November 2024 |bibcode = 1957JFM.....2..417P | s2cid=116675962 }}</ref><ref>{{cite journal | first=J. W. | last=Miles | author-link=John W. Miles | year=1957 | title=On the generation of surface waves by shear flows | journal=Journal of Fluid Mechanics | volume=3 | issue=2 | pages=185–204 | doi=10.1017/S0022112057000567 | doi-broken-date=1 November 2024 |bibcode = 1957JFM.....3..185M | s2cid=119795395 }}</ref> | |||
| In the case of monochromatic linear plane waves in deep water, particles near the surface move in circular paths, making wind waves a combination of ] (back and forth) and ] (up and down) wave motions. When waves propagate in ], (where the depth is less than half the wavelength) the particle trajectories are compressed into ]s.<ref>For the particle trajectories within the framework of linear wave theory, see for instance: | |||
| <br>], page 44.<br>{{cite book | first=H. | last=Lamb | authorlink=Horace Lamb | year=1994 | title=Hydrodynamics | publisher=Cambridge University Press | edition=6th edition| isbn=978-0-521-45868-9 }} Originally published in 1879, the 6th extended edition appeared first in 1932. See §229, page 367.<br>{{cite book | title=Fluid mechanics | author=L. D. Landau and E. M. Lifshitz | year=1986 | publisher=Pergamon Press | series=Course of Theoretical Physics | volume=6 | edition=Second revised edition | isbn=0-08-033932-8 }} See page 33.</ref><ref>A good illustration of the wave motion according to linear theory is given by .</ref> | |||
| ])]] | |||
| As the wave amplitude (height) increases, the particle paths no longer form closed orbits; rather, after the passage of each crest, particles are displaced slightly from their previous positions, a phenomenon known as ].<ref>For nonlinear waves, the particle paths are not closed, as found by ] in 1847, see ]. Or in ], page 44: ''"To this order, it is evident that the particle paths are not exactly closed … pointed out by Stokes (1847) in his classical investigation"''.</ref><ref>Solutions of the particle trajectories in fully nonlinear periodic waves and the Lagrangian wave period they experience can for instance be found in:<br>{{cite journal| author=J.M. Williams| title=Limiting gravity waves in water of finite depth | journal=] | volume=302 | issue=1466 | pages=139–188 | year=1981| doi=10.1098/rsta.1981.0159 |bibcode = 1981RSPTA.302..139W }}<br>{{cite book| title=Tables of progressive gravity waves | author=J.M. Williams | year=1985 | publisher=Pitman | isbn=978-0-273-08733-5 }}</ref> | |||
| ] in a ]. The wave conditions are: mean water depth ''d'' = {{convert|2.50|ft|m|abbr=on}}, ] ''H'' = {{convert|0.339|ft|m|abbr=on}}, wavelength λ = {{convert|6.42|ft|m|abbr=on}}, ] ''T'' = 1.12 s.<ref>Figure 6 from: {{cite journal |first1=R. L. |last1=Wiegel |first2=J. W. |last2=Johnson |year=1950 | title=Proceedings 1st International Conference on Coastal Engineering |journal=Coastal Engineering Proceedings |issue=1 |location=Long Beach, California |publisher=] |pages=5–21 |doi=10.9753/icce.v1.2 |url=http://journals.tdl.org/ICCE/article/view/905|doi-access=free }}</ref>]] | |||
| In linear plane waves of one wavelength in deep water, ] near the surface move not plainly up and down but in circular orbits: forward above and backward below (compared to the wave propagation direction). As a result, the surface of the water forms not an exact ], but more a ] with the sharper curves upwards—as modeled in ] theory. Wind waves are thus a combination of ] and ] waves. | |||
| As the depth below the free surface increases, the radius of the circular motion decreases. At a depth equal to half the ] λ, the orbital movement has decayed to less than 5% of its value at the surface. The ] (also called the celerity) of a surface gravity wave is – for pure ] wave motion of small-] waves – well approximated by | |||
| When waves propagate in ], (where the depth is less than half the wavelength) the particle trajectories are compressed into ]s.<ref>For the particle trajectories within the framework of linear wave theory, see for instance: | |||
| <br>], page 44.<br>{{cite book | first=H. | last=Lamb | author-link=Horace Lamb | year=1994 | title=Hydrodynamics | publisher=Cambridge University Press | edition=6th| isbn=978-0-521-45868-9 }} Originally published in 1879, the 6th extended edition appeared first in 1932. See §229, page 367.<br>{{cite book | title=Fluid mechanics | author=L. D. Landau and E. M. Lifshitz | year=1986 | publisher=Pergamon Press | series=Course of Theoretical Physics | volume=6 | edition=Second revised | isbn=978-0-08-033932-0 }} See page 33.</ref><ref>A good illustration of the wave motion according to linear theory is given by {{Webarchive|url=https://web.archive.org/web/20171114103146/http://www.coastal.udel.edu/faculty/rad/linearplot.html |date=2017-11-14 }}.</ref> | |||
| In reality, for ] values of the wave amplitude (height), the particle paths do not form closed orbits; rather, after the passage of each crest, particles are displaced slightly from their previous positions, a phenomenon known as ].<ref>For nonlinear waves, the particle paths are not closed, as found by ] in 1847, see ]. Or in ], page 44: ''"To this order, it is evident that the particle paths are not exactly closed ... pointed out by Stokes (1847) in his classical investigation"''.</ref><ref>Solutions of the particle trajectories in fully nonlinear periodic waves and the Lagrangian wave period they experience can for instance be found in:<br>{{cite journal| author=J. M. Williams| title=Limiting gravity waves in water of finite depth | journal=] | volume=302 | issue=1466 | pages=139–188 | year=1981| doi=10.1098/rsta.1981.0159 |bibcode = 1981RSPTA.302..139W | s2cid=122673867 }}<br>{{cite book| title=Tables of progressive gravity waves | author=J. M. Williams | year=1985 | publisher=Pitman | isbn=978-0-273-08733-5 }}</ref> | |||
| As the depth below the free surface increases, the radius of the circular motion decreases. At a depth equal to half the ] λ, the orbital movement has decayed to less than 5% of its value at the surface. The ] (also called the celerity) of a surface gravity wave is—for pure ] wave motion of small-] waves—well approximated by | |||
| :<math>c=\sqrt{\frac{g \lambda}{2\pi} \tanh \left(\frac{2\pi d}{\lambda}\right)}</math> | :<math>c=\sqrt{\frac{g \lambda}{2\pi} \tanh \left(\frac{2\pi d}{\lambda}\right)}</math> | ||
| Line 218: | Line 244: | ||
| where | where | ||
| :''S'' = ] of the air-water interface; | :''S'' = ] of the air-water interface; | ||
| :<math>\rho</math> = ] of the water.<ref name=physics_handbook>{{cite book|title=Physics Handbook for Science and Engineering|year=2006|publisher=Studentliteratur|page=263|author=Carl Nordling, Jonny Östermalm|edition=Eight |
:<math>\rho</math> = ] of the water.<ref name=physics_handbook>{{cite book|title=Physics Handbook for Science and Engineering|year=2006|publisher=Studentliteratur|page=263|author=Carl Nordling, Jonny Östermalm|edition=Eight|isbn=978-91-44-04453-8}}</ref> | ||
| When several wave trains are present, as is always the case in nature, the waves form groups. In deep water the groups travel at a ] which is half of the ].<ref>In deep water, the ] is half the ], as is shown ]. Another reference is .</ref> Following a single wave in a group one can see the wave appearing at the back of the group, growing and finally disappearing at the front of the group. | When several wave trains are present, as is always the case in nature, the waves form groups. In deep water, the groups travel at a ] which is half of the ].<ref>In deep water, the ] is half the ], as is shown ]. Another reference is {{Webarchive|url=https://web.archive.org/web/20000312125351/http://musr.physics.ubc.ca/~jess/hr/skept/Waves/node12.html|date=2000-03-12}}.</ref> Following a single wave in a group one can see the wave appearing at the back of the group, growing, and finally disappearing at the front of the group. | ||
| As the water depth <math>d</math> decreases towards the ], this will have an effect: wave height changes due to ] and ]. As the wave height increases, the wave may become unstable when the ] of the wave moves faster than the ]. This causes ''surf'', a breaking of the waves. | As the water depth <math>d</math> decreases towards the ], this will have an effect: wave height changes due to ] and ]. As the wave height increases, the wave may become unstable when the ] of the wave moves faster than the ]. This causes ''surf'', a breaking of the waves. | ||
| Line 230: | Line 256: | ||
| The velocity of propagation of this energy is the ]. | The velocity of propagation of this energy is the ]. | ||
| ==Models== | |||
| ==Wind wave models== | |||
| [[File:Global Wave Height Speed.jpg|thumb|upright=1.25|The image shows the global distribution of wind speed and wave height as observed by NASA's TOPEX/Poseidon's dual-frequency radar altimeter from October 3 to October 12, 1992. Simultaneous observations of wind speed and wave height are helping scientists to predict ocean waves. Wind speed is determined by the strength of the radar signal after it has bounced off the ocean surface and returned to the satellite. A calm sea serves as a good reflector and returns a strong signal; a rough sea tends to scatter the signals and returns a weak pulse. Wave height is determined by the shape of the return radar pulse. | |||
| A calm sea with low waves returns a condensed pulse whereas a rough sea with high waves returns a stretched pulse. Comparing the two images above shows a high degree of correlation between wind speed and wave height. The strongest winds ({{convert|33.6|mph|km/h|abbr=on|disp=semicolon}}) and highest waves are found in the Southern Ocean. The weakest winds — shown as areas of magenta and dark blue — are generally found in the tropical oceans.]] | |||
| {{main|Wind wave model}} | {{main|Wind wave model}} | ||
| Surfers are very interested in the ]. There are many websites that provide predictions of the surf quality for the upcoming days and weeks. Wind wave models are driven by more general ] that predict the winds and pressures over the oceans, seas and lakes. | Surfers are very interested in the ]. There are many websites that provide predictions of the surf quality for the upcoming days and weeks. Wind wave models are driven by more general ] that predict the winds and pressures over the oceans, seas, and lakes. | ||
| Wind wave models are also an important part of examining the impact of ] and ] proposals. For many beach areas there is only patchy information about the wave climate, therefore estimating the effect of wind waves is important for managing ] environments. | Wind wave models are also an important part of examining the impact of ] and ] proposals. For many beach areas there is only patchy information about the wave climate, therefore estimating the effect of wind waves is important for managing ] environments. | ||
| A wind-generated wave can be predicted based on two parameters: wind speed at 10 m above sea level and wind duration, which must blow over long periods of time to be considered fully developed. The significant wave height and peak frequency can then be predicted for a certain fetch length.<ref>Wood, AMM & Fleming, CA 1981, Coastal hydraulics, John Wiley & Sons, New York</ref> | |||
| ==Seismic signals== | ==Seismic signals== | ||
| {{main| |
{{main|Microseism}} | ||
| Ocean water waves generate seismic waves that are globally visible on ].<ref>{{Cite web |url=http://www.earth.northwestern.edu/people/seth/327/HV/Chapter_4_rev1.pdf |title=Peter Bormann. Seismic Signals and Noise |access-date=2012-03-08 |archive-date=2016-03-04 |archive-url=https://web.archive.org/web/20160304002410/http://www.earth.northwestern.edu/people/seth/327/HV/Chapter_4_rev1.pdf |url-status=dead }}</ref> There are two principal constituents of the ocean wave-generated seismic microseism.<ref></ref> The strongest of these is the secondary microseism which is created by ocean floor pressures generated by interfering ocean waves and has a spectrum that is generally between approximately 6–12 s periods, or at approximately half of the period of the responsible interfering waves. The theory for microseism generation by standing waves was provided by ] in 1950 after in 1941 Pierre Bernard suggested this relation with standing waves on the basis of observations.<ref>{{cite journal |first=P. | last=Bernard |author-link=P. Bernard |title=Sur certaines proprietes de la boule etudiees a l'aide des enregistrements seismographiques |journal=Bulletin de l'Institut Océanographique de Monaco |volume=800 |pages=1–19 |year=1941 }}</ref><ref>{{cite journal |first=M. S. | last=Longuet-Higgins |author-link=Michael S. Longuet-Higgins |title=A theory of the origin of microseisms |journal=] |volume=243 |pages=1–35 |year=1950 |doi=10.1098/rsta.1950.0012 |issue=857 |bibcode = 1950RSPTA.243....1L | s2cid=31828394 }}</ref> The weaker primary microseism, also globally visible, is generated by dynamic seafloor pressures of propagating waves above shallower (less than several hundred meters depth) regions of the global ocean. Microseisms were first reported in about 1900, and seismic records provide long-term proxy measurements of seasonal and climate-related large-scale wave intensity in Earth's oceans <ref>{{cite journal |last1=Reguero |first1=Borja |last2=Losada |first2=Inigo J. |last3=Mendez |first3=Fernand J. |title=A recent increase in global wave power as a consequence of oceanic warming |journal=Nature Communications |date=2019 |volume=10 |issue=1 |pages=205 |doi=10.1038/s41467-018-08066-0|doi-access=free |pmid=30643133 |pmc=6331560 |bibcode=2019NatCo..10..205R }}</ref> including those associated with ].<ref>{{cite journal |last1=Aster |first1=Richard C. |last2=McNamara |first2=Daniel E. |last3=Bromirski |first3=Peter D. |title=Multidecadal climate-induced variability in microseisms |journal=Seismological Research Letters |date=2008 |volume=79 |issue=2 |pages=94–202 |doi=10.1785/gssrl.79.2.194|bibcode=2008SeiRL..79..194A }}</ref><ref>{{cite journal |last1=Bromirski |first1=Peter |title=Climate-Induced Decadal Ocean Wave Height Variability From Microseisms: 1931–2021 |journal=Journal of Geophysical Research: Oceans |date=2023 |volume=128 |issue=8 |page=e2023JC019722 |doi=10.1029/2023JC019722|doi-access=free |bibcode=2023JGRC..12819722B }}</ref><ref>{{cite journal |last1=Aster |first1=Richard C. |last2=Ringler |first2=Adam T. |last3=Anthony |first3=Robert E. |last4=Lee |first4=Thomas A. |title=Increasing ocean wave energy observed in Earth's seismic wavefield since the late 20th century |journal=Nature Communications |date=2023 |volume=14 |issue=1 |page=6984 |doi=10.1038/s41467-023-42673-w|doi-access=free |pmid=37914695 |pmc=10620394 }}</ref> | |||
| Ocean water waves generate land seismic waves that propagate hundreds of kilometers into the land.<ref></ref> These seismic signals usually have the period of 6 ± 2 seconds. Such recordings were first reported and understood in about 1900. | |||
| There are two types of seismic "ocean waves". The primary waves are generated in shallow waters by direct water wave-land interaction and have the same period as the water waves (10 to 16 seconds). The more powerful secondary waves are generated by the superposition of ocean waves of equal period traveling in opposite directions, thus generating standing gravity waves – with an associated pressure oscillation at half the period, which is not diminishing with depth. The theory for microseism generation by standing waves was provided by ] in 1950, after in 1941 Pierre Bernard suggested this relation with standing waves on the basis of observations.<ref>{{Citation |first=P. | last=Bernard |author-link=P. Bernard |title=Sur certaines proprietes de la boule etudiees a l'aide des enregistrements seismographiques |journal=Bull. Inst. Oceanogr. Monaco |volume=800 |pages=1–19 |year=1941 }}</ref><ref>{{Citation |first=M.S. | last=Longuet-Higgins |author-link=Michael S. Longuet-Higgins |title=A theory of the origin of microseisms |journal=] |volume=243 |pages=1–35 |year=1950 |doi=10.1098/rsta.1950.0012 |issue=857 |bibcode = 1950RSPTA.243....1L }}</ref> | |||
| ==Internal waves== | |||
| Internal waves can form at the boundary between water layers of different densities. These sub-surface waves are called internal waves. As is the case with ocean waves at the air-ocean interface, internal waves possess troughs, crests, wavelength, and period. Internal waves move very slowly because the density difference between the joined media is very small. Internal waves occur in the ocean at the base of the ], especially at the bottom edge of a steep ]. The wave height of internal waves may be greater than 30 meters (100 feet), causing the pycnocline to undulate slowly through a considerable depth. Their wavelength often exceeds {{Convert|0.8|km|mile}} and their periods are typically 5 to 8 minutes. Internal waves are generated by wind energy, tidal energy, and ocean currents. Surface manifestations of internal waves have been photographed from space. | |||
| Internal waves may mix nutrients into surface water and trigger plankton blooms. They can also affect submarines and oil platforms. | |||
| ==Other types of ocean waves== | |||
| ===Tidal waves=== | |||
| The only true tidal waves are relatively harmless waves associated with the ]s. Tsunami and storm surges are sometimes referred to as "tidal waves" in popular writing. | |||
| ===Storm surge=== | |||
| The abrupt bulge of water driven ashore by a tropical cyclone is called a ]. | |||
| ===Tsunami=== | |||
| A long-wavelength, shallow-water progressive waves caused by the rapid displacement of ocean water are called ]. Tsunami caused by the sudden, vertical movement of Earth along faults are properly called seismic sea waves. Tsunami can also be caused by landslides, icebergs falling from glaciers, volcanic eruptions, asteroid impacts, and other direct displacements of the water surface. All seismic sea waves are tsunami, but not all tsunami are seismic sea waves. The speed of a tsunami is given by the formula for the speed of a shallow-water wave. | |||
| ==See also== | ==See also== | ||
| {{ |
{{portal|Oceans}} | ||
| {{ |
{{div col}} | ||
| * |
*{{annotated link|Airy wave theory}} | ||
| *{{annotated link|Breakwater (structure)}} | |||
| *] | |||
| *{{annotated link|Boussinesq approximation (water waves)}} | |||
| *] | |||
| *{{annotated link|Clapotis}} | |||
| *] | |||
| *{{annotated link|Cross sea}} | |||
| *] | |||
| *{{annotated link|Gravity wave}} | |||
| {{Col-2-of-3}} | |||
| *{{annotated link|Internal wave}} | |||
| *] | |||
| *{{annotated link|Luke's variational principle}} | |||
| *] | |||
| *{{annotated link|Mild-slope equation}} | |||
| *] | |||
| *{{annotated link|Rogue wave}} | |||
| *] | |||
| *{{annotated link|Shallow water equations}} | |||
| {{Col-3-of-3}} | |||
| *{{annotated link|Tsunami}} | |||
| *] | |||
| *{{annotated link|Wave power}} | |||
| *] | |||
| *{{annotated link|Wave radar}} | |||
| *] | |||
| *{{annotated link|Wave setup}} | |||
| {{col-end}} | |||
| *{{annotated link|Waves and shallow water}} | |||
| {{div col end}} | |||
| ==Notes== | |||
| {{reflist|2}} | |||
| ==References== | ==References== | ||
| {{Reflist|30em}} | |||
| ===Scientific=== | ===Scientific=== | ||
| * |
*{{cite book | author= G. G. Stokes | author-link=Sir George Stokes, 1st Baronet |year= 1880 | title= Mathematical and Physical Papers, Volume I | publisher= Cambridge University Press | pages= –229 | url=https://archive.org/details/mathphyspapers01stokrich |ref= 441–455 }} | ||
| * |
*{{cite book | first=O. M. | last=Phillips | author-link=Owen Martin Phillips |title=The dynamics of the upper ocean |publisher=Cambridge University Press | year=1977 | edition=2nd | isbn=978-0-521-29801-8 |ref=Phillips1977}} | ||
| *{{cite book | title=Waves in oceanic and coastal waters | first=Leo H. | last=Holthuijsen | publisher=Cambridge University Press | year=2007 | isbn=0-521-86028- |
*{{cite book | title=Waves in oceanic and coastal waters | first=Leo H. | last=Holthuijsen | publisher=Cambridge University Press | year=2007 | isbn=978-0-521-86028-4 }} | ||
| * {{cite book | publisher = Cambridge University Press | isbn = 978-0-521-46540-3 | last = Janssen | first = Peter | title = The interaction of ocean waves and wind | year = 2004 }} | * {{cite book | publisher = Cambridge University Press | isbn = 978-0-521-46540-3 | last = Janssen | first = Peter | title = The interaction of ocean waves and wind | year = 2004 }} | ||
| ===Other=== | ===Other=== | ||
| *{{cite book | title=The Annapolis Book of Seamanship | edition=2nd revised | author-link=John Rousmaniere | first=John | last=Rousmaniere | publisher=Simon & Schuster | year=1989 | isbn=0-671-67447- |
*{{cite book | title=The Annapolis Book of Seamanship | edition=2nd revised | author-link=John Rousmaniere | first=John | last=Rousmaniere | publisher=Simon & Schuster | year=1989 | isbn=978-0-671-67447-2 | url-access=registration | url=https://archive.org/details/annapolisbookofs00rous_1 }} | ||
| *{{cite |
*{{cite magazine | last=Carr | first=Michael | title=Understanding Waves | magazine=Sail | date=October 1998 | pages=38–45 }} | ||
| ==External links== | ==External links== | ||
| {{Wikiquote}} | |||
| {{Commons category|Ocean surface waves}} | {{Commons category|Ocean surface waves}} | ||
| {{Commons category|Water waves}} | {{Commons category|Water waves}} | ||
| {{Wikibooks|High School Earth Science/Ocean Movements#Waves}} | |||
| * | |||
| * | |||
| * | |||
| * | |||
| * | |||
| * | |||
| * | |||
| {{coastal geography}} | {{coastal geography}} | ||
| {{physical oceanography}} | {{physical oceanography}} | ||
| {{Surfing}} | {{Surfing}} | ||
| {{Underwater diving|scidiv}} | |||
| {{Authority control}} | |||
| ] | ] | ||
| ] | ] | ||
| ] | ] | ||
| ] | |||
| ] | |||
| ] | |||
Latest revision as of 16:35, 2 November 2024
Surface waves generated by wind on open water "Ocean wave" redirects here. For other uses, see Ocean Wave (disambiguation).
In fluid dynamics, a wind wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result of the wind blowing over the water's surface. The contact distance in the direction of the wind is known as the fetch. Waves in the oceans can travel thousands of kilometers before reaching land. Wind waves on Earth range in size from small ripples to waves over 30 m (100 ft) high, being limited by wind speed, duration, fetch, and water depth.
When directly generated and affected by local wind, a wind wave system is called a wind sea. Wind waves will travel in a great circle route after being generated – curving slightly left in the southern hemisphere and slightly right in the northern hemisphere. After moving out of the area of fetch and no longer being affected by the local wind, wind waves are called swells and can travel thousands of kilometers. A noteworthy example of this is waves generated south of Tasmania during heavy winds that will travel across the Pacific to southern California, producing desirable surfing conditions. Wind waves in the ocean are also called ocean surface waves and are mainly gravity waves, where gravity is the main equilibrium force.
Wind waves have a certain amount of randomness: subsequent waves differ in height, duration, and shape with limited predictability. They can be described as a stochastic process, in combination with the physics governing their generation, growth, propagation, and decay – as well as governing the interdependence between flow quantities such as the water surface movements, flow velocities, and water pressure. The key statistics of wind waves (both seas and swells) in evolving sea states can be predicted with wind wave models.
Although waves are usually considered in the water seas of Earth, the hydrocarbon seas of Titan may also have wind-driven waves. Waves in bodies of water may also be generated by other causes, both at the surface and underwater (such as watercraft, animals, waterfalls, landslides, earthquakes, bubbles, and impact events).
Formation





The great majority of large breakers seen at a beach result from distant winds. Five factors influence the formation of the flow structures in wind waves:
- Wind speed or strength relative to wave speed – the wind must be moving faster than the wave crest for energy transfer to the wave.
- The uninterrupted distance of open water over which the wind blows without significant change in direction (called the fetch)
- Width of the area affected by fetch (at a right angle to the distance)
- Wind duration – the time for which the wind has blown over the water.
- Water depth
All of these factors work together to determine the size of the water waves and the structure of the flow within them.
The main dimensions associated with wave propagation are:
- Wave height (vertical distance from trough to crest)
- Wave length (distance from crest to crest in the direction of propagation)
- Wave period (time interval between arrival of consecutive crests at a stationary point)
- Wave direction or azimuth (predominantly driven by wind direction)
A fully developed sea has the maximum wave size theoretically possible for a wind of specific strength, duration, and fetch. Further exposure to that specific wind could only cause a dissipation of energy due to the breaking of wave tops and formation of "whitecaps". Waves in a given area typically have a range of heights. For weather reporting and for scientific analysis of wind wave statistics, their characteristic height over a period of time is usually expressed as significant wave height. This figure represents an average height of the highest one-third of the waves in a given time period (usually chosen somewhere in the range from 20 minutes to twelve hours), or in a specific wave or storm system. The significant wave height is also the value a "trained observer" (e.g. from a ship's crew) would estimate from visual observation of a sea state. Given the variability of wave height, the largest individual waves are likely to be somewhat less than twice the reported significant wave height for a particular day or storm.
Wave formation on an initially flat water surface by wind is started by a random distribution of normal pressure of turbulent wind flow over the water. This pressure fluctuation produces normal and tangential stresses in the surface water, which generates waves. It is usually assumed for the purpose of theoretical analysis that:
- The water is originally at rest.
- The water is not viscous.
- The water is irrotational.
- There is a random distribution of normal pressure to the water surface from the turbulent wind.
- Correlations between air and water motions are neglected.
The second mechanism involves wind shear forces on the water surface. John W. Miles suggested a surface wave generation mechanism that is initiated by turbulent wind shear flows based on the inviscid Orr–Sommerfeld equation in 1957. He found the energy transfer from the wind to the water surface is proportional to the curvature of the velocity profile of the wind at the point where the mean wind speed is equal to the wave speed. Since the wind speed profile is logarithmic to the water surface, the curvature has a negative sign at this point. This relation shows the wind flow transferring its kinetic energy to the water surface at their interface.
Assumptions:
- two-dimensional parallel shear flow
- incompressible, inviscid water and wind
- irrotational water
- slope of the displacement of the water surface is small
Generally, these wave formation mechanisms occur together on the water surface and eventually produce fully developed waves.
For example, if we assume a flat sea surface (Beaufort state 0), and a sudden wind flow blows steadily across the sea surface, the physical wave generation process follows the sequence:
- Turbulent wind forms random pressure fluctuations at the sea surface. Ripples with wavelengths in the order of a few centimeters are generated by the pressure fluctuations. (The Phillips mechanism)
- The winds keep acting on the initially rippled sea surface causing the waves to become larger. As the waves grow, the pressure differences get larger causing the growth rate to increase. Finally, the shear instability expedites the wave growth exponentially. (The Miles mechanism)
- The interactions between the waves on the surface generate longer waves and the interaction will transfer wave energy from the shorter waves generated by the Miles mechanism to the waves which have slightly lower frequencies than the frequency at the peak wave magnitudes, then finally the waves will be faster than the crosswind speed (Pierson & Moskowitz).
| Conditions necessary for a fully developed sea at given wind speeds, and the parameters of the resulting waves | |||||
|---|---|---|---|---|---|
| Wind conditions | Wave size | ||||
| Wind speed in one direction | Fetch | Wind duration | Average height | Average wavelength | Average period and speed |
| 19 km/h (12 mph) | 19 km (12 mi) | 2 hr | 0.27 m (0.89 ft) | 8.5 m (28 ft) | 3.0 sec, 10.2 km/h (9.3 ft/sec) |
| 37 km/h (23 mph) | 139 km (86 mi) | 10 hr | 1.5 m (4.9 ft) | 33.8 m (111 ft) | 5.7 sec, 21.4 km/h (19.5 ft/sec) |
| 56 km/h (35 mph) | 518 km (322 mi) | 23 hr | 4.1 m (13 ft) | 76.5 m (251 ft) | 8.6 sec, 32.0 km/h (29.2 ft/sec) |
| 74 km/h (46 mph) | 1,313 km (816 mi) | 42 hr | 8.5 m (28 ft) | 136 m (446 ft) | 11.4 sec, 42.9 km/h (39.1 ft/sec) |
| 92 km/h (57 mph) | 2,627 km (1,632 mi) | 69 hr | 14.8 m (49 ft) | 212.2 m (696 ft) | 14.3 sec, 53.4 km/h (48.7 ft/sec) |
| NOTE: Most of the wave speeds calculated from the wave length divided by the period are proportional to the square root of the wave length. Thus, except for the shortest wave length, the waves follow the deep water theory. The 28 ft long wave must be either in shallow water or intermediate depth. | |||||
Types

Three different types of wind waves develop over time:
- Capillary waves, or ripples, dominated by surface tension effects.
- Gravity waves, dominated by gravitational and inertial forces.
- Seas, raised locally by the wind.
- Swells, which have traveled away from where they were raised by the wind, and have to a greater or lesser extent dispersed.
Ripples appear on smooth water when the wind blows, but will die quickly if the wind stops. The restoring force that allows them to propagate is surface tension. Sea waves are larger-scale, often irregular motions that form under sustained winds. These waves tend to last much longer, even after the wind has died, and the restoring force that allows them to propagate is gravity. As waves propagate away from their area of origin, they naturally separate into groups of common direction and wavelength. The sets of waves formed in this manner are known as swells. The Pacific Ocean is 19,800 km (12,300 mi) from Indonesia to the coast of Colombia and, based on an average wavelength of 76.5 m (251 ft), would have ~258,824 swells over that width.
It is sometimes alleged that out of a set of waves, the seventh wave in a set is always the largest; while this isn't the case, the waves in the middle of a given set tend to be larger than those before and after them.
Individual "rogue waves" (also called "freak waves", "monster waves", "killer waves", and "king waves") much higher than the other waves in the sea state can occur. In the case of the Draupner wave, its 25 m (82 ft) height was 2.2 times the significant wave height. Such waves are distinct from tides, caused by the Moon and Sun's gravitational pull, tsunamis that are caused by underwater earthquakes or landslides, and waves generated by underwater explosions or the fall of meteorites—all having far longer wavelengths than wind waves.
The largest ever recorded wind waves are not rogue waves, but standard waves in extreme sea states. For example, 29.1 m (95 ft) high waves were recorded on the RRS Discovery in a sea with 18.5 m (61 ft) significant wave height, so the highest wave was only 1.6 times the significant wave height. The biggest recorded by a buoy (as of 2011) was 32.3 m (106 ft) high during the 2007 typhoon Krosa near Taiwan.
Spectrum

Ocean waves can be classified based on: the disturbing force that creates them; the extent to which the disturbing force continues to influence them after formation; the extent to which the restoring force weakens or flattens them; and their wavelength or period. Seismic sea waves have a period of about 20 minutes, and speeds of 760 km/h (470 mph). Wind waves (deep-water waves) have a period up to about 20 seconds.
| Wave type | Typical wavelength | Disturbing force | Restoring force |
|---|---|---|---|
| Capillary wave | < 2 cm | Wind | Surface tension |
| Wind wave | 60–150 m (200–490 ft) | Wind over ocean | Gravity |
| Seiche | Large, variable; a function of basin size | Change in atmospheric pressure, storm surge | Gravity |
| Seismic sea wave (tsunami) | 200 km (120 mi) | Faulting of sea floor, volcanic eruption, landslide | Gravity |
| Tide | Half the circumference of Earth | Gravitational attraction, rotation of Earth | Gravity |
The speed of all ocean waves is controlled by gravity, wavelength, and water depth. Most characteristics of ocean waves depend on the relationship between their wavelength and water depth. Wavelength determines the size of the orbits of water molecules within a wave, but water depth determines the shape of the orbits. The paths of water molecules in a wind wave are circular only when the wave is traveling in deep water. A wave cannot "feel" the bottom when it moves through water deeper than half its wavelength because too little wave energy is contained in the water movement below that depth. Waves moving through water deeper than half their wavelength are known as deep-water waves. On the other hand, the orbits of water molecules in waves moving through shallow water are flattened by the proximity of the sea bottom surface. Waves in water shallower than 1/20 their original wavelength are known as shallow-water waves. Transitional waves travel through water deeper than 1/20 their original wavelength but shallower than half their original wavelength.
In general, the longer the wavelength, the faster the wave energy will move through the water. The relationship between the wavelength, period and velocity of any wave is:
where C is speed (celerity), L is the wavelength, and T is the period (in seconds). Thus the speed of the wave derives from the functional dependence of the wavelength on the period (the dispersion relation).
The speed of a deep-water wave may also be approximated by:
where g is the acceleration due to gravity, 9.8 meters (32 feet) per second squared. Because g and π (3.14) are constants, the equation can be reduced to:
when C is measured in meters per second and L in meters. In both formulas the wave speed is proportional to the square root of the wavelength.
The speed of shallow-water waves is described by a different equation that may be written as:
where C is speed (in meters per second), g is the acceleration due to gravity, and d is the depth of the water (in meters). The period of a wave remains unchanged regardless of the depth of water through which it is moving. As deep-water waves enter the shallows and feel the bottom, however, their speed is reduced, and their crests "bunch up", so their wavelength shortens.
Spectral models
Sea state can be described by the sea wave spectrum or just wave spectrum . It is composed of a wave height spectrum (WHS) and a wave direction spectrum (WDS) . Many interesting properties about the sea state can be found from the wave spectra.
WHS describes the spectral density of wave height variance ("power") versus wave frequency, with dimension . The relationship between the spectrum and the wave amplitude for a wave component is:
Some WHS models are listed below.
- The International Towing Tank Conference (ITTC) recommended spectrum model for fully developed sea (ISSC spectrum/modified Pierson-Moskowitz spectrum):
- ITTC recommended spectrum model for limited fetch (JONSWAP spectrum)
- where
- (The latter model has since its creation improved based on the work of Phillips and Kitaigorodskii to better model the wave height spectrum for high wavenumbers.)
As for WDS, an example model of might be:
Thus the sea state is fully determined and can be recreated by the following function where is the wave elevation, is uniformly distributed between 0 and , and is randomly drawn from the directional distribution function
Shoaling and refraction

As waves travel from deep to shallow water, their shape changes (wave height increases, speed decreases, and length decreases as wave orbits become asymmetrical). This process is called shoaling.
Wave refraction is the process that occurs when waves interact with the sea bed to slow the velocity of propagation as a function of wavelength and period. As the waves slow down in shoaling water, the crests tend to realign at a decreasing angle to the depth contours. Varying depths along a wave crest cause the crest to travel at different phase speeds, with those parts of the wave in deeper water moving faster than those in shallow water. This process continues while the depth decreases, and reverses if it increases again, but the wave leaving the shoal area may have changed direction considerably. Rays—lines normal to wave crests between which a fixed amount of energy flux is contained—converge on local shallows and shoals. Therefore, the wave energy between rays is concentrated as they converge, with a resulting increase in wave height.
Because these effects are related to a spatial variation in the phase speed, and because the phase speed also changes with the ambient current—due to the Doppler shift—the same effects of refraction and altering wave height also occur due to current variations. In the case of meeting an adverse current the wave steepens, i.e. its wave height increases while the wavelength decreases, similar to the shoaling when the water depth decreases.
Breaking


Some waves undergo a phenomenon called "breaking". A breaking wave is one whose base can no longer support its top, causing it to collapse. A wave breaks when it runs into shallow water, or when two wave systems oppose and combine forces. When the slope, or steepness ratio, of a wave, is too great, breaking is inevitable.
Individual waves in deep water break when the wave steepness—the ratio of the wave height H to the wavelength λ—exceeds about 0.17, so for H > 0.17 λ. In shallow water, with the water depth small compared to the wavelength, the individual waves break when their wave height H is larger than 0.8 times the water depth h, that is H > 0.8 h. Waves can also break if the wind grows strong enough to blow the crest off the base of the wave.
In shallow water, the base of the wave is decelerated by drag on the seabed. As a result, the upper parts will propagate at a higher velocity than the base and the leading face of the crest will become steeper and the trailing face flatter. This may be exaggerated to the extent that the leading face forms a barrel profile, with the crest falling forward and down as it extends over the air ahead of the wave.
Three main types of breaking waves are identified by surfers or surf lifesavers. Their varying characteristics make them more or less suitable for surfing and present different dangers.
- Spilling, or rolling: these are the safest waves on which to surf. They can be found in most areas with relatively flat shorelines. They are the most common type of shorebreak. The deceleration of the wave base is gradual, and the velocity of the upper parts does not differ much with height. Breaking occurs mainly when the steepness ratio exceeds the stability limit.
- Plunging, or dumping: these break suddenly and can "dump" swimmers—pushing them to the bottom with great force. These are the preferred waves for experienced surfers. Strong offshore winds and long wave periods can cause dumpers. They are often found where there is a sudden rise in the seafloor, such as a reef or sandbar. Deceleration of the wave base is sufficient to cause upward acceleration and a significant forward velocity excess of the upper part of the crest. The peak rises and overtakes the forward face, forming a "barrel" or "tube" as it collapses.
- Surging: these may never actually break as they approach the water's edge, as the water below them is very deep. They tend to form on steep shorelines. These waves can knock swimmers over and drag them back into deeper water.
When the shoreline is near vertical, waves do not break but are reflected. Most of the energy is retained in the wave as it returns to seaward. Interference patterns are caused by superposition of the incident and reflected waves, and the superposition may cause localized instability when peaks cross, and these peaks may break due to instability. (see also clapotic waves)
Physics of waves
See also: Airy wave theory
Wind waves are mechanical waves that propagate along the interface between water and air; the restoring force is provided by gravity, and so they are often referred to as surface gravity waves. As the wind blows, pressure and friction perturb the equilibrium of the water surface and transfer energy from the air to the water, forming waves. The initial formation of waves by the wind is described in the theory of Phillips from 1957, and the subsequent growth of the small waves has been modeled by Miles, also in 1957.

In linear plane waves of one wavelength in deep water, parcels near the surface move not plainly up and down but in circular orbits: forward above and backward below (compared to the wave propagation direction). As a result, the surface of the water forms not an exact sine wave, but more a trochoid with the sharper curves upwards—as modeled in trochoidal wave theory. Wind waves are thus a combination of transversal and longitudinal waves.
When waves propagate in shallow water, (where the depth is less than half the wavelength) the particle trajectories are compressed into ellipses.
In reality, for finite values of the wave amplitude (height), the particle paths do not form closed orbits; rather, after the passage of each crest, particles are displaced slightly from their previous positions, a phenomenon known as Stokes drift.
As the depth below the free surface increases, the radius of the circular motion decreases. At a depth equal to half the wavelength λ, the orbital movement has decayed to less than 5% of its value at the surface. The phase speed (also called the celerity) of a surface gravity wave is—for pure periodic wave motion of small-amplitude waves—well approximated by
where
- c = phase speed;
- λ = wavelength;
- d = water depth;
- g = acceleration due to gravity at the Earth's surface.
In deep water, where , so and the hyperbolic tangent approaches , the speed approximates
In SI units, with in m/s, , when is measured in metres. This expression tells us that waves of different wavelengths travel at different speeds. The fastest waves in a storm are the ones with the longest wavelength. As a result, after a storm, the first waves to arrive on the coast are the long-wavelength swells.
For intermediate and shallow water, the Boussinesq equations are applicable, combining frequency dispersion and nonlinear effects. And in very shallow water, the shallow water equations can be used.
If the wavelength is very long compared to the water depth, the phase speed (by taking the limit of c when the wavelength approaches infinity) can be approximated by
On the other hand, for very short wavelengths, surface tension plays an important role and the phase speed of these gravity-capillary waves can (in deep water) be approximated by
where
- S = surface tension of the air-water interface;
- = density of the water.
When several wave trains are present, as is always the case in nature, the waves form groups. In deep water, the groups travel at a group velocity which is half of the phase speed. Following a single wave in a group one can see the wave appearing at the back of the group, growing, and finally disappearing at the front of the group.
As the water depth decreases towards the coast, this will have an effect: wave height changes due to wave shoaling and refraction. As the wave height increases, the wave may become unstable when the crest of the wave moves faster than the trough. This causes surf, a breaking of the waves.
The movement of wind waves can be captured by wave energy devices. The energy density (per unit area) of regular sinusoidal waves depends on the water density , gravity acceleration and the wave height (which, for regular waves, is equal to twice the amplitude, ):
The velocity of propagation of this energy is the group velocity.
Models
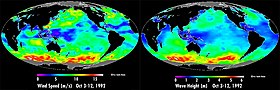
Surfers are very interested in the wave forecasts. There are many websites that provide predictions of the surf quality for the upcoming days and weeks. Wind wave models are driven by more general weather models that predict the winds and pressures over the oceans, seas, and lakes.
Wind wave models are also an important part of examining the impact of shore protection and beach nourishment proposals. For many beach areas there is only patchy information about the wave climate, therefore estimating the effect of wind waves is important for managing littoral environments.
A wind-generated wave can be predicted based on two parameters: wind speed at 10 m above sea level and wind duration, which must blow over long periods of time to be considered fully developed. The significant wave height and peak frequency can then be predicted for a certain fetch length.
Seismic signals
Main article: MicroseismOcean water waves generate seismic waves that are globally visible on seismographs. There are two principal constituents of the ocean wave-generated seismic microseism. The strongest of these is the secondary microseism which is created by ocean floor pressures generated by interfering ocean waves and has a spectrum that is generally between approximately 6–12 s periods, or at approximately half of the period of the responsible interfering waves. The theory for microseism generation by standing waves was provided by Michael Longuet-Higgins in 1950 after in 1941 Pierre Bernard suggested this relation with standing waves on the basis of observations. The weaker primary microseism, also globally visible, is generated by dynamic seafloor pressures of propagating waves above shallower (less than several hundred meters depth) regions of the global ocean. Microseisms were first reported in about 1900, and seismic records provide long-term proxy measurements of seasonal and climate-related large-scale wave intensity in Earth's oceans including those associated with anthropogenic global warming.
See also
- Airy wave theory – Fluid dynamics theory on the propagation of gravity waves
- Breakwater (structure) – Coastal defense structure
- Boussinesq approximation (water waves) – Approximation valid for weakly non-linear and fairly long waves
- Clapotis – Non-breaking standing wave pattern
- Cross sea – Sea state with two wave systems traveling at oblique angles
- Gravity wave – Wave where gravity is the main restoring force
- Internal wave – Type of wave within a fluid medium
- Luke's variational principle – Mathematics of surface waves on a fluid
- Mild-slope equation – Physics phenomenon and formula
- Rogue wave – Unexpectedly large transient ocean surface wave
- Shallow water equations – Set of partial differential equations that describe the flow below a pressure surface in a fluid
- Tsunami – Series of water waves caused by the displacement of a large volume of a body of water
- Wave power – Transport of energy by wind waves, and the capture of that energy to do useful work
- Wave radar – Technology for measuring surface waves on water
- Wave setup – The increase in mean water level due to the presence of breaking waves
- Waves and shallow water – Effect of shallow water on a surface gravity wave
References
- Tolman, H. L. (23 June 2010). Mahmood, M.F. (ed.). CBMS Conference Proceedings on Water Waves: Theory and Experiment (PDF). Howard University, US, 13–18 May 2008: World Scientific Publications. ISBN 978-981-4304-23-8.
{{cite book}}: CS1 maint: location (link) - Holthuijsen (2007), page 5.
- Lorenz, R. D.; Hayes, A. G. (2012). "The Growth of Wind-Waves in Titan's Hydrocarbon Seas". Icarus. 219 (1): 468–475. Bibcode:2012Icar..219..468L. doi:10.1016/j.icarus.2012.03.002.
- Barnes, Jason W.; Sotin, Christophe; Soderblom, Jason M.; Brown, Robert H.; Hayes, Alexander G.; Donelan, Mark; Rodriguez, Sebastien; Mouélic, Stéphane Le; Baines, Kevin H.; McCord, Thomas B. (2014-08-21). "Cassini/VIMS observes rough surfaces on Titan's Punga Mare in specular reflection". Planetary Science. 3 (1): 3. Bibcode:2014PlSci...3....3B. doi:10.1186/s13535-014-0003-4. ISSN 2191-2521. PMC 4959132. PMID 27512619.
- Heslar, Michael F.; Barnes, Jason W.; Soderblom, Jason M.; Seignovert, Benoît; Dhingra, Rajani D.; Sotin, Christophe (2020-08-14). "Tidal Currents Detected in Kraken Mare Straits from Cassini VIMS Sun Glitter Observations". The Planetary Science Journal. 1 (2): 35. arXiv:2007.00804. Bibcode:2020PSJ.....1...35H. doi:10.3847/PSJ/aba191. ISSN 2632-3338. S2CID 220301577.
- Young, I. R. (1999). Wind generated ocean waves. Elsevier. p. 83. ISBN 978-0-08-043317-2.
- Weisse, Ralf; von Storch, Hans (2008). Marine climate change: Ocean waves, storms and surges in the perspective of climate change. Springer. p. 51. ISBN 978-3-540-25316-7.
- ^ Phillips, O. M. (2006). "On the generation of waves by turbulent wind". Journal of Fluid Mechanics. 2 (5): 417. Bibcode:1957JFM.....2..417P. doi:10.1017/S0022112057000233 (inactive 1 November 2024). S2CID 116675962.
{{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) - Miles, John W. (2006). "On the generation of surface waves by shear flows". Journal of Fluid Mechanics. 3 (2): 185. Bibcode:1957JFM.....3..185M. doi:10.1017/S0022112057000567 (inactive 1 November 2024). S2CID 119795395.
{{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) - "Chapter 16, Ocean Waves". Archived from the original on 2016-05-11. Retrieved 2013-11-12.
- Hasselmann, K.; et al. (1973). "Measurements of wind-wave growth and swell decay during the Joint North Sea Wave Project (JONSWAP)". Ergnzungsheft zur Deutschen Hydrographischen Zeitschrift Reihe A. 8 (12): 95. hdl:10013/epic.20654.
- Pierson, Willard J.; Moskowitz, Lionel (15 December 1964). "A proposed spectral form for fully developed wind seas based on the similarity theory of S. A. Kitaigorodskii". Journal of Geophysical Research. 69 (24): 5181–5190. Bibcode:1964JGR....69.5181P. doi:10.1029/JZ069i024p05181.
- "Know the risks: Waves". Royal National Lifeboat Institution. Retrieved 31 August 2024.
- Holliday, Naomi P.; Yelland, Margaret J.; Pascal, Robin; Swail, Val R.; Taylor, Peter K.; Griffiths, Colin R.; Kent, Elizabeth (2006). "Were extreme waves in the Rockall Trough the largest ever recorded?". Geophysical Research Letters. 33 (L05613). Bibcode:2006GeoRL..33.5613H. doi:10.1029/2005GL025238.
- P. C. Liu; H. S. Chen; D.-J. Doong; C. C. Kao; Y.-J. G. Hsu (11 June 2008). "Monstrous ocean waves during typhoon Krosa". Annales Geophysicae. 26 (6): 1327–1329. Bibcode:2008AnGeo..26.1327L. doi:10.5194/angeo-26-1327-2008.
- Munk, Walter H. (1950). "Proceedings 1st International Conference on Coastal Engineering". Coastal Engineering Proceedings (1). Long Beach, California: ASCE: 1–4. doi:10.9753/icce.v1.1.
- Tom Garrison (2009). Oceanography: An Invitation to Marine Science (7th ed.). Yolanda Cossio. ISBN 978-0495391937.
- International Towing Tank Conference (ITTC), retrieved 11 November 2010
- International Ship and Offshore Structures Congress
- Pierson, W. J.; Moscowitz, L. (1964), "A proposed spectral form for fully developed wind seas based on the similarity theory of S A Kitaigorodskii", Journal of Geophysical Research, 69 (24): 5181–5190, Bibcode:1964JGR....69.5181P, doi:10.1029/JZ069i024p05181
- Elfouhaily, T.; Chapron, B.; Katsaros, K.; Vandemark, D. (July 15, 1997). "A unified directional spectrum for long and short wind-driven waves" (PDF). Journal of Geophysical Research. 102 (C7): 15781–15796. Bibcode:1997JGR...10215781E. doi:10.1029/97jc00467.
- Jefferys, E. R. (1987), "Directional seas should be ergodic", Applied Ocean Research, 9 (4): 186–191, Bibcode:1987AppOR...9..186J, doi:10.1016/0141-1187(87)90001-0
- Longuet-Higgins, M. S.; Stewart, R. W. (1964). "Radiation stresses in water waves; a physical discussion, with applications". Deep-Sea Research. 11 (4): 529–562. Bibcode:1964DSRA...11..529L. doi:10.1016/0011-7471(64)90001-4.
- Gulrez, Tauseef; Hassanien, Aboul Ella (2011-11-13). Advances in Robotics and Virtual Reality. Springer Science & Business Media. ISBN 9783642233630.
- R.J. Dean and R.A. Dalrymple (2002). Coastal processes with engineering applications. Cambridge University Press. ISBN 978-0-521-60275-4. p. 96–97.
- Phillips, O. M. (1957). "On the generation of waves by turbulent wind". Journal of Fluid Mechanics. 2 (5): 417–445. Bibcode:1957JFM.....2..417P. doi:10.1017/S0022112057000233 (inactive 1 November 2024). S2CID 116675962.
{{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) - Miles, J. W. (1957). "On the generation of surface waves by shear flows". Journal of Fluid Mechanics. 3 (2): 185–204. Bibcode:1957JFM.....3..185M. doi:10.1017/S0022112057000567 (inactive 1 November 2024). S2CID 119795395.
{{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) - Figure 6 from: Wiegel, R. L.; Johnson, J. W. (1950). "Proceedings 1st International Conference on Coastal Engineering". Coastal Engineering Proceedings (1). Long Beach, California: ASCE: 5–21. doi:10.9753/icce.v1.2.
- For the particle trajectories within the framework of linear wave theory, see for instance:
Phillips (1977), page 44.
Lamb, H. (1994). Hydrodynamics (6th ed.). Cambridge University Press. ISBN 978-0-521-45868-9. Originally published in 1879, the 6th extended edition appeared first in 1932. See §229, page 367.
L. D. Landau and E. M. Lifshitz (1986). Fluid mechanics. Course of Theoretical Physics. Vol. 6 (Second revised ed.). Pergamon Press. ISBN 978-0-08-033932-0. See page 33. - A good illustration of the wave motion according to linear theory is given by Prof. Robert Dalrymple's Java applet Archived 2017-11-14 at the Wayback Machine.
- For nonlinear waves, the particle paths are not closed, as found by George Gabriel Stokes in 1847, see the original paper by Stokes. Or in Phillips (1977), page 44: "To this order, it is evident that the particle paths are not exactly closed ... pointed out by Stokes (1847) in his classical investigation".
- Solutions of the particle trajectories in fully nonlinear periodic waves and the Lagrangian wave period they experience can for instance be found in:
J. M. Williams (1981). "Limiting gravity waves in water of finite depth". Philosophical Transactions of the Royal Society A. 302 (1466): 139–188. Bibcode:1981RSPTA.302..139W. doi:10.1098/rsta.1981.0159. S2CID 122673867.
J. M. Williams (1985). Tables of progressive gravity waves. Pitman. ISBN 978-0-273-08733-5. - Carl Nordling, Jonny Östermalm (2006). Physics Handbook for Science and Engineering (Eight ed.). Studentliteratur. p. 263. ISBN 978-91-44-04453-8.
- In deep water, the group velocity is half the phase velocity, as is shown here. Another reference is Archived 2000-03-12 at the Wayback Machine.
- Wood, AMM & Fleming, CA 1981, Coastal hydraulics, John Wiley & Sons, New York
- "Peter Bormann. Seismic Signals and Noise" (PDF). Archived from the original (PDF) on 2016-03-04. Retrieved 2012-03-08.
- Ardhuin, Fabrice, Lucia Gualtieri, and Eleonore Stutzmann. "How ocean waves rock the Earth: two mechanisms explain seismic noise with periods 3 to 300 s." Geophys. Res. Lett. 42 (2015).
- Bernard, P. (1941). "Sur certaines proprietes de la boule etudiees a l'aide des enregistrements seismographiques". Bulletin de l'Institut Océanographique de Monaco. 800: 1–19.
- Longuet-Higgins, M. S. (1950). "A theory of the origin of microseisms". Philosophical Transactions of the Royal Society A. 243 (857): 1–35. Bibcode:1950RSPTA.243....1L. doi:10.1098/rsta.1950.0012. S2CID 31828394.
- Reguero, Borja; Losada, Inigo J.; Mendez, Fernand J. (2019). "A recent increase in global wave power as a consequence of oceanic warming". Nature Communications. 10 (1): 205. Bibcode:2019NatCo..10..205R. doi:10.1038/s41467-018-08066-0. PMC 6331560. PMID 30643133.
- Aster, Richard C.; McNamara, Daniel E.; Bromirski, Peter D. (2008). "Multidecadal climate-induced variability in microseisms". Seismological Research Letters. 79 (2): 94–202. Bibcode:2008SeiRL..79..194A. doi:10.1785/gssrl.79.2.194.
- Bromirski, Peter (2023). "Climate-Induced Decadal Ocean Wave Height Variability From Microseisms: 1931–2021". Journal of Geophysical Research: Oceans. 128 (8): e2023JC019722. Bibcode:2023JGRC..12819722B. doi:10.1029/2023JC019722.
- Aster, Richard C.; Ringler, Adam T.; Anthony, Robert E.; Lee, Thomas A. (2023). "Increasing ocean wave energy observed in Earth's seismic wavefield since the late 20th century". Nature Communications. 14 (1): 6984. doi:10.1038/s41467-023-42673-w. PMC 10620394. PMID 37914695.
Scientific
- G. G. Stokes (1880). Mathematical and Physical Papers, Volume I. Cambridge University Press. pp. 197–229.
- Phillips, O. M. (1977). The dynamics of the upper ocean (2nd ed.). Cambridge University Press. ISBN 978-0-521-29801-8.
- Holthuijsen, Leo H. (2007). Waves in oceanic and coastal waters. Cambridge University Press. ISBN 978-0-521-86028-4.
- Janssen, Peter (2004). The interaction of ocean waves and wind. Cambridge University Press. ISBN 978-0-521-46540-3.
Other
- Rousmaniere, John (1989). The Annapolis Book of Seamanship (2nd revised ed.). Simon & Schuster. ISBN 978-0-671-67447-2.
- Carr, Michael (October 1998). "Understanding Waves". Sail. pp. 38–45.
External links
| Underwater diving | |||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||

 of the wavelength on the period (the
of the wavelength on the period (the 


 . It is composed of a wave height spectrum (WHS)
. It is composed of a wave height spectrum (WHS)  and a wave direction spectrum (WDS)
and a wave direction spectrum (WDS)  . Many interesting properties about the sea state can be found from the wave spectra.
. Many interesting properties about the sea state can be found from the wave spectra.
 .
The relationship between the spectrum
.
The relationship between the spectrum  and the wave amplitude
and the wave amplitude  for a wave component
for a wave component  is:
is:






 is the wave elevation,
is the wave elevation,  is uniformly distributed between 0 and
is uniformly distributed between 0 and  , and
, and  is randomly drawn from the directional distribution function
is randomly drawn from the directional distribution function 


 , so
, so  and the hyperbolic tangent approaches
and the hyperbolic tangent approaches  , the speed
, the speed  approximates
approximates

 in m/s,
in m/s,  , when
, when  is measured in metres.
This expression tells us that waves of different wavelengths travel at different speeds. The fastest waves in a storm are the ones with the longest wavelength. As a result, after a storm, the first waves to arrive on the coast are the long-wavelength swells.
is measured in metres.
This expression tells us that waves of different wavelengths travel at different speeds. The fastest waves in a storm are the ones with the longest wavelength. As a result, after a storm, the first waves to arrive on the coast are the long-wavelength swells.


 =
=  decreases towards the
decreases towards the  and the wave height
and the wave height  (which, for regular waves, is equal to twice the
(which, for regular waves, is equal to twice the  ):
):




