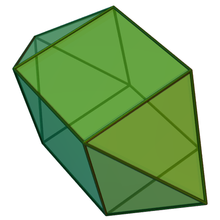
| Elongated square bipyramid | |
|---|---|
 | |
| Type | Johnson J14 – J15 – J16 |
| Faces | 8 triangles 4 squares |
| Edges | 20 |
| Vertices | 10 |
| Vertex configuration | |
| Symmetry group | |
| Dual polyhedron | square bifrustum |
| Properties | convex |
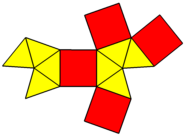
| Net | |
 | |
In geometry, the elongated square bipyramid (or elongated octahedron) is the polyhedron constructed by attaching two equilateral square pyramids onto a cube's faces that are opposite each other. It can also be seen as 4 lunes (squares with triangles on opposite sides) linked together with squares to squares and triangles to triangles. It is also been named the pencil cube or 12-faced pencil cube due to its shape.
A zircon crystal is an example of an elongated square bipyramid.
Construction
The elongated square bipyramid is constructed by attaching two equilateral square pyramids onto the faces of a cube that are opposite each other, a process known as elongation. This construction involves the removal of those two squares and replacing them with those pyramids, resulting in eight equilateral triangles and four squares as their faces.. A convex polyhedron in which all of its faces are regular is a Johnson solid, and the elongated square bipyramid is one of them, denoted as , the fifteenth Johnson solid.
Properties
Given that is the edge length of an elongated square bipyramid. The height of an elongated square pyramid can be calculated by adding the height of two equilateral square pyramids and a cube. The height of a cube is the same as the given edge length , and the height of an equilateral square pyramid is . Therefore, the height of an elongated square bipyramid is: Its surface area can be calculated by adding all the area of eight equilateral triangles and four squares: Its volume is obtained by slicing it into two equilateral square pyramids and a cube, and then adding them: Its dihedral angle can be obtained in a similar way as the elongated square pyramid, by adding the angle of square pyramids and a cube:
- The dihedral angle of an elongated square bipyramid between two adjacent triangles is the dihedral angle of an equilateral triangle between its lateral faces,
- The dihedral angle of an elongated square bipyramid between two adjacent squares is the dihedral angle of a cube between those,
- The dihedral angle of an equilateral square pyramid between square and triangle is . Therefore, the dihedral angle of an elongated square bipyramid between triangle-to-square, on the edge where the equilateral square pyramids attach the cube, is

The elongated square bipyramid has the dihedral symmetry, the dihedral group of order eight: it has an axis of symmetry passing through the apices of square pyramids and the center of a cube, and its appearance is symmetrical by reflecting across a horizontal plane.
Related polyhedra and honeycombs
The elongated square bipyramid is dual to the square bifrustum, which has eight trapezoidals and two squares.
A special kind of elongated square bipyramid without all regular faces allows a self-tessellation of Euclidean space. The triangles of this elongated square bipyramid are not regular; they have edges in the ratio 2:√3:√3.
 The honeycomb
The honeycomb The half-honeycomb
The half-honeycomb
It can be considered a transitional phase between the cubic and rhombic dodecahedral honeycombs. Here, the cells are colored white, red, and blue based on their orientation in space. The square pyramid caps have shortened isosceles triangle faces, with six of these pyramids meeting together to form a cube. The dual of this honeycomb is composed of two kinds of octahedra (regular octahedra and triangular antiprisms), formed by superimposing octahedra into the cuboctahedra of the rectified cubic honeycomb. Both honeycombs have a symmetry of .

Cross-sections of the honeycomb, through cell centers, produce a chamfered square tiling, with flattened horizontal and vertical hexagons, and squares on the perpendicular polyhedra.
With regular faces, the elongated square bipyramid can form a tessellation of space with tetrahedra and octahedra. (The octahedra can be further decomposed into square pyramids.) This honeycomb can be considered an elongated version of the tetrahedral-octahedral honeycomb.
References
- ^ Critchlow, Keith. Order in Space: A design source book. p. 46–47.
- Goldberg, Michael (January 1981). "On the space-filling octahedra". Geometriae Dedicata. 10 (1): 323–335. doi:10.1007/BF01447431.
- Rajwade, A. R. (2001). Convex Polyhedra with Regularity Conditions and Hilbert's Third Problem. Texts and Readings in Mathematics. Hindustan Book Agency. p. 84–89. doi:10.1007/978-93-86279-06-4. ISBN 978-93-86279-06-4.
- Uehara, Ryuhei (2020). Introduction to Computational Origami: The World of New Computational Geometry. Springer. p. 62. doi:10.1007/978-981-15-4470-5. ISBN 978-981-15-4470-5. S2CID 220150682.
- Sapiña, R. "Area and volume of the Johnson solid ". Problemas y Ecuaciones (in Spanish). ISSN 2659-9899. Retrieved 2020-09-09.
- ^ Berman, Martin (1971). "Regular-faced convex polyhedra". Journal of the Franklin Institute. 291 (5): 329–352. doi:10.1016/0016-0032(71)90071-8. MR 0290245.
- ^ Johnson, Norman W. (1966). "Convex polyhedra with regular faces". Canadian Journal of Mathematics. 18: 169–200. doi:10.4153/cjm-1966-021-8. MR 0185507. S2CID 122006114. Zbl 0132.14603.
- "J15 honeycomb".


 , the fifteenth Johnson solid.
, the fifteenth Johnson solid.
 is the edge length of an elongated square bipyramid. The height of an elongated square pyramid can be calculated by adding the height of two equilateral square pyramids and a cube. The height of a cube is the same as the given edge length
is the edge length of an elongated square bipyramid. The height of an elongated square pyramid can be calculated by adding the height of two equilateral square pyramids and a cube. The height of a cube is the same as the given edge length  . Therefore, the height of an elongated square bipyramid is:
. Therefore, the height of an elongated square bipyramid is:
 Its surface area can be calculated by adding all the area of eight equilateral triangles and four squares:
Its surface area can be calculated by adding all the area of eight equilateral triangles and four squares:
 Its volume is obtained by slicing it into two equilateral square pyramids and a cube, and then adding them:
Its volume is obtained by slicing it into two equilateral square pyramids and a cube, and then adding them:
 Its
Its 

 . Therefore, the dihedral angle of an elongated square bipyramid between triangle-to-square, on the edge where the equilateral square pyramids attach the cube, is
. Therefore, the dihedral angle of an elongated square bipyramid between triangle-to-square, on the edge where the equilateral square pyramids attach the cube, is 

 .
.